Find the velocity of the centre of mass of two identical particles moving with velocities v1 and v2. Solution in Bengali
If the mass of each particle is m, the resultant momentum = (m→v1+m→v2)=m(→v1+→v2). If the velocity of the centre of mass of the two particles is →r, then the momentum of the centre of mass = (m+m) →r=2m→v.
∴ 2m→v=m(→v1+→v2)
Hence →v=→v1+→v22
Topper's Solved these Questions
- BOOK - CHHAYA PUBLICATIONCHAPTER - STATICSEXERCISE - EXAMINATION ARCHIVE with solutions (WBJEE)3videos
STATICS
- BOOK - CHHAYA PUBLICATIONCHAPTER - STATICSEXERCISE - EXAMINATION ARCHIVE with solutions (JEE Main )1videos
STATICS
- BOOK - CHHAYA PUBLICATIONCHAPTER - STATICSEXERCISE - Integer answer type1videos
STATICS
- BOOK - CHHAYA PUBLICATIONCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - CBSE SCANNER16videos
SIMPLE HARMONIC MOTION
- BOOK - CHHAYA PUBLICATIONCHAPTER - SUPERPOSITION OF WAVESEXERCISE - CBSE Scanner14videos
SUPERPOSITION OF WAVES
Similar Questions
A large number of particles are placed around the origin each at a distance R from the origin. The distance of the centre of mass of the system from the origin is
07:01View SolutionDistance of the centre of mass of a solid uniform cone from its vertex is z0. If the radius of its base is R and its height is h, then z0 is equal to
03:08View SolutionTwo ice skaters A and B approach each other at right angles. Skater A has a mass 30 kg and velocity 1 m.s−1, skater B has a mass 20 kg and velocity 2m.s−1. They meet and cling together. Find the final velocity (in m.s−1) of the couple.
04:44View SolutionExplain the relation in phase between displacement velocity and acceleration in SHM, graphically as well as theoretically.
04:19View SolutionA progressive wave is given by y(x,l)=Bcos(300t−0.15x) where x and y are in cm and t in second . What is the (i) direction of propagation, (ii) wavelength , (iii) frequency, (iv) wave speed and (v) phase difference between two points 0 . 2 m apart ?
05:00View SolutionIn the figure block moves downwards with velocity v1, the wedge rightwards with velocity v2. The correct relation between v1 and v2 is
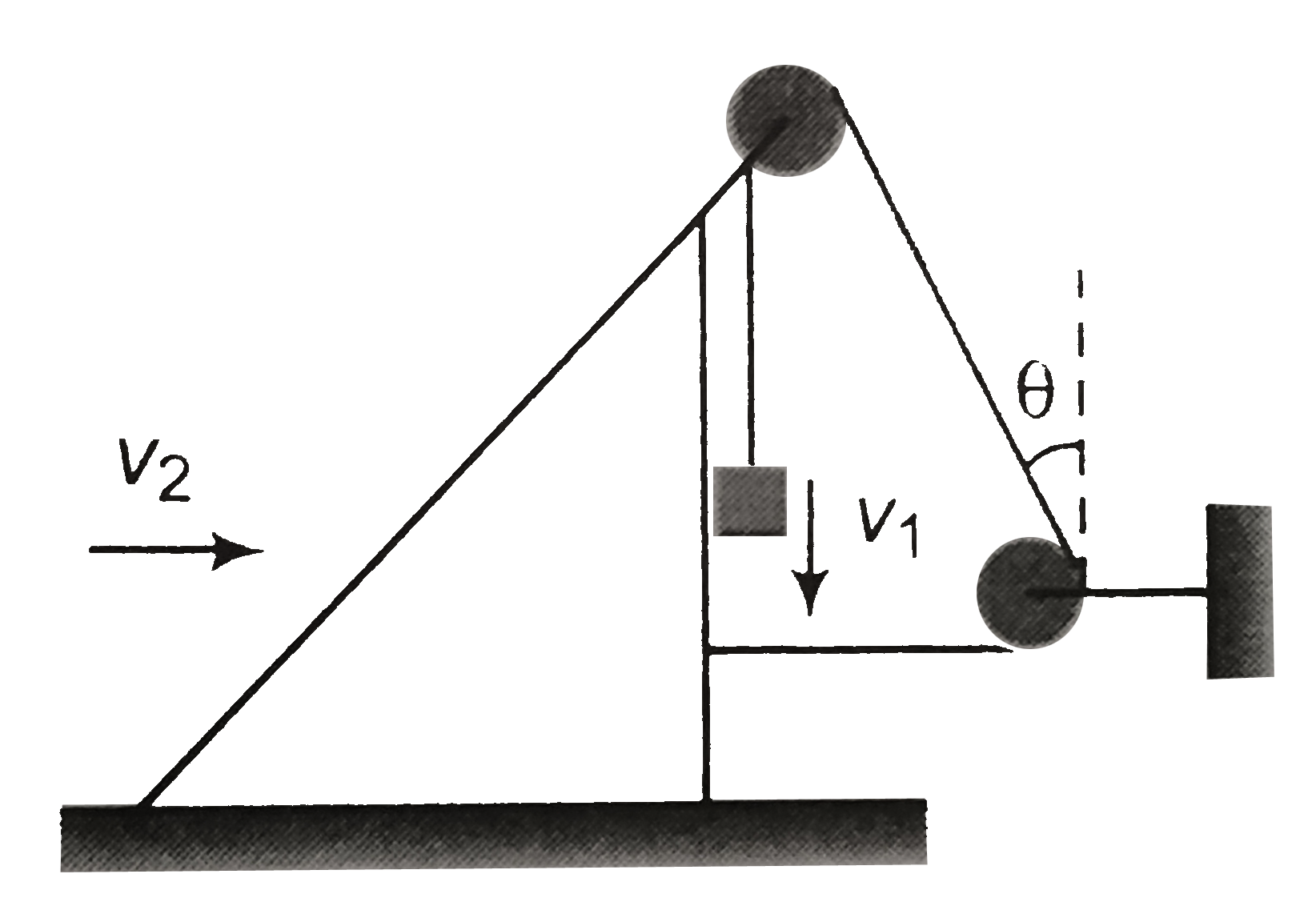 View Solution
View SolutionIn fig., blocks A and B move with velocities v_(1) and v_(2) along horizontal direction. Find the ratio of v_(1)//v_(2)
09:20View SolutionLet there are three equal masses situated at the vertices of an equilateral triangle, as shown in Fig. Now particle A starts with a velocity v1 towards line AB, particle B starts with the velocity v2, towards line BC and particle C starts with velocity v3 towards line CA. Find the displacement of the centre of mass of the three particles A,B and C after time t. What would it be if v1=v2=v3?
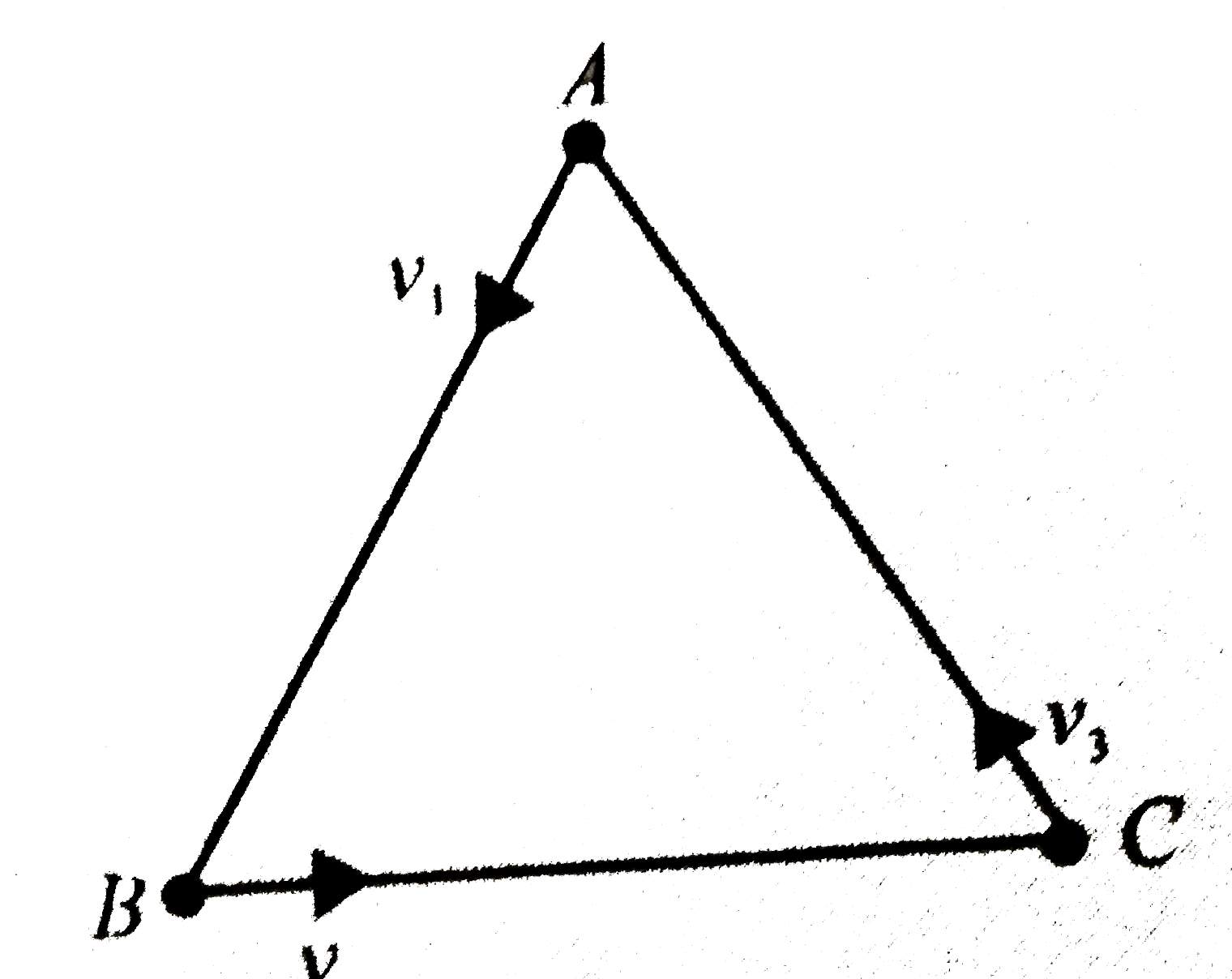 02:10View Solution
02:10View SolutionTwo masses, m1 and m2 , are moving with velocities v1 and v2. Find their total kinetic energy in the reference frame of centre of mass.
04:20View SolutionThe velocities of two particles of masses m1 and m2 relative to an observer in an inertial frame are v1 and v2. Determine the velocity of the center of mass relative to the observer and the velocity of each particle relative to the center of mass.
02:34View SolutionA particle of mass m is moving with a uniform velocity v_(1) . It is given an impulse such that its velocity becomes v_(2) . The impulse is equal to
10:09View Solutionकिसी कण के सापेक्ष वेग क्या अभिप्राय है ? दो कण A तथा B एक सीधी सड़क पर एक ही दिशा में क्रमश :vA एवं vB वेग में गतिशील हैं। दिखाइए कि कण A का कण B के सापेक्ष वेग vAB=vA−vB.
05:47View SolutionA particle of mass m is moving with a uniform velocity v_(1) . It is given an impulse such that its velocity becomes v_(2) . The impulse is equal to
01:11View SolutionTwo particles are moving with velocities v_(1) and v_2 . Their relative velocity is the maximum, when the angle between their velocities is
01:34View SolutionTwo identical particles move towards each other with velocity 2 v and v respectively. The velocity of centre of mass is
02:37View Solution

