Five particles each of mass 'm' are kept at five verties of a regular pentagon . A sixth particles of mass 'M' is kept at center of the pentagon 'O'.Distance between'M' and is 'm' is 'a'. Find
(a) net force on 'M'
(b) magnitude of net force on 'M' if any one particle is removed from one of the verties.
The correct Answer is:A, B
(a)FA=FB=FC=FD=FE=GMma2=F(say)
Angle between two successive force vectors is θ=360∘5=72∘.
When these five force vectors are added as per polygon law of vector addition we get another closed regular polygon as shown below.


Therefore, net resultant force on 'M' is zero. (b) When particle at A is removed, then FA will be removed. So, magnitude of net force will be F as shown below: 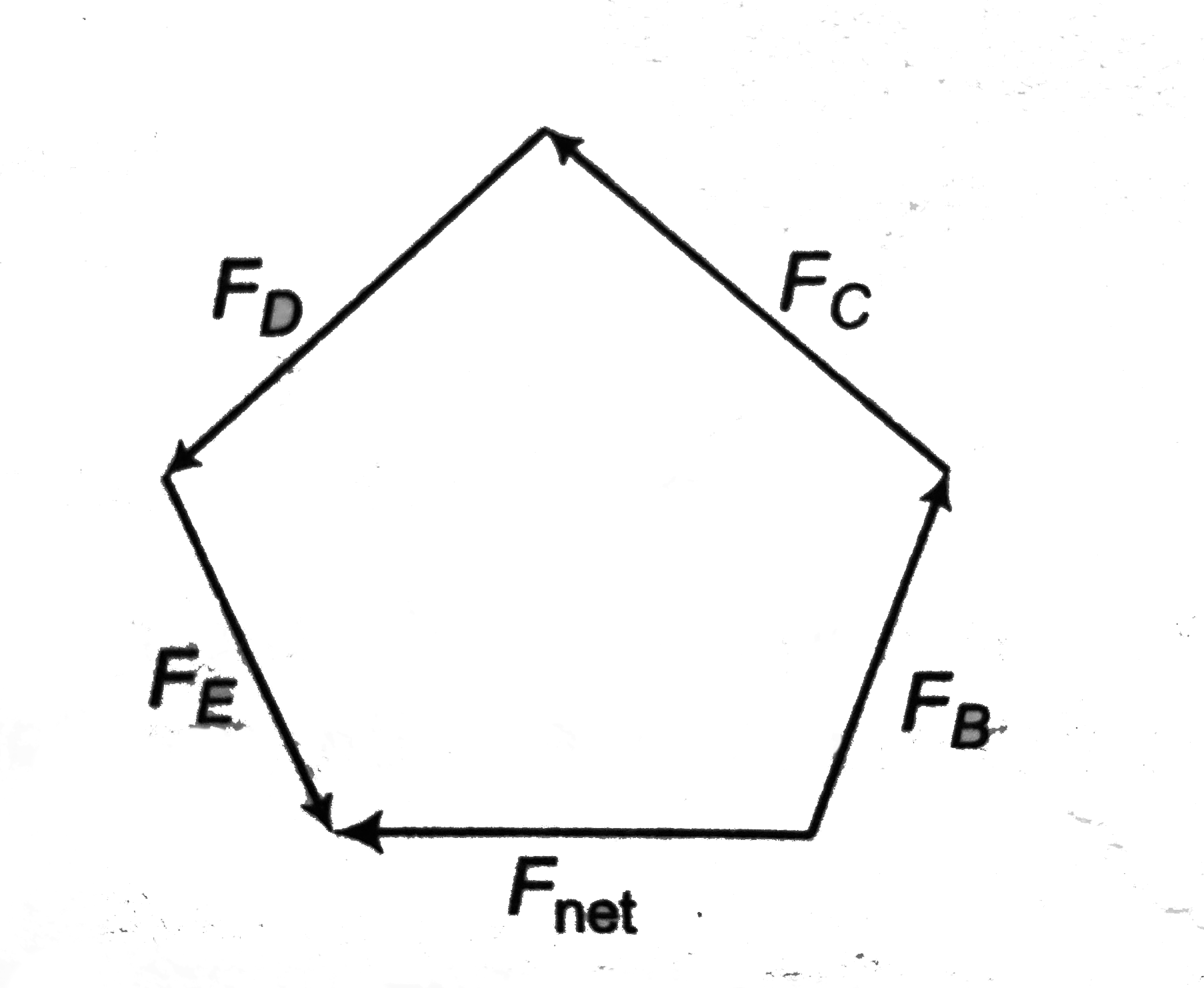
∴Fnet=F=GMma2
Topper's Solved these Questions
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - Solved Examples
16 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - Miscellaneous Examples
8 Videos
- GENERAL PHYSICSBOOK - DC PANDEYCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
- KINEMATICSBOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
11 Videos
Similar Questions
Six particles each of mass 'm' are placed at six verties A,B,C,D,Eand F of a regular hexagon of side 'a'. A seventh particle of mass 'M' is kept at center 'O' of the hexagon.
(a)Find net force on 'M'.
(b)Find net force on 'M' if particles at A is removed.
(c) Find net force on 'M' if particles at A and C are removed .
View SolutionFour particles each of mass m are kept at the four vertices of a square of side 'a' . Find graitational potential energy of this system.
View Solution
Knowledge Check
Three particles each of mass m are kept at vertices of an equilateral triangle of side L. The gravitational field at centre due to these particle is
AzeroB3GML2C9GML2D12GM√3L2Three particles each of mass m are kept at the vertices of an euilateral triangle of side L. The gravitational field at the centre due to these particle is
AzeroB3GML2C9GML2D2GML2Six particles each of massm are placed at the corners of a regular hexagon of edge length a. If a point mass m0 is placed at the centre of the hexagon, then the net gravitational force on the point mass is
A6Gm2a2B6Gmm0a2CzeroD6Gma4
Four particles each of mass 'm' are placed at the four vertices of a square 'a' .Find net force on any one the particle.
View SolutionFive point masses m each are kept at five vertices of a regular pentagon. Distance of centre of pentagon from any one of the verticle is 'a'. Find gravitational potential and filed strength at centre.
View SolutionEight particles of mass 'm' each are placed at the verties of a cube of side 'a' . Find gravitational potential energy of this system .
 .
.
View SolutionA heavy nucleous of masss M kept at rest in laboratory rejects a light particle of mass m .
View SolutionThree particles each of mass m are placed at the three corners of an equilateral triangle of side L. A particle of mass M is kept at the midpoint of any one side. What is the force acting on M, due to this system of 3 particles ?
View Solution
