For a circular coil of radius R and N turns carrying current I, the magnitude of the magnetic field at a point on its axis at a distance x from its centre is given by $B = \frac{μ_{0} I R^{2} N}{2 {(x^{2} + R^{2})}^{3 / 2}}$
(a) Show that this reduces to the familiar result for field at the centre of the coil.
(b) Consider two parallel coaxial circular coils of equal radius R, and number of turns N, carrying equal currents in the same direction, and separated by a distance R. Show that the field on the axis around the mid-point between the coils is uniform over a distance that is small as compared to R and is given by $B = 0 \cdot 72 \frac{μ_{0} N I}{R}$ approximately.
[Such as arrangement to produce a nearly uniform magnetic field over a small region is known as Helmholtz coils.]

Video Solution

Text Solution

Verified by Experts

(a) If we substitute $x = 0$ in this relation then we can get magnetic field at the centre of the coil.
$B = \frac{μ_{0} I R^{2} N}{2 R^{3}} = \frac{μ_{0} N I}{2 R}$
Hence, it has reduced to the relation for magnetic field at the centre of the coil.
(b)

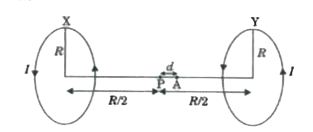
Let the midpoint of the line joining the centres of two coils be at P. Let A be the point around P. Let $P A = d$ such that $d < < R$ . Let distance of the point A is $R / 2 + d$ from X then it must be $R / 2 - d$ from the coil Y.
For coil X :
$x = R / 2 + d$
Magnetic field at A due to coil X
$B_{X} = \frac{μ_{0}}{4 π} \frac{2 π I R^{2} N}{{(R^{2} + x^{2})}^{3 / 2}}$
$= \frac{μ_{0}}{4 π} \times \frac{2 π I N R^{2}}{{[R^{2} + {(\frac{R}{2} + d)}^{2}]}^{3 / 2}}$
$= \frac{μ_{0}}{4 π} \times \frac{2 π I N R^{2}}{{[R^{2} + \frac{R^{2}}{4} + d^{2} + R d]}^{3 / 2}}$
Since $d < < R, d^{2}$ can be removed from the above exprcsion.
$\Rightarrow B_{X} = \frac{μ_{0} I N R^{2}}{2 {(\frac{5}{4} R^{2} + R d)}^{\frac{3}{2}}}$
$= \frac{μ_{0} I N R^{2}}{2 {(\frac{5}{4} R^{2})}^{\frac{3}{2}} {[1 + \frac{4 d}{5 R}]}^{\frac{3}{2}}}$
$= \frac{μ_{0} I N R^{2}}{2 {(\frac{5}{4} R^{2})}^{\frac{3}{2}}} {[1 + \frac{4 d}{5 R}]}^{- \frac{3}{2}}$
The direction of magnetic field $B_{X}$ is along PA towards the centre of coil Y.
For coil Y:
$x = R / 2 - d$
Magnetic field at A due to to coil Y:
$B_{Y} = \frac{μ_{0}}{4 π} \frac{2 π I R^{2} N}{{(R^{2} + x^{2})}^{3 / 2}}$
$= \frac{μ_{0}}{4 π} \times \frac{2 π I N R^{2}}{{[R^{2} + {(\frac{R}{2} - d)}^{2}]}^{3 / 2}}$
$= \frac{μ_{0}}{4 π} \times \frac{2 π I N R^{2}}{{[R^{2} + \frac{R^{2}}{4} + d^{2} - R d]}^{3 / 2}}$
Since $d < < R, d^{2}$ can be removed from the above expression.
$\Rightarrow B_{Y} = \frac{μ_{0} I N R^{2}}{2 {(\frac{5}{4} R^{2} - R d)}^{\frac{3}{2}}}$
$= \frac{μ_{0} I N R^{2}}{2 {(\frac{5}{4} R^{2})}^{\frac{3}{2}} {[1 - \frac{4 d}{5 R}]}^{\frac{3}{2}}}$
$= \frac{μ_{0} I N R^{2}}{2 {(\frac{5}{4} R^{2})}^{\frac{3}{2}}} {[1 - \frac{4 d}{5 R}]}^{- \frac{3}{2}}$
The direction of magnetic field $B_{Y}$ is along PA, towards the centre of coil Y. So, the resultant magnetic field at point A due to coils X and Y will be:
$B = B_{X} + B_{Y}$
$= \frac{μ_{0} I N R^{2}}{2 {(\frac{5}{4} R^{2})}^{\frac{3}{2}}} {[1 + \frac{4 d}{5 R}]}^{- \frac{3}{2}} + \frac{μ_{0} I N R^{2}}{2 {(\frac{5}{4} R^{2})}^{\frac{3}{2}}} {[1 - \frac{4 d}{5 R}]}^{- \frac{3}{2}}$
$= \frac{μ_{0} I N R^{2}}{2 {(\frac{5}{4} R^{2})}^{\frac{3}{2}}} ⎡ ⎣ {(1 + \frac{4 d}{5 R})}^{- \frac{3}{2}} + {(1 - \frac{4 d}{5 R})}^{- \frac{3}{2}} ⎤ ⎦$
Expanding the above expression by binomial theorem and neglecting higher powers as $d < < R$ , we get ,
$= \frac{μ_{0} I N R^{2}}{2 {(\frac{5}{4} R^{2})}^{\frac{3}{2}}} [(1 - \frac{3}{2} \times \frac{4 d}{5 R}) + (1 + \frac{3}{2} \times \frac{4 d}{5 R})]$
$= \frac{μ_{0} I N}{2 R} {(\frac{4}{5})}^{\frac{3}{2}} \times 2$
$= 0.72 \frac{μ_{0} N I}{R}$
$∴$ Total magnetic field due to two magnetic fields
$= 0.72 \frac{μ_{0} N I}{R}$ T at A due to coils X and Y . So, we can say that around P, the value of magnetic field remains almost constant because d is not appearing in the result.

Updated on:21/07/2023