For a wave described by y=Asin(ωt−kx), consider the following or not and in what direction and describe whether the particle is speeding up, slowing sown or instantanteously not accelerating?
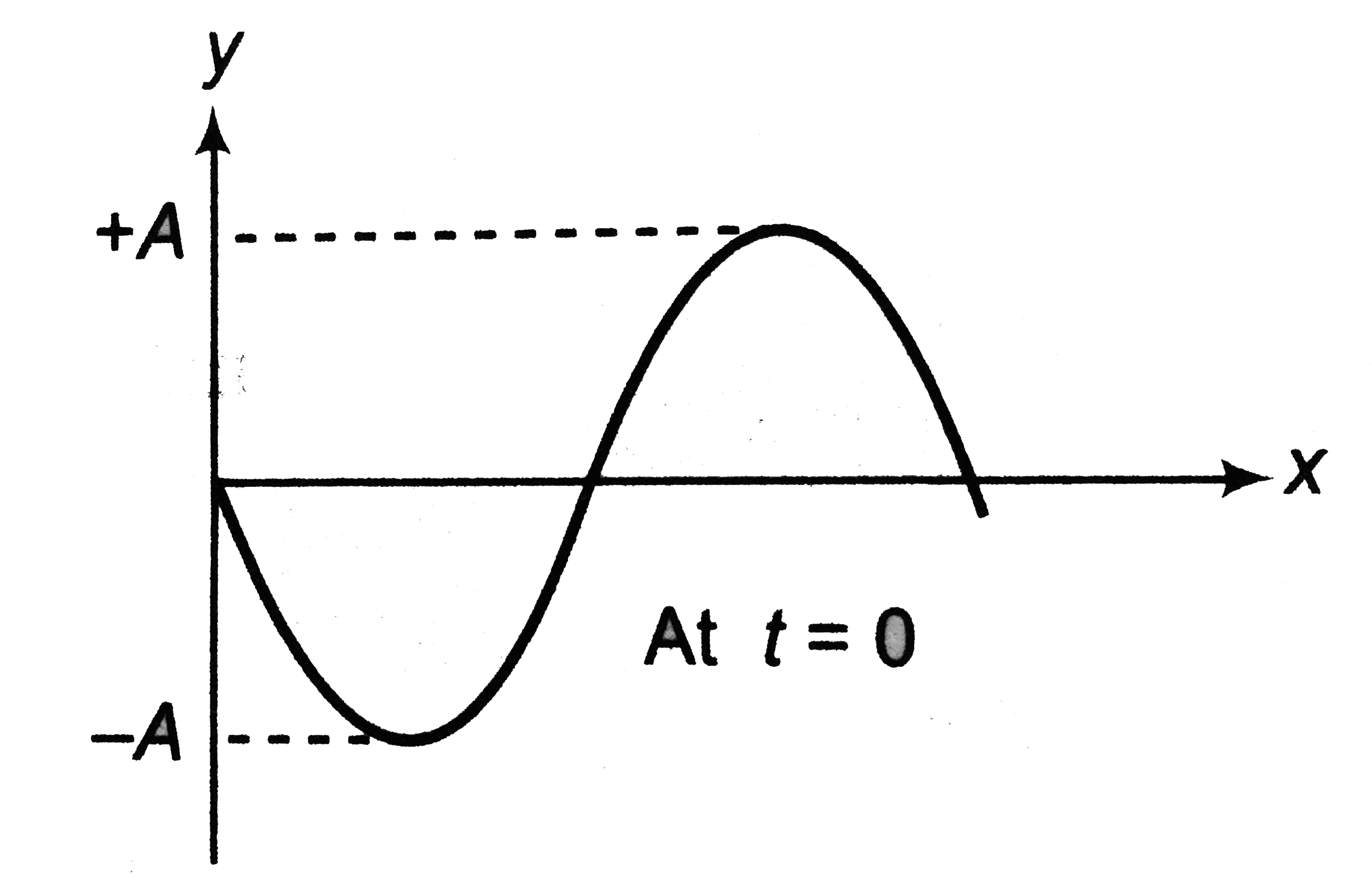
y=Asin(ωt−kx)
Particle velocity vp(x,t)=∂y∂t=ωAcos(ωt−kx) and particle acceleration
ap(x,t)=∂2y∂t=−ω2Asin(ωt−kx)
(a) t=0,x=0:vp=+ωA and ap=0
i.e. particle is moving upward but its acceleration is zero.
(b) t=0, x=π4k x=π4k
∴kx=π4
vp=ωAcos(−π4)=+ωA√2
and ap=−ω2Asin(−π4)=+ω2A√2
Velocity of particle is positive, i.e. the particle is moving upward (along positive y- direction). Further (vP) and (ap)are∈thesamedirection(⊥harepositive).Hence,thepartic≤isspeed∈gup.<br>(C)t =0 , x =(pi)/(2k) , x=(pi)/(2k) :. kx = (pi)/(2)<br>v_(p) =omegaA cos(-pi//2) = 0<br>a_(p) = -omega^(2) A sin (-pi//2) =omega^(2) A<br>i.e.partic≤isstationaryoratitsextermeposition(y = - A).So,itisspeed∈gupatthis∈stant.<br>(d)t =0 , x= (3pi)/(4k) , x = (3pi)/(4k) :. kx = (3pi)/(4)<br>:. v_(p) = omegaA cos (-(3pi)/(4)) = -(omegaA)/(sqrt(2))<br>a_(p) = -omega^(2)A sin(-(3pi)/(4)) = + -(0megaA)/sqrt(2)<br>a_(p) = - omega^(2) A sin (-(3pi)/(4))= + (omega^(2)A )/sqrt(2)<br>Velocityofpartic≤is¬ative,i.e.thepartic≤ismov∈gdownwards.Furtherv_(p)and(a_(P)) are in opposite direction , i.e. the particle is slowing down.
Topper's Solved these Questions
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Exercise 17.1
4 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Exercise 17.2
2 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Example Type 5
1 Videos
- VECTORSBOOK - DC PANDEY ENGLISHCHAPTER - VECTORSEXERCISE - Medical enrances gallery
9 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
Similar Questions
A wave on a string is described by y(x,t)=Acos(kx−ωt). (a) Graph y,vy, and ay as function of x for time t=0. (b) consider the following points on the string: (i) x=0,(ii) x=pi//4k, (iii) x=pi//2k, (iv)x=3pi//4k, (v)x=pi//k, (vi)x=5pi//4k, (vii) x=3pi//2k, (viii) x=7pi//4k. For a particle at each of these points at t=0, describes in words whether the particle is moving and in what direction, and whether the particle is speeding up, slowing down, or instantaneously not acceleraring.
View SolutionA wave is described by y=Asin(ωt−kx). The speeds of which of the following particles in the medium are increasing at time t=0?
View Solution
Knowledge Check
A transverse wave is represented by y=Asin(ωt−kx). For what value of the wavelength is the wave velocity equal to the maximum particle velocity?
AπA2BπAC2πADA
In a wave motion y=asin(kx−ωt), y can represent
View SolutionIn a stationary wave represented by y=asinωtcoskx, amplitude of the component progerssive wave is
View SolutionThe wave described by y =0.25sin(10πx−2πt) where x and y are in meters and t in seconds , is a wave travelling along the
View SolutionA wave y=asin(ωt−kx) on a string meets with another wave producing a node at x=0. Then the equation of the unknown wave is
View Solution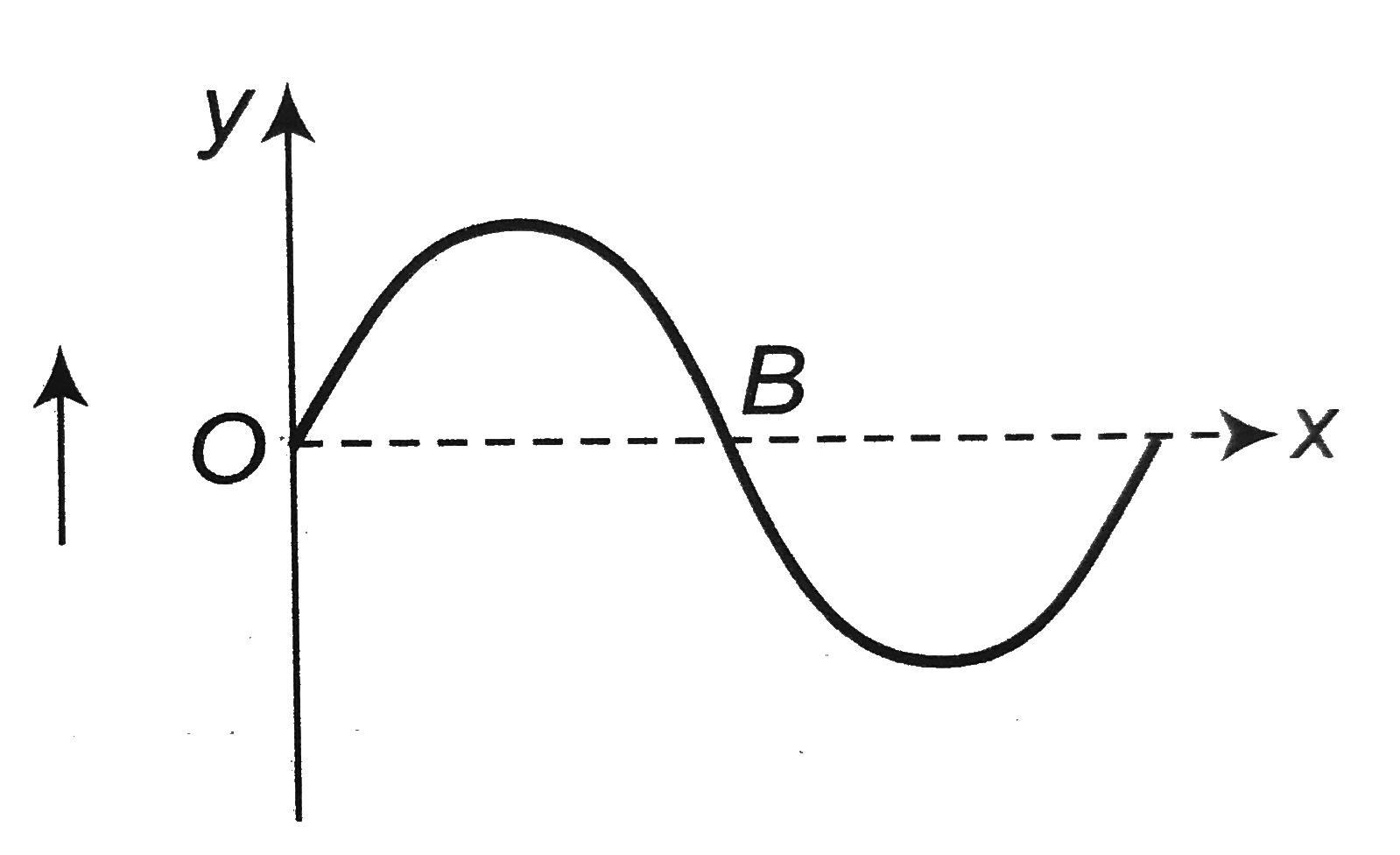
Figure below shows the wave y=Asin(ωt−kx) at any instant travelling in the +ve x-direction. What is the slope of the curve at B?
View SolutionA wave y=Acos(ωt–kx) passes through a medium. If V is the particle velocity and a is the particle acceleration then,
View SolutionThe wave described by y=0.25sin(10πx−2πt) , where x and y are in metres and t in seconds , is a wave travelling along the:
View Solution
