For the one dimensional motion, described by x=t−sint
x(t)>0 for all t>0
v(t)>0 for all t>0
a(t)>−0 for all t>0
v(t) lies between 0 and 2 .
The correct Answer is:(a,d)
Since , x =t - sin t .
v = 1 - cot t
and a = sin t .
x(t)>0 for all t>0
At t=0,v(t)=0. So , v(t) can be zero for one value of t.
At t = 0 and π ,a (t) = 0 .
If t=0,v (t) = 0 , if t=π,v(t)=1−(−1)=2
So , v(t) lies between 0 and 2 .
Here we can see that v(t) is also equal to zero at certain instants and hence is not always greater then zero . So option (b) is not correct .
The correct options are (a) and (d).
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - HIGHER ORDER THINKING SKILL AND ADVANCED LEVEL QUESTION WITH ANSWER
5 Videos
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - REVISION EXERCISE (VERY SHORT ANSWER QUESTION)
40 Videos
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - NCERT (MULTIPLE CHOICE QUESTION (TYPE-I))
6 Videos
- MOTION IN A PLANE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Chapter Practice Test
15 Videos
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Practice Test (For Board Examination)
12 Videos
Similar Questions
The motion of a particle executing SHM in one dimension is described by x=−0.5sin(2t+π/4) where x is in meters and t in seconds. The frequency of oscillation in Hz is
View SolutionFigure gives the x-t plot of a particle is one dimensional motion. Give the signs of position, velocity and acceleration of the particle at t = 0.3s
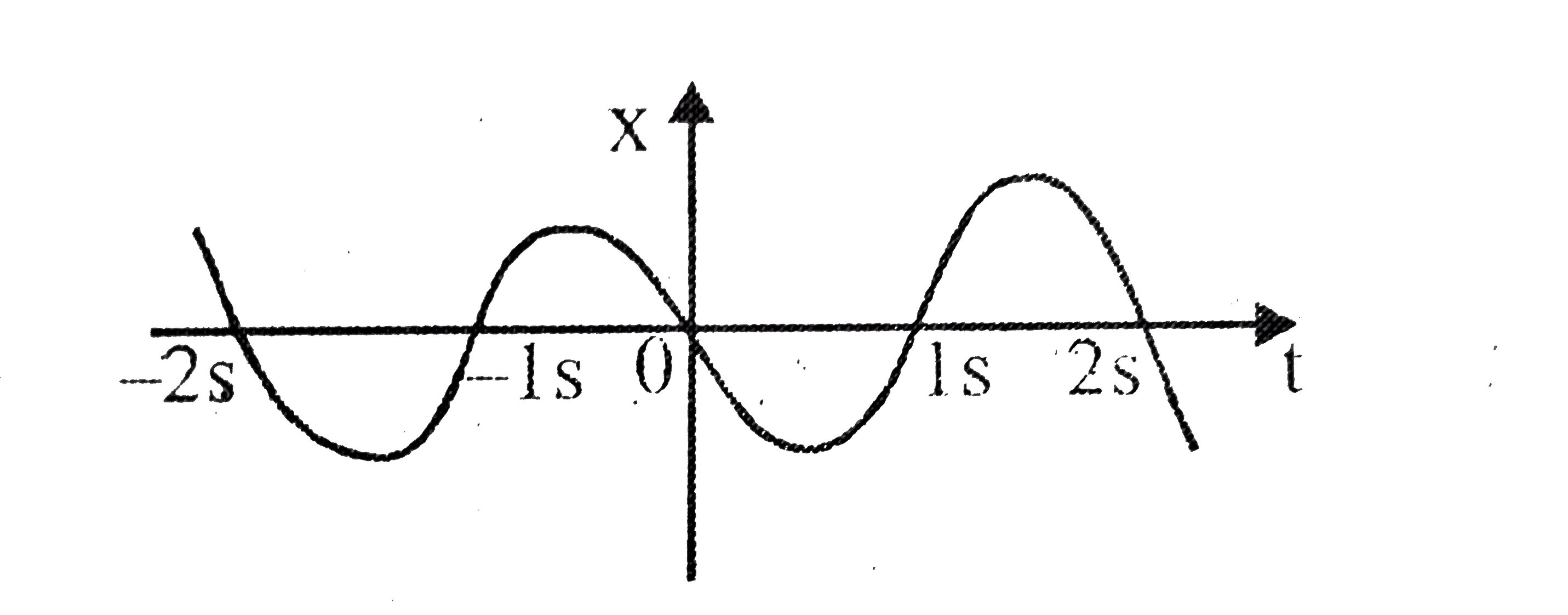
View Solution
Knowledge Check
Force on a particle in one-dimensional motion is given by F=Av+Bt+CxAt+D, where F= force, v= speed, t= time, x= position and A, B, C and D are constants. Dimension of C will be-
AM2L−2T0BML−1T0CM2L0T−2DNone of theseIn the one-dimensional motion of a particle, the relation between position x and time t is given by x2+2x=t (here x>0). Choose the correct statement.
AThe retardation of the particle is 14(x+1)3BThe uniform velocity of the particle is 1(x+1)3CBoth are correctDBoth are wrongIn one dimensional motion, instantaneous speed y satisfies the condition 0 −VoT<x<voT
3) the acceleration is always a 1 - negative number 4) the motion has no turning pointsAthe displacement in time T must always take non- negative valuesBthe displacement in time T satistics : −v0T<x<v0TCthe acceleration is always a non - negative numberDthe motion has no turning points
Which of the following graphs cannot represent one dimensional motion of a particle?
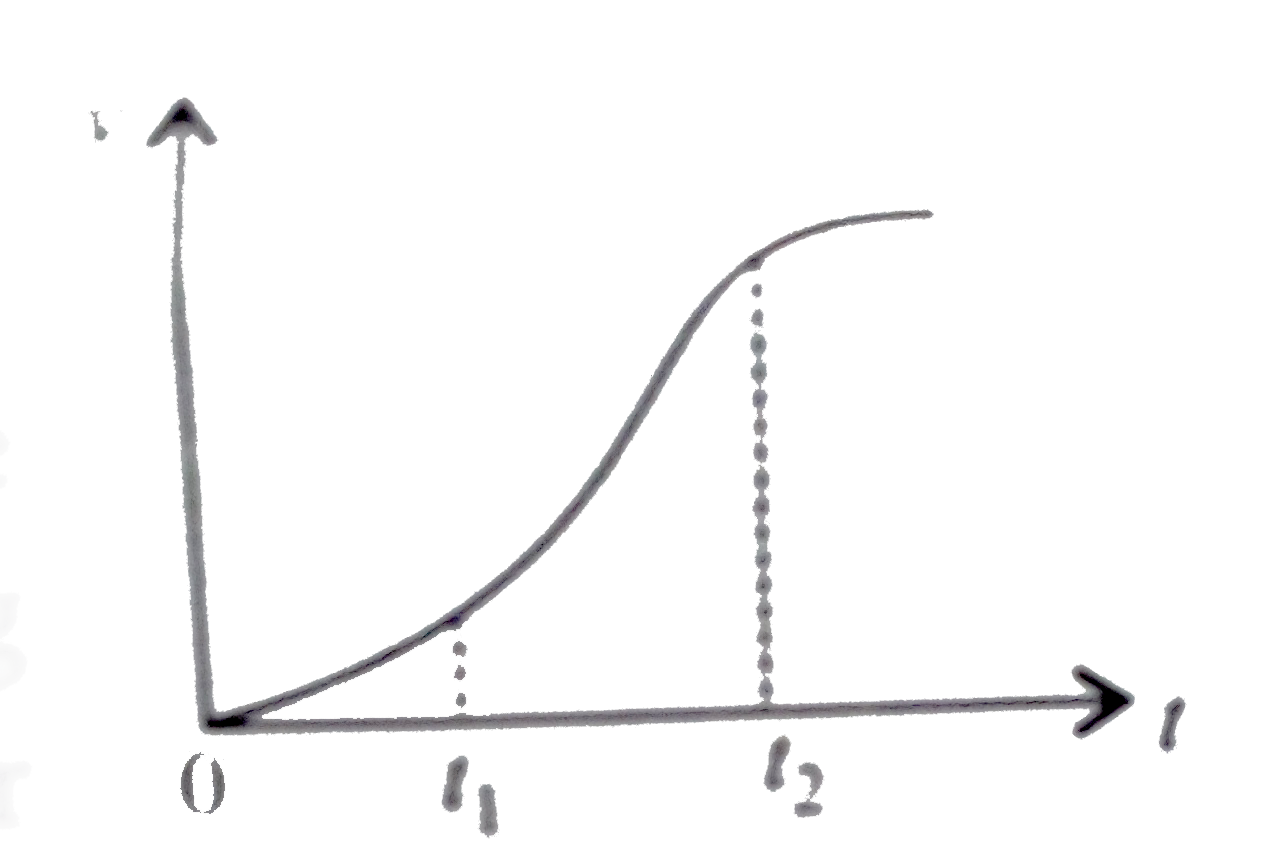
View SolutionThe velocity-time graph of a particle in one-dimensional motion is shown in the figure. Which of the following formulae is correct for describing the motion of the particle over the time interval t1 to t2?
View SolutionDuring one dimensional motion on x-axis Velocity time graph for particle is given.
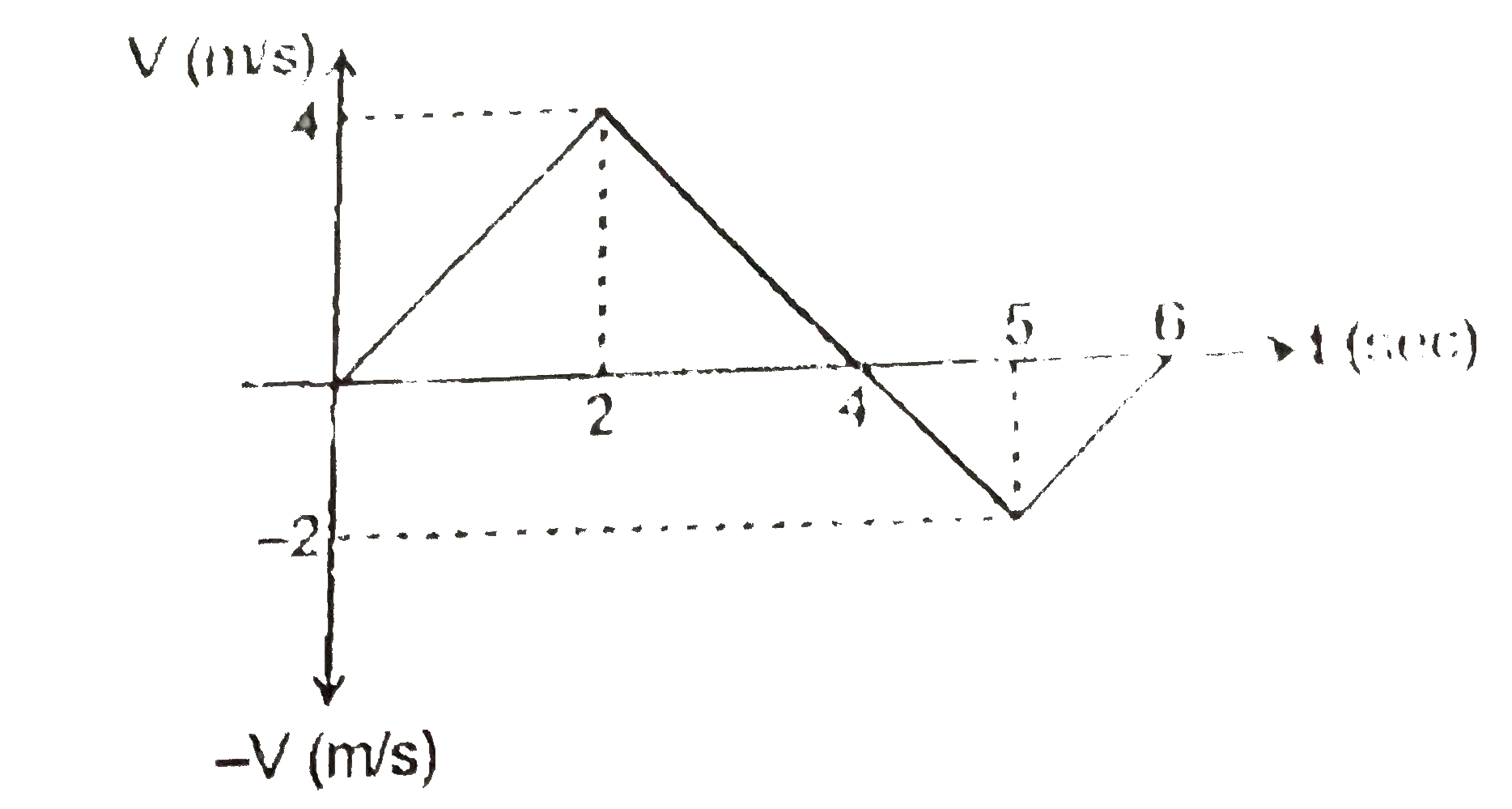
Average acceleration between (t = 0 sec) to (t = 6 sec)
View SolutionA body is initially at rest. It undergoes one dimensional motion with constant acceleration. The power delivered to it at time t is proportional to
View SolutionA body is initially at rest. It undergoes one dimensional motion with constant acceleration. The power delivered to it at time t is proportional to
View Solution
