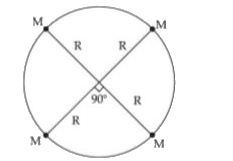
Four particles, each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is:
sqrt((GM)/(R ) (1+2sqrt2))
The correct Answer is:D
To find the speed of each particle moving in a circle under mutual gravitational attraction, we can follow these steps:
Step 1: Understand the Configuration
We have four particles, each of mass M, positioned at the corners of a square with side length R. The distance between any two adjacent particles is R, and the distance between diagonal particles is √2R.
Step 2: Calculate the Gravitational Force
Each particle experiences gravitational attraction from the other three particles. The gravitational force between any two particles is given by:
F=GM2d2
where G is the gravitational constant and d is the distance between the particles.
Step 3: Identify Forces Acting on One Particle
For a particle (say particle A), the forces acting on it due to the other three particles (B, C, and D) need to be considered. The forces from B and C will act at an angle of 45 degrees to the line connecting A to the center of the square.
1. The force between A and B (distance R):
FAB=GM2R2
2. The force between A and C (distance R):
FAC=GM2R2
3. The force between A and D (distance √2R):
FAD=GM2(√2R)2=GM22R2
Step 4: Resolve Forces into Components
The forces FAB and FAC will have components along the radial direction (towards the center of the circle) and tangential direction. Since they are at 45 degrees:
- The radial components of FAB and FAC add up, while the tangential components will cancel out.
The total radial force Fr acting on particle A is:
Fr=FABcos(45∘)+FACcos(45∘)+FAD
=GM2R2⋅1√2+GM2R2⋅1√2+GM22R2
=√2⋅GM2R2+GM22R2
Step 5: Set Up the Centripetal Force Equation
The centripetal force required to keep the particle moving in a circle of radius R is given by:
Fc=Mv2R
Setting the total radial force equal to the centripetal force:
√2⋅GM2R2+GM22R2=Mv2R
Step 6: Solve for Speed v
Rearranging gives:
v2=R(√2⋅GM2R2+GM22R2)
=GM2R(√2+12)
=GM2R(2√2+12)
Taking the square root gives:
v=√GMR⋅2√2+12
Final Answer
Thus, the speed of each particle is:
v=√GMR⋅2√2+12
---
We have four particles, each of mass M, positioned at the corners of a square with side length R. The distance between any two adjacent particles is R, and the distance between diagonal particles is √2R.
Each particle experiences gravitational attraction from the other three particles. The gravitational force between any two particles is given by:
F=GM2d2
where G is the gravitational constant and d is the distance between the particles.
For a particle (say particle A), the forces acting on it due to the other three particles (B, C, and D) need to be considered. The forces from B and C will act at an angle of 45 degrees to the line connecting A to the center of the square.
FAB=GM2R2
FAC=GM2R2
FAD=GM2(√2R)2=GM22R2
The forces FAB and FAC will have components along the radial direction (towards the center of the circle) and tangential direction. Since they are at 45 degrees:
- The radial components of FAB and FAC add up, while the tangential components will cancel out.
Fr=FABcos(45∘)+FACcos(45∘)+FAD
=GM2R2⋅1√2+GM2R2⋅1√2+GM22R2
=√2⋅GM2R2+GM22R2
The centripetal force required to keep the particle moving in a circle of radius R is given by:
Fc=Mv2R
√2⋅GM2R2+GM22R2=Mv2R
Rearranging gives:
v2=R(√2⋅GM2R2+GM22R2)
=GM2R(√2+12)
=GM2R(2√2+12)
v=√GMR⋅2√2+12
Thus, the speed of each particle is:
v=√GMR⋅2√2+12
Topper's Solved these Questions
Similar Questions
Four particles each of mass M, move along a circle of radius R under the action of their mutual gravitational attraction as shown in figure. The speed of each particle is :
View SolutionFor particles of equal massses M move along a circle of radius R under th action of their mutual gravitational attraction. Find the speed of each particle.
View Solution
Knowledge Check
Two particles each of mass M and equidistant from each other move along a circle of radius R under the action of their mutual gravitional attraction the speed of each particle is
A√GM2RB12R√1GMC12√GMRD√4GMRFour particles each of mass M move along a circle of radius R under the action of their mutula gravitational attraction the speed of each paritcles is
A√GMRB√2√2GMRC√GMR(1+2√2)D12√GMR(1+2√2)Four particles each of mass M move along a circle of radius R under the action of their mutula gravitational attraction the speed of each paritcles is
AGmRB√2√2GMRC√GMR(2√2+1)D√GMR2√2+14
Two particles of equal mass go round a circle of radius R under the action of their mutual gravitational attraction. Find the speed of each particle.
View Solution_S01_003_Q01.png)
Two particles of equal mass (m) each move in a circle of radius (r) under the action of their mutual gravitational attraction find the speed of each particle.
View SolutionTwo particles of same mass m go around a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is ,
View SolutionTwo particles of equal mass go around a circle of radius R under the action of their mutual gravitational attraction. The speed v of earth particle is
View SolutionTwo particles of equal mass m go around a circle of radius R under the action the of their mutual gravitational attraction . The speed v of each particle is
View Solution
