Four particles, each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is: Solution in Kannada
12√GMR(1+2√2)
√GMR
√2√2GMR
√GMR(1+2√2)
The correct Answer is:A
Net force on any one particle

=GM2(2R)2+GM2(R√2)2cos45∘+GM2(R√2)2cos45∘
=GM2R2[14+1√2]
This force will be equal to centripetal force so
Mu2R=GM2R2[1+2√24]
u=√GM4R[1+2√2]=12√GMR[2√2+1]
So correct choice is (a).
Topper's Solved these Questions
- GRAVITATIONBOOK - MODERN PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - Recent Competitive Questions
8 Videos
- GRAVITATIONBOOK - MODERN PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - Multiple Choice Questions (LEVEL-II)
64 Videos
- ELECTROSTATICSBOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - RECENT COMPETITIVE QUESTIONS
39 Videos
- HEAT AND THERMODYNAMICSBOOK - MODERN PUBLICATIONCHAPTER - HEAT AND THERMODYNAMICSEXERCISE - Recent Competitve Questions
17 Videos
Similar Questions
An astronaut on a strange planet finds that acceleration due to gravity is twice as that on the surface of earth. Which of the following could explain thin ?
View SolutionIn order that a body of 15 kg weighs zero at the equator, then the angular speed of earth is :
(g=10ms−1)
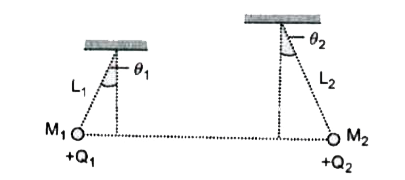
View SolutionTwo small spheres of masses M1andM2 are suspended by weightless insulating threads of lengths L1andL2 The spheres carry charges Q1andq2 respectively . The spheres are suspended such that they are in level with one another and the threads are inclined to the vertical at angles of θ1andθ2 as shown . Which one o fthe following conditions , if θ1=θ2 ?
View SolutionThe temperature of a gas contained in a closed vessel of constant volume increases by 1∘C when the pressure of the gas is increased by 1%. The initial temperature of the gas is:
View SolutionFour particles, each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is:
View SolutionTwo particles each of mass M and equidistant from each other move along a circle of radius R under the action of their mutual gravitional attraction the speed of each particle is
View SolutionFour particles, each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. Calculate the speed of each particle
View SolutionFour particles, each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. Calculate the speed of each particle
View SolutionFour particles, each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. Calculate the speed of each particle
View SolutionFour particles, each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction, the speed of each particle is
View SolutionFour particles, each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. Calculate the speed of each particle
View SolutionFour particles, each of mass M and equidistant "from each other, move along a circle of radius R under the action of the mutual gravitational attraction. The speed of each particle is ......... (G = universal gravitational constant)
View SolutionFour particles, each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is:
View SolutionFour particles , each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is:
View SolutionFour particles , each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is:
View Solution
