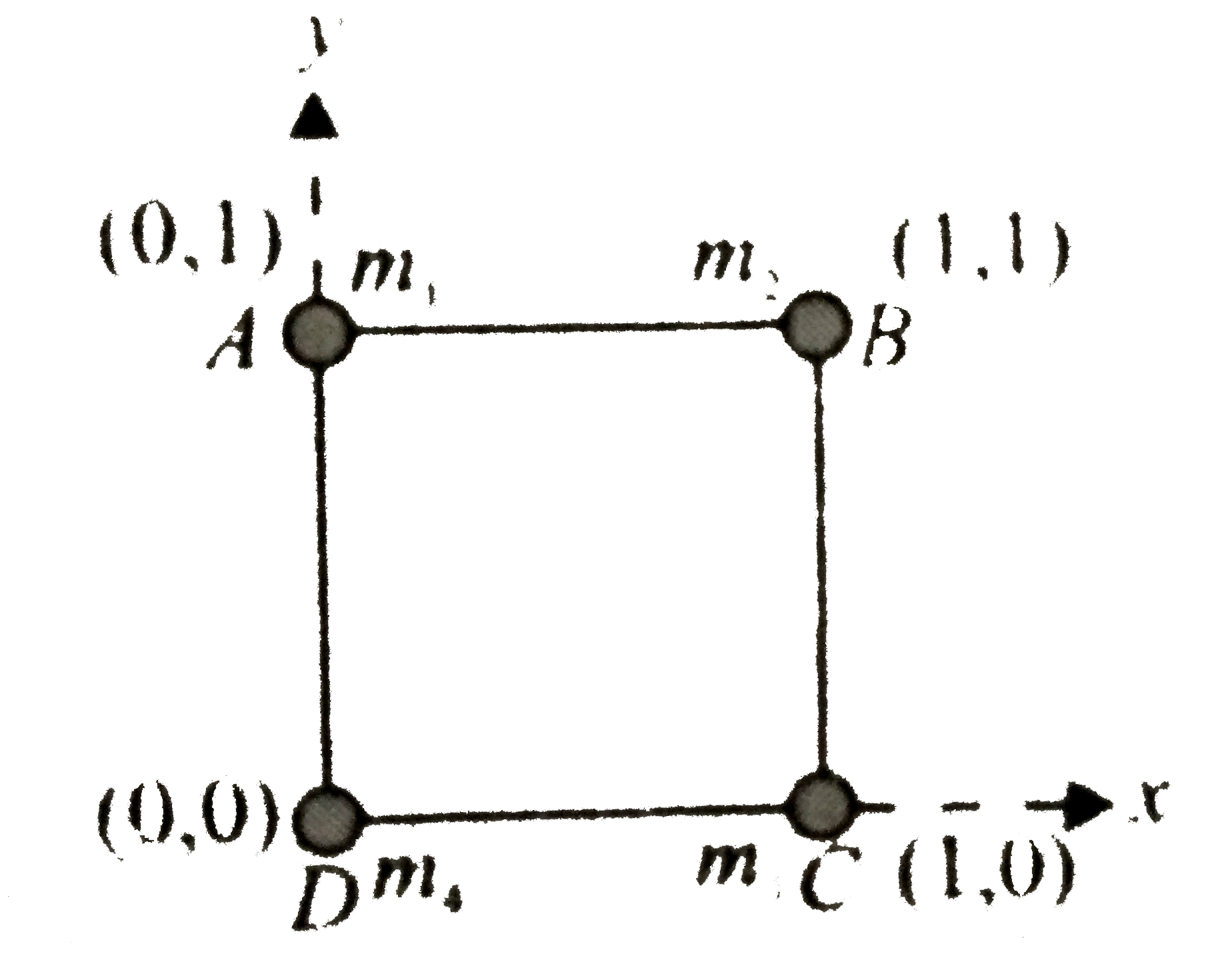
Four particles of masses 1kg, 2kg, 3kg and 4kg are placed at the four vertices A, B, C and D of a square of side 1m. Find the position of centre of mass of the particles. Assume that D is situated at the origin.
To find the position of the center of mass of the four particles placed at the vertices of a square, we can follow these steps:
Step 1: Identify the coordinates of the vertices and the masses
We have four particles with the following masses and coordinates:
- Mass mA=1kg at vertex A (0, 1)
- Mass mB=2kg at vertex B (1, 1)
- Mass mC=3kg at vertex C (1, 0)
- Mass mD=4kg at vertex D (0, 0)
Step 2: Write down the formula for the center of mass
The center of mass →Rcm can be calculated using the formula:
→Rcm=mA→rA+mB→rB+mC→rC+mD→rDmA+mB+mC+mD
where →rA,→rB,→rC,→rD are the position vectors of the particles.
Step 3: Calculate the total mass
The total mass M is:
M=mA+mB+mC+mD=1+2+3+4=10kg
Step 4: Calculate the x-coordinate of the center of mass
The x-coordinate of the center of mass is calculated as follows:
xcm=mA⋅xA+mB⋅xB+mC⋅xC+mD⋅xDM
Substituting the values:
xcm=(1⋅0)+(2⋅1)+(3⋅1)+(4⋅0)10=0+2+3+010=510=0.5
Step 5: Calculate the y-coordinate of the center of mass
The y-coordinate of the center of mass is calculated similarly:
ycm=mA⋅yA+mB⋅yB+mC⋅yC+mD⋅yDM
Substituting the values:
ycm=(1⋅1)+(2⋅1)+(3⋅0)+(4⋅0)10=1+2+0+010=310=0.3
Step 6: Combine the coordinates to find the center of mass
Thus, the position of the center of mass is:
→Rcm=(xcm,ycm)=(0.5,0.3)
Final Answer
The position of the center of mass of the particles is (0.5,0.3).
---
We have four particles with the following masses and coordinates:
- Mass mA=1kg at vertex A (0, 1)
- Mass mB=2kg at vertex B (1, 1)
- Mass mC=3kg at vertex C (1, 0)
- Mass mD=4kg at vertex D (0, 0)
The center of mass →Rcm can be calculated using the formula:
→Rcm=mA→rA+mB→rB+mC→rC+mD→rDmA+mB+mC+mD
where →rA,→rB,→rC,→rD are the position vectors of the particles.
The total mass M is:
M=mA+mB+mC+mD=1+2+3+4=10kg
The x-coordinate of the center of mass is calculated as follows:
xcm=mA⋅xA+mB⋅xB+mC⋅xC+mD⋅xDM
Substituting the values:
xcm=(1⋅0)+(2⋅1)+(3⋅1)+(4⋅0)10=0+2+3+010=510=0.5
The y-coordinate of the center of mass is calculated similarly:
ycm=mA⋅yA+mB⋅yB+mC⋅yC+mD⋅yDM
Substituting the values:
ycm=(1⋅1)+(2⋅1)+(3⋅0)+(4⋅0)10=1+2+0+010=310=0.3
Thus, the position of the center of mass is:
→Rcm=(xcm,ycm)=(0.5,0.3)
The position of the center of mass of the particles is (0.5,0.3).
Topper's Solved these Questions
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Check points
50 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Taking it together
73 Videos
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery
38 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
Similar Questions
Four particles of masses 1kg,2kg,3kg and 4kg are placed the four vertices A,B,C and D, respectively, of a square of side 1m. Find the position of centre of mass of the particles.
View SolutionFour particles of masses 1kg, 2kg, 3kg and 4kg are placed at the four vertices A,B,C and D of a square of side 1m. Find square of distance of their centre of mass from A.
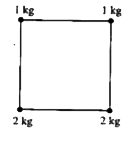
View SolutionFour point masses 1 kg, 1 kg, 2 kg and 2 kg are placed at the corners of a square of side a m as shown in Fig. Find the centre of mass of the system.
View SolutionThree particles of masses 1kg, 2kg and 3kg are placed at the corners A, B and C respectively of an equilateral triangle ABC of edge 1m. Find the distance of their centre of mass from A.
View SolutionFour particles having masses, m, 2m, 3m, and 4m are placed at the four corners of a square of edge a. Find the gravitational force acting on a particle of mass m placed at the centre.
View SolutionFour particles having masses, m, 2m, 3m, and 4m are placed at the four corners of a square of edge a. Find the gravitational force acting on a particle of mass m placed at the centre.
View SolutionFour particles having masses, m, 2m, 3m, and 4m are placed at the four corners of a square of edge a. Find the gravitational force acting on a particle of mass m placed at the center.
View SolutionThree particles of masses 1.0 kg 2.0 kg and 3.0 kg are placed at the corners A,B and C respectively of an equilateral triangle ABC of edge 1m. Locate the centre of mass of the system.
View Solution4 Masses 8 kg, 2 kg, 4 kg and 2 kg are placed at the corners A, B, C, D respectively of a square ABCD of diagonal 80 cm. The distance of centre of mass from A will be
View SolutionFind the potential energy of 4-particles, each of mass 1 kg placed at the four vertices of a square of side length 1 m.
View Solution
