How does the angle of deviation formed by a prism change with the increase in the angle of incidence ?
Draw a graph showing the variation in the angle of deviation with the angle of incidence at a prism surface.
To understand how the angle of deviation formed by a prism changes with the increase in the angle of incidence, we can follow these steps:
Step 1: Understanding the Terms
- Angle of Incidence (I): The angle at which the incoming light ray strikes the prism surface.
- Angle of Deviation (δ): The angle between the direction of the incoming ray and the direction of the outgoing ray after passing through the prism.
Step 2: Observing the Behavior of Light in a Prism
When light enters a prism, it bends towards the base of the prism due to refraction. The amount of bending depends on the angle at which the light hits the prism.
Step 3: Analyzing the Graph
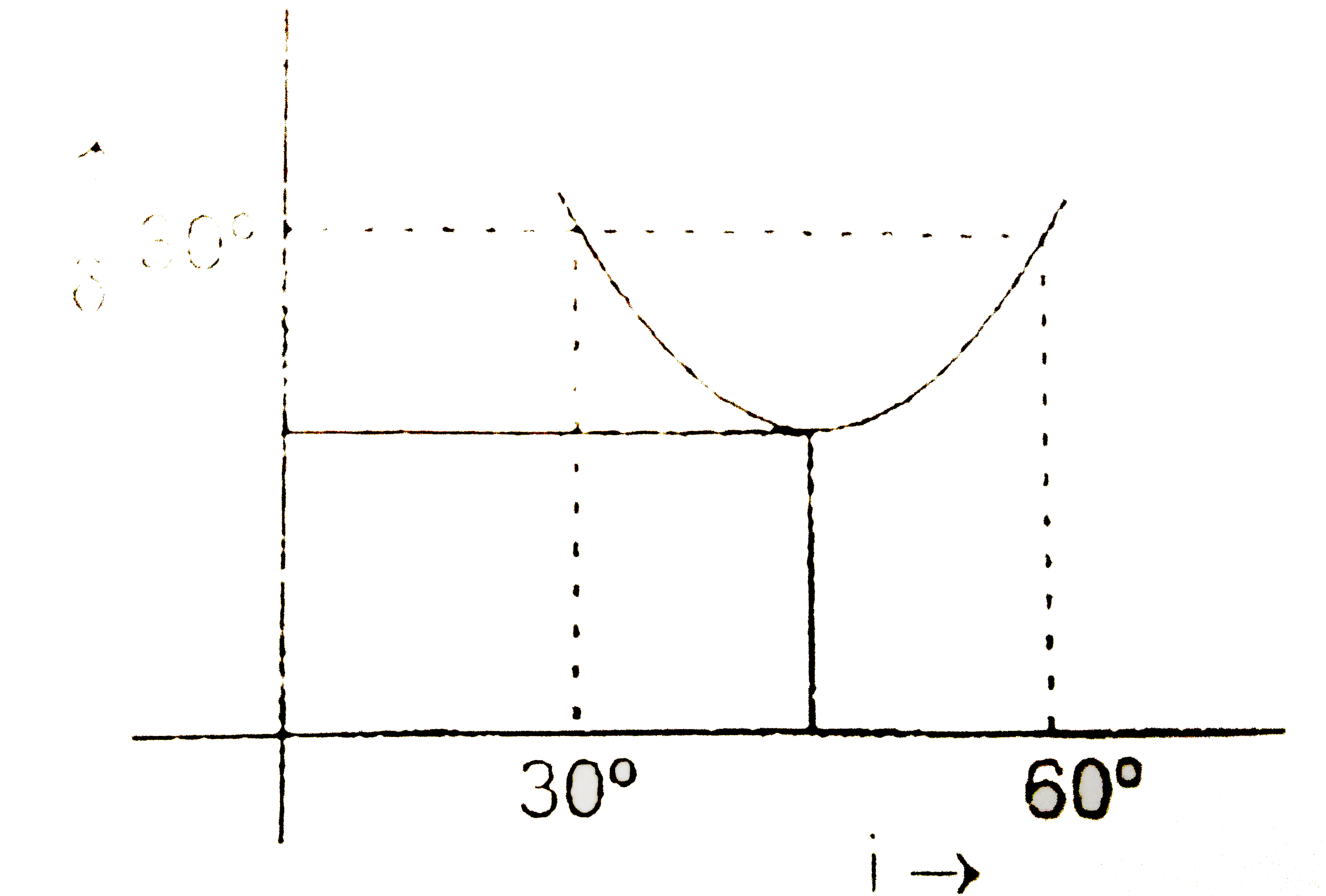
1. Graph Axes:
- The x-axis represents the angle of incidence (I).
- The y-axis represents the angle of deviation (δ).
2. Graph Shape:
- Initially, as the angle of incidence increases from zero, the angle of deviation decreases.
- There is a specific angle of incidence (I_min) where the angle of deviation reaches its minimum (δ_min).
- After this point, as the angle of incidence continues to increase, the angle of deviation starts to increase again.
Step 4: Drawing the Graph
- Start by plotting the angle of incidence (I) on the x-axis.
- Plot the angle of deviation (δ) on the y-axis.
- Draw a curve that decreases to a minimum point and then increases, indicating the relationship between the two angles.
Step 5: Conclusion
The relationship can be summarized as follows:
- As the angle of incidence increases from zero, the angle of deviation decreases until it reaches a minimum value (δ_min).
- Beyond this minimum angle of incidence, any further increase in the angle of incidence results in an increase in the angle of deviation.
- Angle of Incidence (I): The angle at which the incoming light ray strikes the prism surface.
- Angle of Deviation (δ): The angle between the direction of the incoming ray and the direction of the outgoing ray after passing through the prism.
When light enters a prism, it bends towards the base of the prism due to refraction. The amount of bending depends on the angle at which the light hits the prism.
1. Graph Axes:
- The x-axis represents the angle of incidence (I).
- The y-axis represents the angle of deviation (δ).
2. Graph Shape:
- Initially, as the angle of incidence increases from zero, the angle of deviation decreases.
- There is a specific angle of incidence (I_min) where the angle of deviation reaches its minimum (δ_min).
- After this point, as the angle of incidence continues to increase, the angle of deviation starts to increase again.
- Start by plotting the angle of incidence (I) on the x-axis.
- Plot the angle of deviation (δ) on the y-axis.
- Draw a curve that decreases to a minimum point and then increases, indicating the relationship between the two angles.
The relationship can be summarized as follows:
- As the angle of incidence increases from zero, the angle of deviation decreases until it reaches a minimum value (δ_min).
- Beyond this minimum angle of incidence, any further increase in the angle of incidence results in an increase in the angle of deviation.
Topper's Solved these Questions
Similar Questions
(a) How does the angle of deviation produced by a prism change with increase in the angle of incidence. Draw a curve showing the variation in the angle of deviation with the angle of incidence at a prism surface.
(b) Using the curve in part (a) above, how do you infer that for a given prism, the angle of minimum deviation δmin is unique for a given light.
View SolutionHow does the angle of minimum deviation produced by a prism change with increase in (i) the wavelength of incident light, and (ii) the refracting angle of prism ?
View SolutionHow does the angle of deviation depend on the refracting angle of the prism?
View Solution(a) Deduce the expression, by drawing a suitable ray diagram, for the refractive index of a triangular glass prisms in terms of the angle of minimum deviation (D) and the angle of prism (A).
Draw a plot showing the variation of the angle of deviation with the angle of incidence.
(b) Calculate the value of the angle of incidence when a ray of light incident on one face of an equilateral glass prism produces the emergent ray, which just grazes along the adjacent face. Refractive index of prism is √2
View SolutionFrom the graph fo angle of deviation δ versus angle of incidwence i. Find the prism angle
View SolutionDefine refractive index of a transparent medium. A ray of light passes through a triangular prism. Plot a graph showing the variation of angle of deviation with the angle of incidence.
View SolutionA light ray of angles of incidence 40∘ emerged from the prism in minimum deviation position. Then what is the angle of incidence on the second surface ?
View SolutionHow does the angle of minimum deviation of a glass prism vary, if the incident violet light is replaced with red light?
View SolutionHow does the angle of minimum deviation of a glass prism vary, if the incident violet light is replaced with red light?
View SolutionIn a regular prism, what is the relation between angle of incidence and angle of emergence when it is in the minimum deviation position ?
View Solution
