Derive a relationship between S.I. and C.G.S. unit of work.
To derive the relationship between the S.I. unit of work (Joule) and the C.G.S. unit of work (Erg), we will follow these steps:
Step 1: Define the S.I. unit of work
The S.I. unit of work is defined as:
1 Joule (J)=1 Newton (N)×1 meter (m)
Step 2: Express Newton in terms of C.G.S. units
We know that:
1 Newton=105 dyne
where dyne is the C.G.S. unit of force.
Step 3: Express meter in terms of centimeters
We also know that:
1 meter=100 centimeters (cm)
Step 4: Substitute the values into the Joule definition
Now, substituting the values from Steps 2 and 3 into the definition of Joule:
1 Joule=(105 dyne)×(100 cm)
Step 5: Calculate the value
Now we can calculate:
1 Joule=105×100 dyne cm
1 Joule=105×102 dyne cm
1 Joule=107 dyne cm
Step 6: Relate Joules to Ergs
Since 1 dyne cm=1 Erg, we can conclude:
1 Joule=107 Erg
Final Relationship
Thus, the relationship between the S.I. unit of work (Joule) and the C.G.S. unit of work (Erg) is:
1 Joule=107 Erg
---
The S.I. unit of work is defined as:
1 Joule (J)=1 Newton (N)×1 meter (m)
We know that:
1 Newton=105 dyne
where dyne is the C.G.S. unit of force.
We also know that:
1 meter=100 centimeters (cm)
Now, substituting the values from Steps 2 and 3 into the definition of Joule:
1 Joule=(105 dyne)×(100 cm)
Now we can calculate:
1 Joule=105×100 dyne cm
1 Joule=105×102 dyne cm
1 Joule=107 dyne cm
Since 1 dyne cm=1 Erg, we can conclude:
1 Joule=107 Erg
Thus, the relationship between the S.I. unit of work (Joule) and the C.G.S. unit of work (Erg) is:
1 Joule=107 Erg
Topper's Solved these Questions
Similar Questions
Knowledge Check
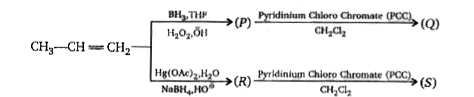
Relationship between products (Q) and (S) is:APositional isomerBChain isomerCStereoisomerDFunctional isomer
Write the relationship between the SI and CGS unit of moment of force.
View SolutionExpress the relationship between the C.G.S. and S.I. units of density.
View SolutionS.I. & C.I
View SolutionHow the S.I. unit and C.G.S. unit of force are related ?
View SolutionWhat do you understand by KpandKc ? Derive a relationship between the two.
View SolutionState and define the S.I. unit of work.
View SolutionFind the zeros of polynomial g(s)=4s2−4s+1 and verify the relationship between the zeros and their coefficients:
View Solution
