If a compressed spring is dissolved in acid, what happens to the elastic potential energy of the spring ?
To understand what happens to the elastic potential energy of a compressed spring when it is dissolved in acid, we can break down the process into a series of steps:
1. Understanding Elastic Potential Energy:
- A compressed spring stores elastic potential energy due to the deformation of its shape. This energy is a result of the spring being under stress from compression.
2. Dissolving the Spring in Acid:
- When the compressed spring is introduced to acid, a chemical reaction occurs. The acid interacts with the material of the spring, leading to its dissolution.
3. Energy Transformation:
- As the spring dissolves, the elastic potential energy that was stored in the spring is released. This energy does not just disappear; instead, it is transformed into another form of energy.
4. Conversion to Kinetic Energy:
- The stored elastic potential energy is converted into kinetic energy. This kinetic energy is associated with the movement of the molecules that are formed as the spring dissolves in the acid.
5. Increase in Temperature:
- The kinetic energy gained by the molecules contributes to an increase in the temperature of the acid solution. This is because the motion of the molecules increases, which is perceived as an increase in thermal energy or heat.
6. Final Conclusion:
- Therefore, we can conclude that the elastic potential energy of the compressed spring is converted into heat energy as the spring dissolves in acid, leading to an increase in the temperature of the acid.
Summary:
When a compressed spring is dissolved in acid, its elastic potential energy is transformed into kinetic energy and subsequently into heat energy, resulting in a slight increase in the temperature of the acid.
---
- A compressed spring stores elastic potential energy due to the deformation of its shape. This energy is a result of the spring being under stress from compression.
- When the compressed spring is introduced to acid, a chemical reaction occurs. The acid interacts with the material of the spring, leading to its dissolution.
- As the spring dissolves, the elastic potential energy that was stored in the spring is released. This energy does not just disappear; instead, it is transformed into another form of energy.
- The stored elastic potential energy is converted into kinetic energy. This kinetic energy is associated with the movement of the molecules that are formed as the spring dissolves in the acid.
- The kinetic energy gained by the molecules contributes to an increase in the temperature of the acid solution. This is because the motion of the molecules increases, which is perceived as an increase in thermal energy or heat.
- Therefore, we can conclude that the elastic potential energy of the compressed spring is converted into heat energy as the spring dissolves in acid, leading to an increase in the temperature of the acid.
When a compressed spring is dissolved in acid, its elastic potential energy is transformed into kinetic energy and subsequently into heat energy, resulting in a slight increase in the temperature of the acid.
Topper's Solved these Questions
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Objective-1
29 Videos
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Objective-2
7 Videos
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Exercises
32 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Question for short Answer
15 Videos
- SOUND WAVESBOOK - HC VERMA ENGLISHCHAPTER - SOUND WAVESEXERCISE - All Questions
133 Videos
Similar Questions
"A mass is attached to one end of a spring and by holding the other end in hand, the spring is whirled in a horizontal circle" What happens to the length of the spring ?
View SolutionA compressed spring of an air gun has kinetic energy.
View SolutionIn figure, k=100N/m,M=1kg and F=10N
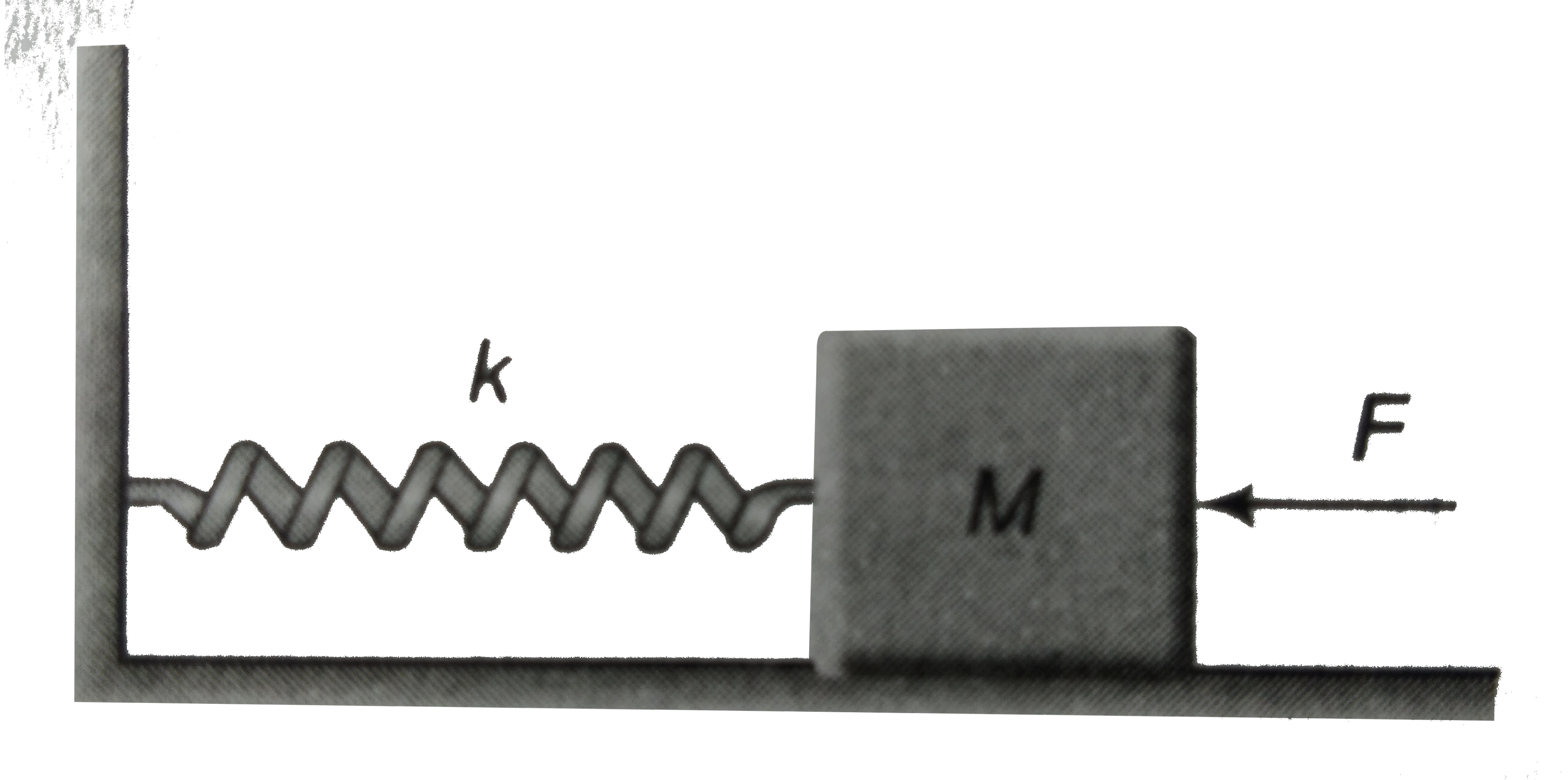
(a) Find the compression of the spring in the equilibrium position
(b) A sharp blow by some external agent imparts a speed of 2m/s to the block towards left. Find the sum of the potential energy of the spring and the kinetic energy of the block at this instant.
(c) Find the time period of the resulting simple harmonic motion.
(d) Find the amplitude.
(e) Write the potential energy of the spring when the block is at the left estreme.
(f) Write the potential energy of the spring when the block is at the right extreme.
The answers of (b), (e) and (f) are different. Explain why this does not violate the principle of conservation of energy ?
View SolutionTwo masses m and 2m are attached to two ends of an ideal spring as shown in figure. When the spring is in the compressed state, the energy of the spring is 60 J, if the spring is released, then at its natural length

View SolutionAnswer the question briefly and to the point:
What will happen to the potential energy of the atoms of a solid when compressed? On stretching a wire ?
View SolutionA block is fully submerged in a vessel filled with water by a spring attached to the bottom of the vessel. In equilibrium position spring is compressed. If the vessel now moves downwards with an acceleration a(<g). What happens to the length of the spring.?
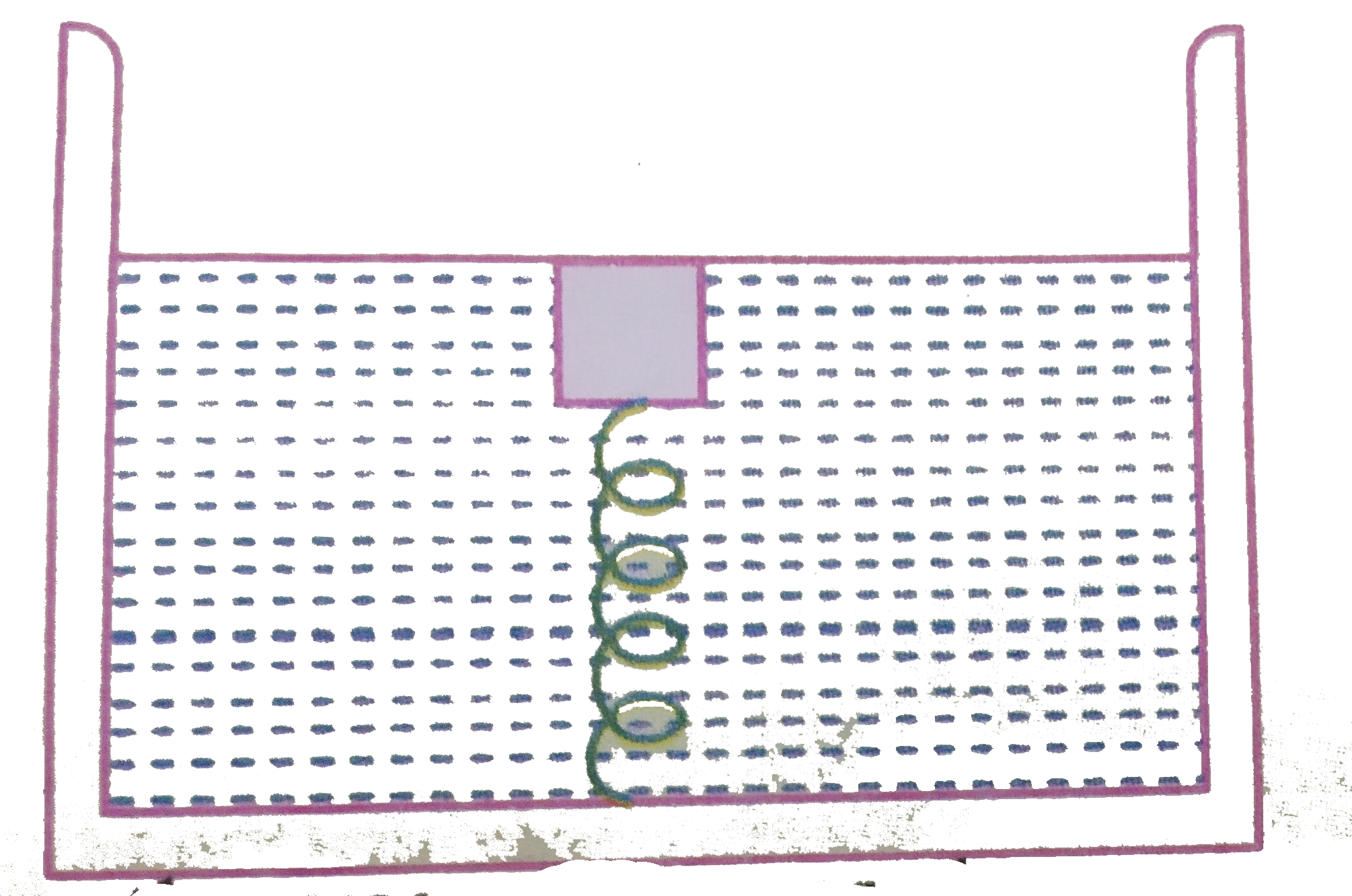
View SolutionA spring is kept compressed by a small trolley of mass ring 0-5 kg lying on a smooth horizontal surface as shown in the adjacent Fig. When the trolley is released, it is found to move at a speed = 2 m s−1. What potential energy did the spring poses when compressed?

View SolutionThe noble gases found dissolved in spring water are
View SolutionA force of 10 N holds an ideal spring with a 20Nm spring constant in compression. The potential energy stored in the spring is
View SolutionA block of mass m is initially moving to the right on a horizontal frictionless surface at a speed v. It then compresses a spring of spring constant k. At the instant when the kinetic energy of the block is equal to the potential energy of the spring, the spring is compressed a distance of :
View Solution
