If the temperature of the body θ and time of cooling 't' and room temperature of the surrounding θ0. The cooling curve is


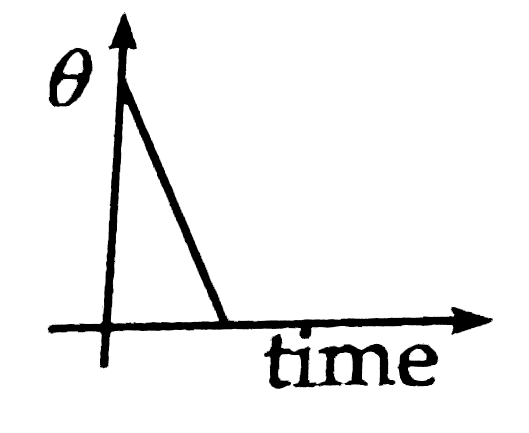
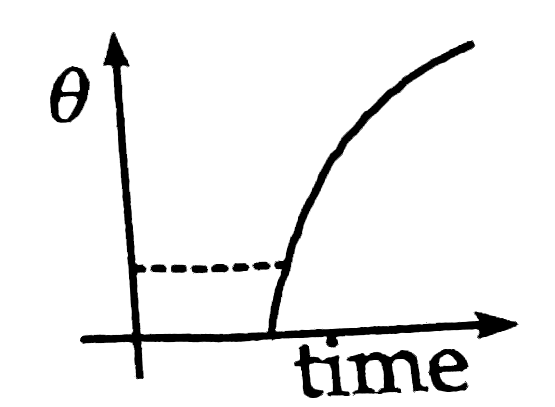
The correct Answer is:B
Topper's Solved these Questions
- KINETIC THEORY OF GASES & RADIATION BOOK - NIKITA PUBLICATIONCHAPTER - KINETIC THEORY OF GASES & RADIATION EXERCISE - MCQs (Question Given in MHT-CET)
79 Videos
- KINETIC THEORY OF GASES & RADIATION BOOK - NIKITA PUBLICATIONCHAPTER - KINETIC THEORY OF GASES & RADIATION EXERCISE - MCQs (Stefans Law of Black Body Radiation)
52 Videos
- INTERFERENCE AND DIFFRACTIONBOOK - NIKITA PUBLICATIONCHAPTER - INTERFERENCE AND DIFFRACTIONEXERCISE - MULTPLE CHOICE QUESTIONS
333 Videos
- MAGNETIC EFFECT OF ELECTRIC CURRENTBOOK - NIKITA PUBLICATIONCHAPTER - MAGNETIC EFFECT OF ELECTRIC CURRENTEXERCISE - MCQs (Sensitivity and accuracy of M.C.G.)
1 Videos
Similar Questions
Themperature of a body θ is slightly more than the temperature of the surrounding θ0 its rate of cooling (R) versus temperature of body (θ) is plotted its shape would be .
View SolutionThe temperature of water in calorimeter is θ and the temperature of surrounding is θ0(<θ). Observation are recorded for temperature difference (θ−θ0) and time t. The cooling curve is best represented in graph.
View Solution
Knowledge Check
Themperature of a body θ is slightly more than the temperature of the surrounding θ0 its rate of cooling (R) versus temperature of body (θ) is plotted its shape would be .
A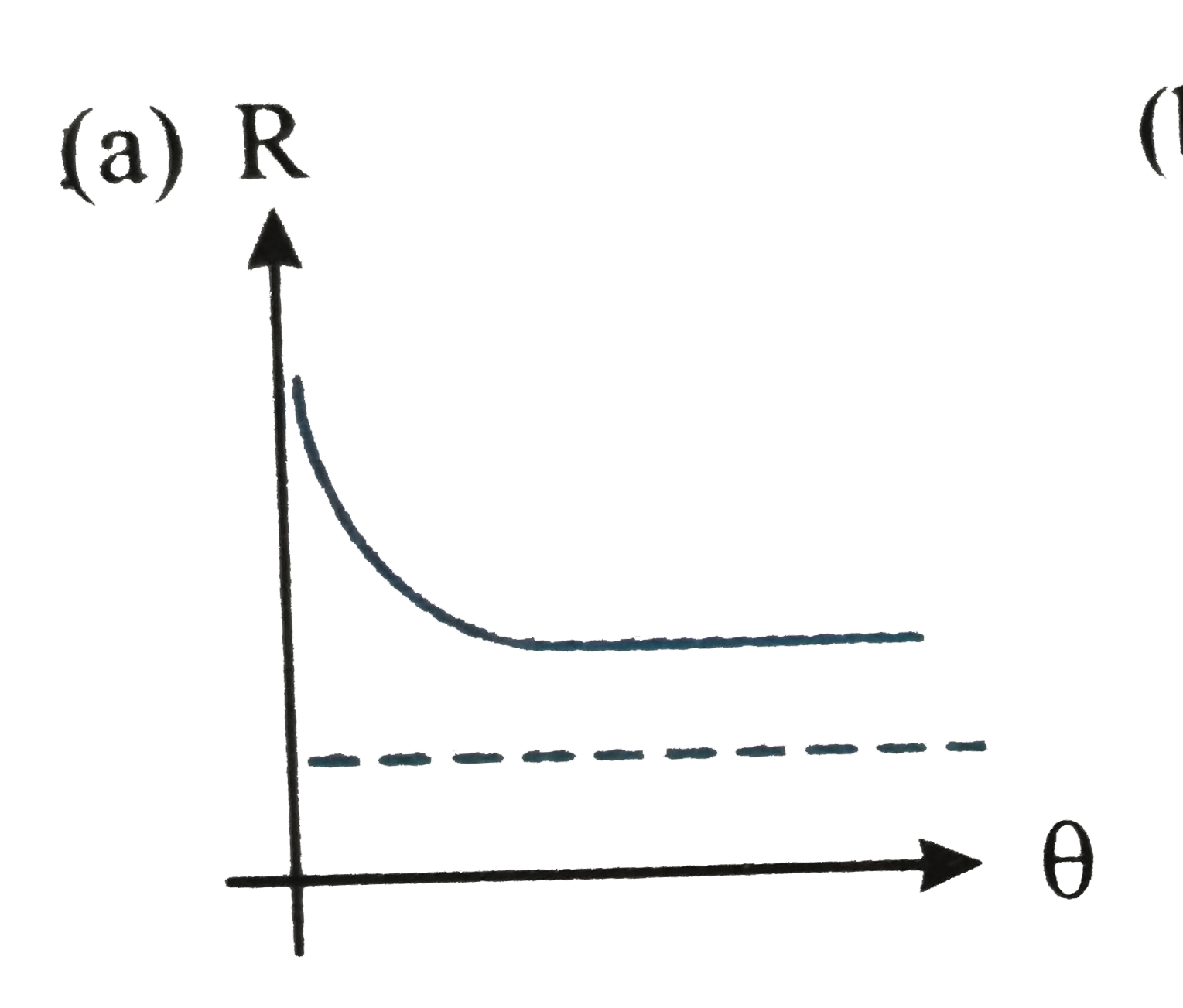 B
B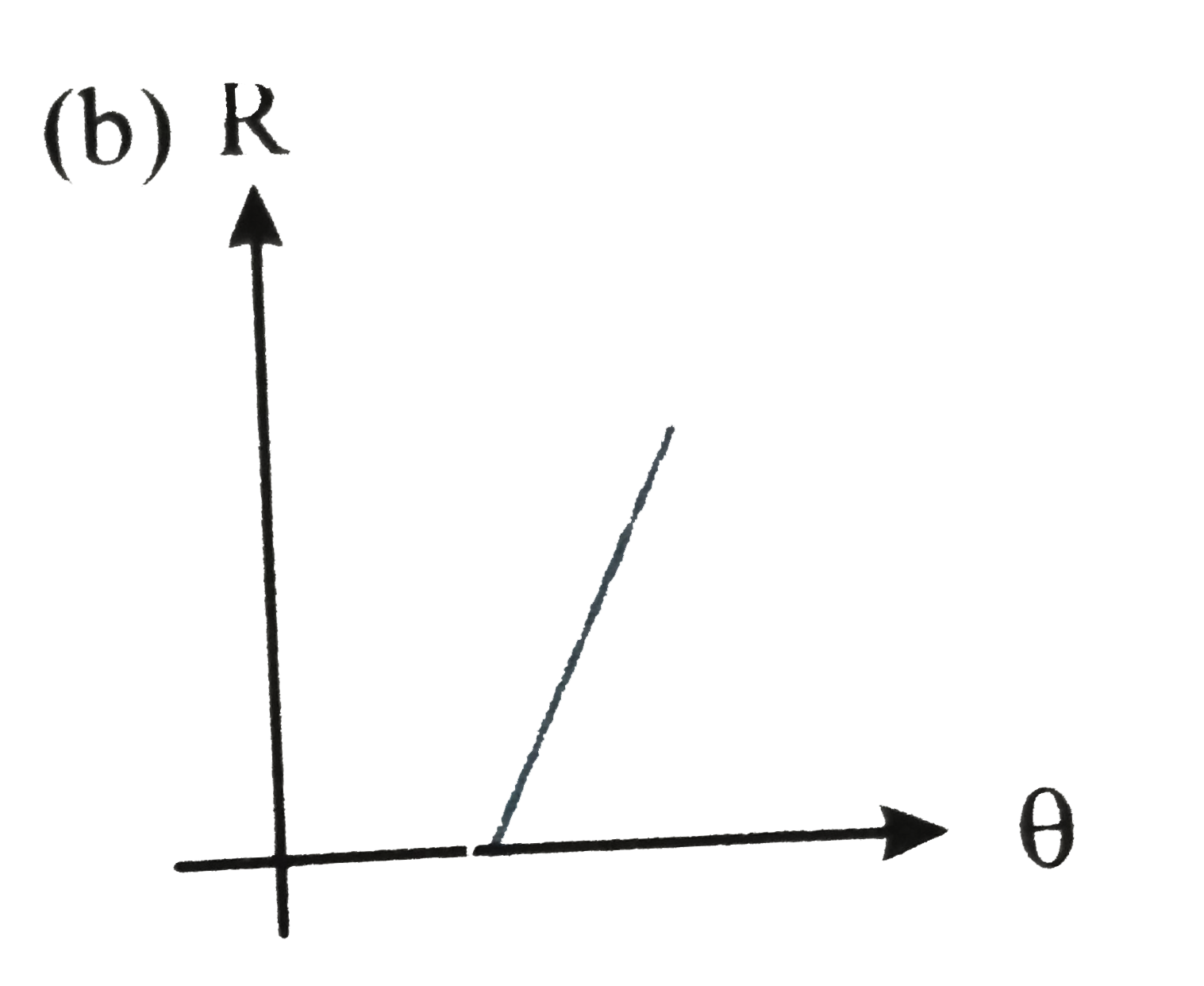 C
C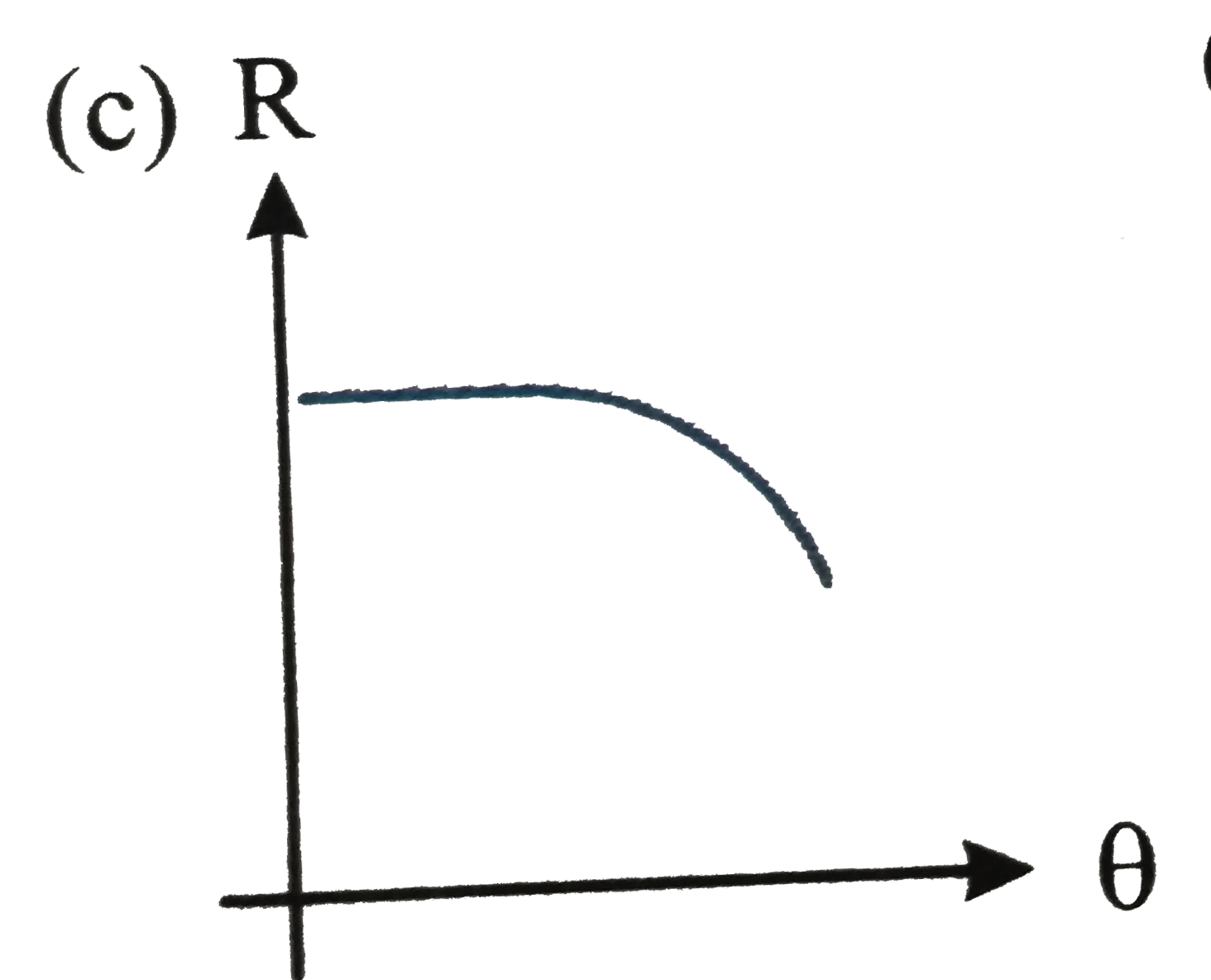 D
D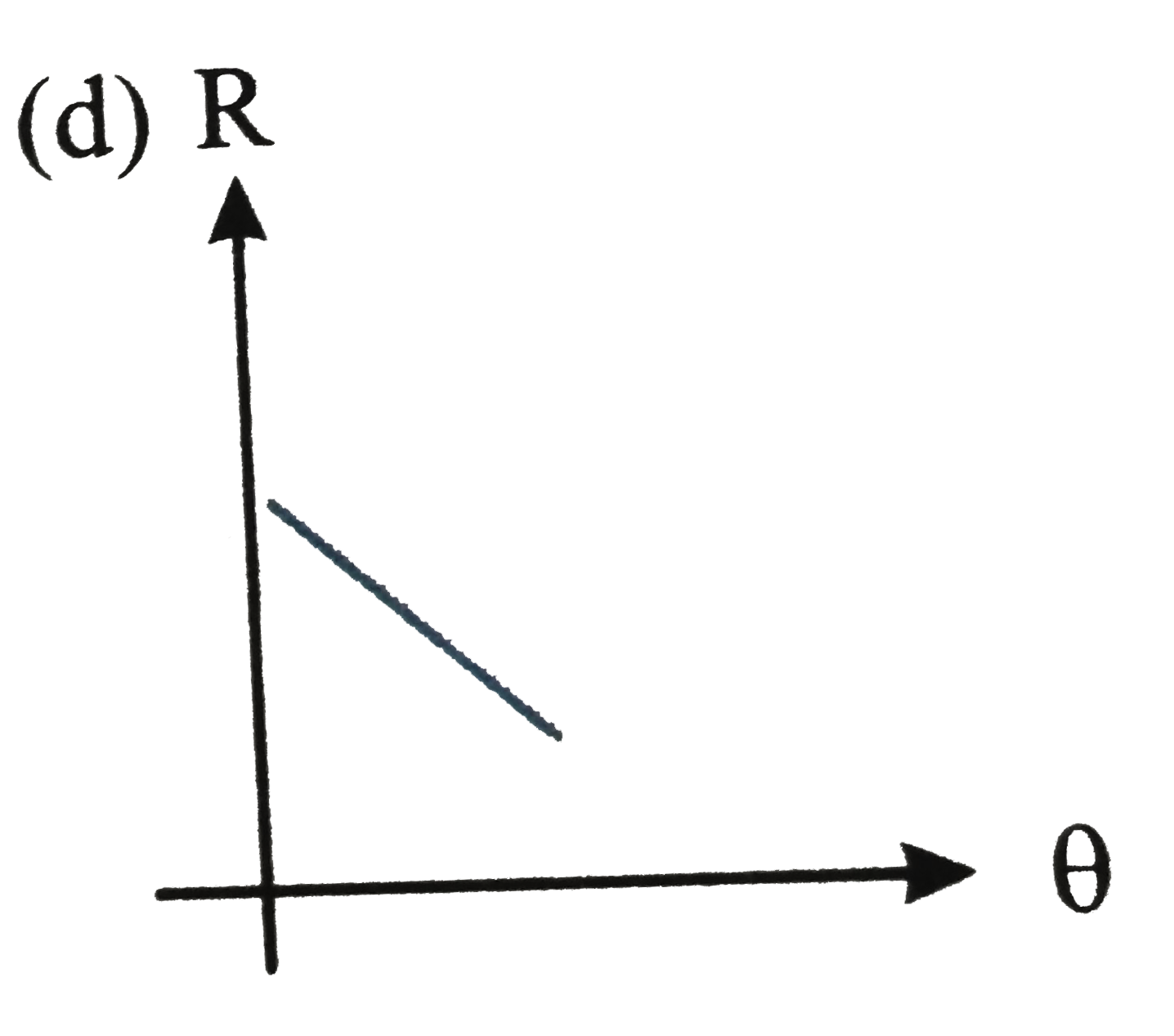
According to Newton's law of cooling, the rate of cooling of a body in air is proportional to the difference between the temperature of the body and the temperature of the surrounding air. If the air temperature is 20∘C and the body cools for 20 minutes from 140∘C→80∘C, then the temperature will be 50∘C in
A30 minutesB40 minutesC50 minutesD60 minutesA liquid in a beaker has temperature θ(t) at time t and θ0 is temperature of surroundings, then according to Newton's law of cooling the correct graph between loge(θ−θ0) and t is :
A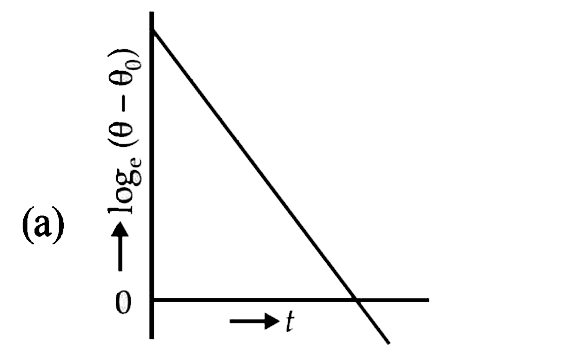 B
B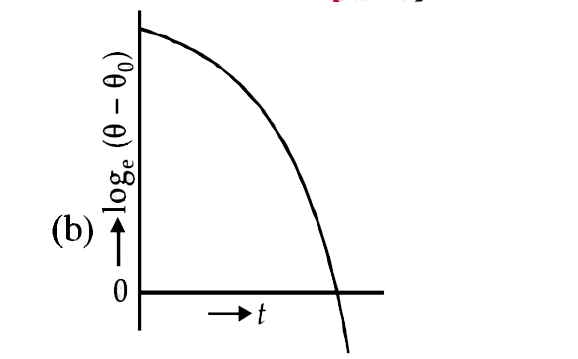 C
C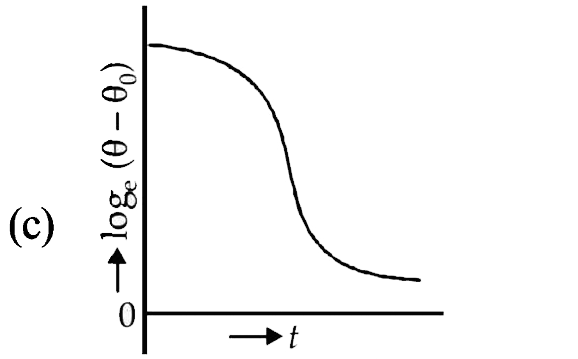 D
D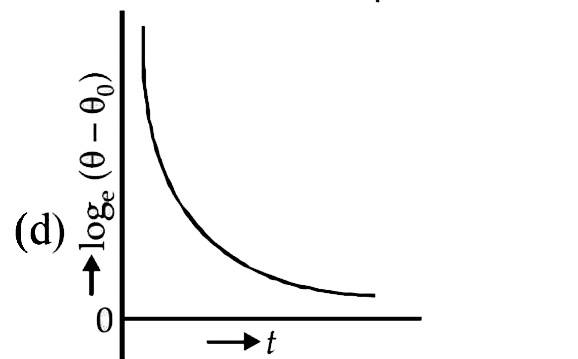
A liquid in a beaker has temperature θ(t) at time t and θ0 is temperature of surroundings, then according to Newton's law of cooling the correct graph between loge(θ−θ0) and t is :
View SolutionTwo bodies A and B of equal masses, area and emissivity cooling under Newton's law of cooling from same temperature are represented by the graph: If θ is the instantaneous temperature of the body and θ0, is the temperature of surroundings, then relationship between their specific heats is
View SolutionA body cools at the rate of 0.5∘C/s when it is 50∘C above the surrounding temperature. The rat of cooling at excess temperature of 30∘C over the surrounding temperature is
View SolutionA block of metal is heated to a temperature of 200∘C which is much higher than the room temperature. It is allowed to cool up to room temperature, in a room which is free from air currents. Its cooling curve (graph of temperature against time) is based on
View SolutionAs difference of temperature of body and surroundings increases, rate of cooling
View Solution
