Themperature of a body θ is slightly more than the temperature of the surrounding θ0 its rate of cooling (R) versus temperature of body (θ) is plotted its shape would be .
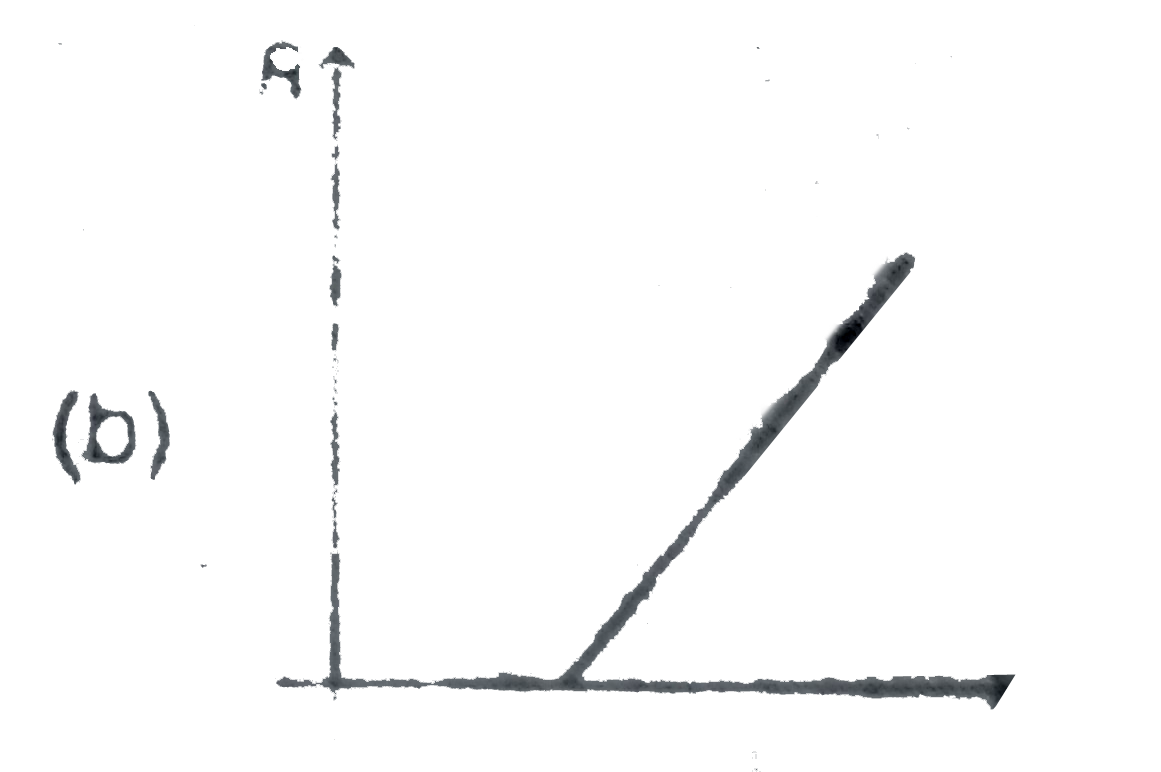

The correct Answer is:B
To solve the problem, we need to analyze the relationship between the rate of cooling (R) and the temperature of the body (θ) when the temperature of the body is slightly more than the temperature of the surroundings (θ₀).
1. Understanding the Concept of Cooling:
- According to Newton's Law of Cooling, the rate of cooling of an object is proportional to the difference in temperature between the object and its surroundings. This can be expressed mathematically as:
dθdt=−k(θ−θ0)
where k is a positive constant, θ is the temperature of the body, and θ0 is the surrounding temperature.
2. Setting Up the Equation:
- Rearranging the equation, we can express the rate of cooling R as:
R=−dθdt=k(θ−θ0)
- Here, R is positive when θ>θ0, indicating that the body is cooling down.
3. Analyzing the Temperature Difference:
- Since it is given that θ is slightly greater than θ0, we can denote this difference as:
Δθ=θ−θ0
- As Δθ approaches zero, the rate of cooling R will also be small but positive.
4. Plotting the Graph:
- The relationship R=k(θ−θ0) indicates that as θ increases, R increases linearly.
- When θ=θ0, R=0. As θ increases beyond θ0, R increases linearly.
5. Conclusion on the Shape of the Graph:
- Therefore, when we plot R (on the y-axis) against θ (on the x-axis), we will get a straight line that starts from the origin (0,0) when θ=θ0 and rises linearly as θ increases.
Final Answer:
The shape of the graph of the rate of cooling R versus the temperature of the body θ is a straight line that passes through the origin and has a positive slope.
- According to Newton's Law of Cooling, the rate of cooling of an object is proportional to the difference in temperature between the object and its surroundings. This can be expressed mathematically as:
dθdt=−k(θ−θ0)
where k is a positive constant, θ is the temperature of the body, and θ0 is the surrounding temperature.
- Rearranging the equation, we can express the rate of cooling R as:
R=−dθdt=k(θ−θ0)
- Here, R is positive when θ>θ0, indicating that the body is cooling down.
- Since it is given that θ is slightly greater than θ0, we can denote this difference as:
Δθ=θ−θ0
- As Δθ approaches zero, the rate of cooling R will also be small but positive.
- The relationship R=k(θ−θ0) indicates that as θ increases, R increases linearly.
- When θ=θ0, R=0. As θ increases beyond θ0, R increases linearly.
- Therefore, when we plot R (on the y-axis) against θ (on the x-axis), we will get a straight line that starts from the origin (0,0) when θ=θ0 and rises linearly as θ increases.
The shape of the graph of the rate of cooling R versus the temperature of the body θ is a straight line that passes through the origin and has a positive slope.
Topper's Solved these Questions
Similar Questions
The temperature of water in calorimeter is θ and the temperature of surrounding is θ0(<θ). Observation are recorded for temperature difference (θ−θ0) and time t. The cooling curve is best represented in graph.
View SolutionA body cools from 50∘C to 40∘C in 5 min. If the temperature of the surrounding is 20∘C , the temperature of the body after the next 5 min would be
View SolutionTwo bodies A and B of equal masses, area and emissivity cooling under Newton's law of cooling from same temperature are represented by the graph: If θ is the instantaneous temperature of the body and θ0, is the temperature of surroundings, then relationship between their specific heats is
View SolutionA body at a temperature of 500F is placed outdoors where the temperature is 1000F . If the rate of change of the temperature of a body is proportional to the temperature difference between the body and its surrounding medium. If after 5 min the temperature of the body is 600F , find (a) how long it will take the body to reach a temperature of 750 F and (b) the temperature of the body after 20 min.
View SolutionA body takes 10 min to cool douwn from 62∘C to 50∘C. If the temperature of surrounding is 26∘C then in the next 10 minutes temperature of the body will be
View SolutionIf a body cools down from 80∘Cto 60∘C in 10 min when the temperature of the surrounding of the is 30∘C . Then, the temperature of the body after next 10 min will be
View SolutionA liquid in a beaker has temperature θ(t) at time t and θ0 is temperature of surroundings, then according to Newton's law of cooling the correct graph between loge(θ−θ0) and t is :
View SolutionFor a black body at temperature 727∘C, its radiating power is 60 watt and temperature of surrounding is 227∘C. If temperature of black body is changed to 1227∘C then its radiating power will be-
View SolutionThe correct graph between the temperature of a hot body kept in cooler surrounding and time is (Assume newton's law of cooling)
View SolutionA body with an initial temperature θ1 is allowed to cool in a surrounding which is at a constant temperature of θ0(θ<θ1) Assume that Newton's law of cooling is obeyed Let k= constant The temperature of the body after time t is best experssed by .
View Solution
