In a certain oscillatory system (particle is performing SHM), the amplitude of motion is 5 m and the time period is 4 s. the minimum time taken by the particle for passing betweens points, which are at distances of 4 m and 3 m from the centre and on the same side of it will approximately be
1645s
745s
845s
1345s
The correct Answer is:C
To solve the problem, we need to find the minimum time taken by a particle performing Simple Harmonic Motion (SHM) to travel between two points at distances of 4 m and 3 m from the center of motion. Here’s a step-by-step solution:
Step 1: Understand the Parameters
Given:
- Amplitude (A) = 5 m
- Time period (T) = 4 s
Step 2: Calculate the Angular Frequency
The angular frequency (ω) can be calculated using the formula:
ω=2πT
Substituting the given time period:
ω=2π4=π2 rad/s
Step 3: Set Up the Equations for the Distances
The displacement in SHM is given by:
x=Asin(ωt)
For the first position (x1 = 3 m):
3=5sin(ωt1)⟹sin(ωt1)=35
For the second position (x2 = 4 m):
4=5sin(ωt2)⟹sin(ωt2)=45
Step 4: Calculate Time for Each Position
Using the inverse sine function:
1. For t1:
ωt1=sin−1(35)
2. For t2:
ωt2=sin−1(45)
Step 5: Find the Time Difference
The time difference t2−t1 can be expressed as:
t2−t1=1ω(sin−1(45)−sin−1(35))
Step 6: Calculate sin−1(35) and sin−1(45)
Using a calculator:
- sin−1(35)≈0.6435 radians
- sin−1(45)≈0.9273 radians
Step 7: Substitute Values
Now substitute these values into the time difference equation:
t2−t1=1π2(0.9273−0.6435)
Calculating the difference:
0.9273−0.6435=0.2838 radians
Thus,
t2−t1=2π×0.2838
Step 8: Final Calculation
Calculating the time difference:
t2−t1≈2×0.28383.1416≈0.180 seconds
Conclusion
The minimum time taken by the particle to pass between the points at distances of 4 m and 3 m from the center is approximately 0.180 seconds.
Given:
- Amplitude (A) = 5 m
- Time period (T) = 4 s
The angular frequency (ω) can be calculated using the formula:
ω=2πT
Substituting the given time period:
ω=2π4=π2 rad/s
The displacement in SHM is given by:
x=Asin(ωt)
For the first position (x1 = 3 m):
3=5sin(ωt1)⟹sin(ωt1)=35
For the second position (x2 = 4 m):
4=5sin(ωt2)⟹sin(ωt2)=45
Using the inverse sine function:
1. For t1:
ωt1=sin−1(35)
2. For t2:
ωt2=sin−1(45)
The time difference t2−t1 can be expressed as:
t2−t1=1ω(sin−1(45)−sin−1(35))
Using a calculator:
- sin−1(35)≈0.6435 radians
- sin−1(45)≈0.9273 radians
Now substitute these values into the time difference equation:
t2−t1=1π2(0.9273−0.6435)
Calculating the difference:
0.9273−0.6435=0.2838 radians
Thus,
t2−t1=2π×0.2838
Calculating the time difference:
t2−t1≈2×0.28383.1416≈0.180 seconds
The minimum time taken by the particle to pass between the points at distances of 4 m and 3 m from the center is approximately 0.180 seconds.
Topper's Solved these Questions
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Multiple Correct
35 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Assertion Reasoning
6 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Subjective
21 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Interger
11 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type
3 Videos
Similar Questions
A particle is performing SHM of amplitude 'A' and time period 'T'. Find the time taken by the particle to go from 0→A/2.
View SolutionA particle performs SHM on x- axis with amplitude A and time period period T .The time taken by the particle to travel a distance A/5 string from rest is
View SolutionA particle performs SHM on x- axis with amplitude A and time period period T .The time taken by the particle to travel a distance A/5 starting from rest is
View SolutionA particle is performing simple harmonic motion on a straight line with time period 12 second. The minimum time taken by particle to cover distance equal to amplitude will be
View SolutionDefine amplitude of S.H.M. ? Does Amplitude affect the time period ?
View SolutionA particle executes SHM with amplitude of 20 cm and time period of 12 s. What is the minimum time required for it to move between two points 10 cm on either side of the mean position?
View SolutionA particle is performing SHM about mean position O as shown in the figure given below. Its amplitude is 5 m and time period of oscillation is 4 s. The minimum time taken by the particle to move from point A to B is nearly
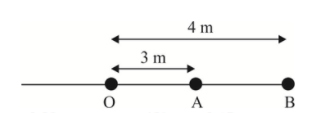
View SolutionFor a particle performing SHM, equation of motion is given as d2dt2+4x=0. Find the time period
View SolutionThe maximum velocity of a particle, executing SHM with an amplitude 7 mm is 4.4 m/s. the period of oscillation is
View SolutionA particle executes a simple harmonic motion of time period T. Find the time taken by the particle to go directly from its mean position to half the amplitude.
View Solution
