In a circuit shown in figure the battery emf is equal to E=2.0V, its internal resistance is r=9.0Ω, the capacitance of the capacitor is C=10μF, the coil inductance is L=100mH, and the resistance is R=100Ω . At a certain momenta the switch Sw was disconneted.
Find the energy of oscillations in the circuit
(b) t=0.30s after the switch was disconnected,
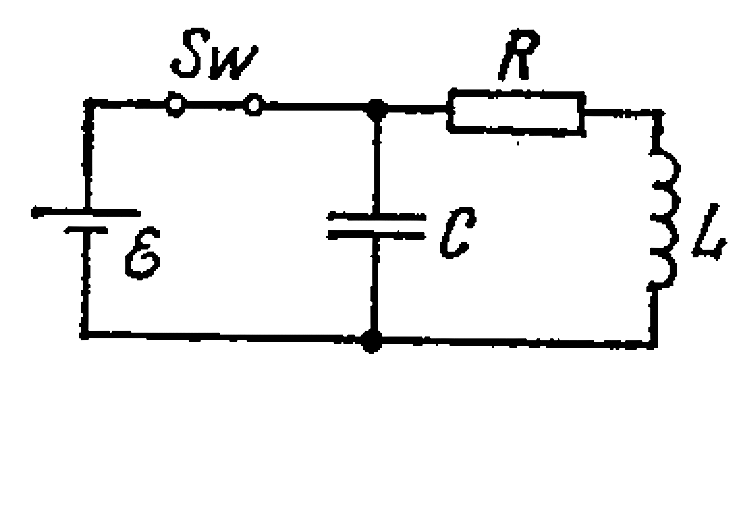
At t=0 current throught the coil =εR+r
P.D. across the condenser =εR+r
(a) At t=0, energy stroed=W0
=12L(εR+r)2+12C(εR+r)2=12ε2(L+CR2)(R+r)=20mJ
(b) The current and the change stores decrease as e−tR/2L so energy decreases as e−tR/L
∴W=W0e−tR/L=0.10mJ.

Topper's Solved these Questions
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Elastic Waves
39 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Exercise
2 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
Similar Questions
A coil of inductance 1.0H and resistance 100Ω is connected to a battery of emf 12V. Find the energy stored in the magnetic field associated with the coil at an instant 10ms after the circuit is switched on.
View SolutionA resistor is connected to a battery of emf 10 V and internal resistance 0.3Ω. What is the resistance of the resistor to be inserted in the circuit for the circuit current 1.2A?
View Solution
Knowledge Check
In the circuit shown in figure switch S is closed at time t=0
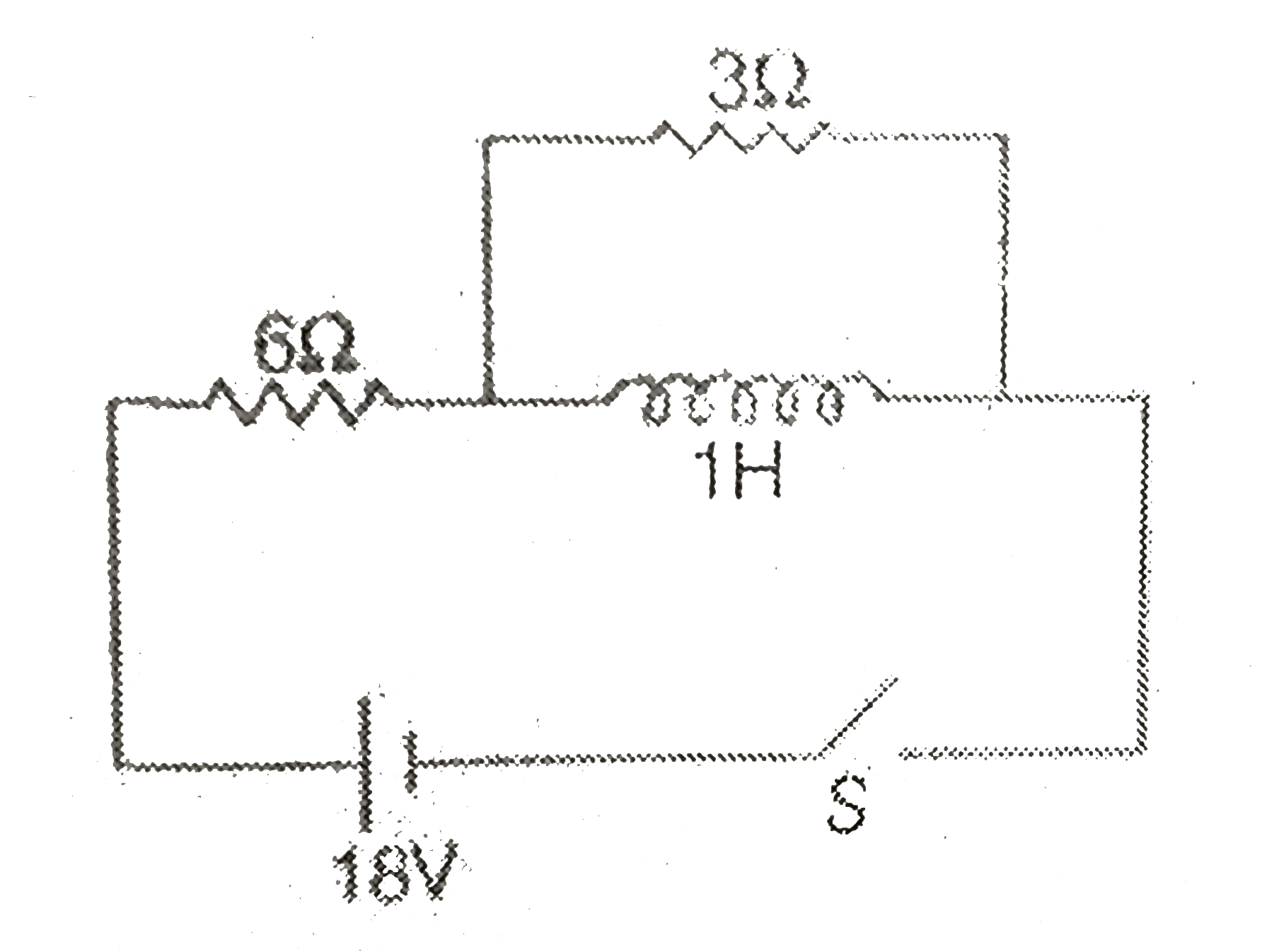
At what time current through 3Ω resistance and 1H inductor are equal?AIn√53BIn(83)CIn(53)DIn√83A coil of inductance 1.0 H and resistance 100 ohm is connected to a battery of emf 12 V. Find the energy stored in the magnetic field of the coil 10 ms after the circuit is switched on.
A2.88 mJB2.53 mJC1.59 mJD1.75 mJAn uncharged capacitor of capacitance 100μF is connected to a battery of emf 20V at t = 0 through a resistance 10Ω, then
(i) the maximum rate at which energy is stored in the capacitor is :A10 J/sB20 J/sC40 J/sD5 J/s
An oscillating circuit consist of a capacitor with capacitance C=4.0μF and a coil with inductance L=2.0μH and active resistance R=10Ω. Find the ration of the energy of the coil's magnetic field to that of the capacitor's electric field at the moment when the current has the maximum value .
View SolutionAn oscillating circuuit incorporates a leaking capacitor. Its capacitance is equal C and active resistance to R. The coil inductance is is L. The resistance of the coil and the wires is negligible. Find :
(a) the damped oscillation frequecy of such a circuit ,
(b) its quality factor.
View SolutionA 20(μ)Fcapacitor is joined to a battery of emf 6.0V through a resistance of 100(Ω).find the charge on the capacitor 2.0ms after the connections are made .
View SolutionIn an oscillating circuit shown in figure, the coil inductance is equal to L=2.5μF. The capacitor have capacitances C1=2.0μF and C2=3.0μF. The capacitors were charged to a voltage V=180V, and then the switch Swwas closed. Find :
(a) the natural oscillation frequency,
(b) the peak value of the current flowing through the coil.
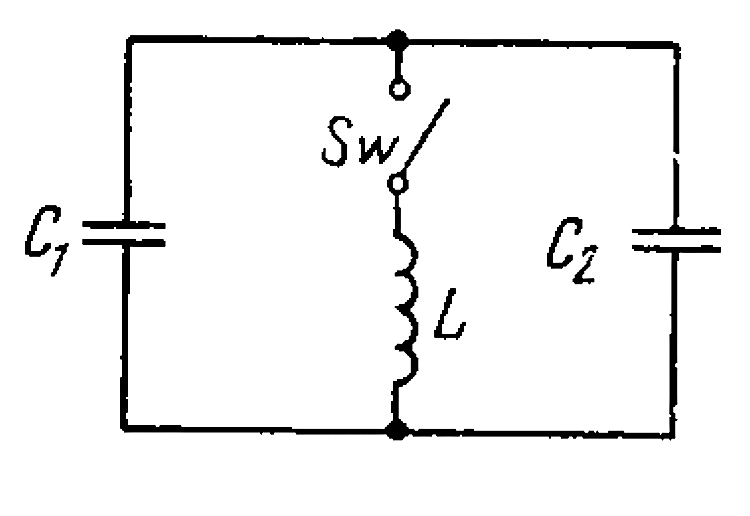
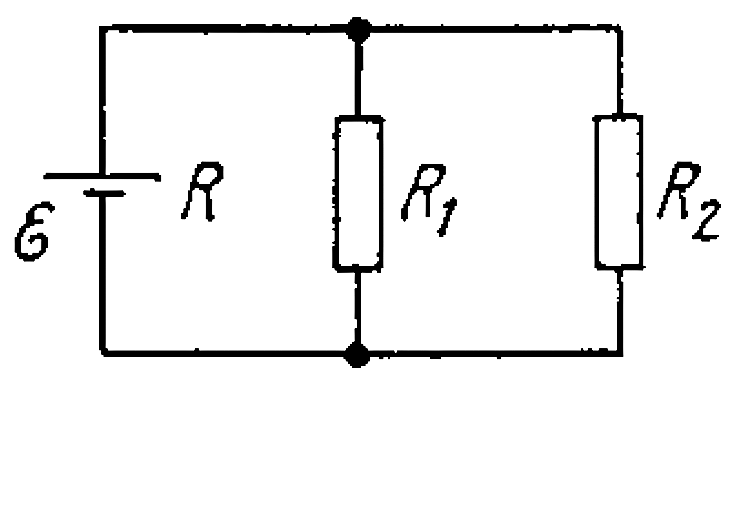
View SolutionIn the circuit shown in Fig. the emf of the sources is equal to ξ=5.0V and the resistances are equal to R1=4.0Ω and R2=6.0Ω. The internal resistance of the source equals R=1.10Ω. Find the currents flowing through the resistances R1 and R2.
View Solution
