In a coaxial, straight cable, the central conductor and the outer conductor carry equal currents in opposite directions. The magnetic field is zero.
outside the cable
inside the inner conductor
inside the outer conductor
in between the two conductor
The correct Answer is:A
To solve the problem regarding the magnetic field in a coaxial cable with equal currents in opposite directions, we can follow these steps:
1. Understand the Configuration:
- We have a coaxial cable consisting of a central conductor and an outer conductor. The central conductor carries a current I in one direction, while the outer conductor carries an equal current I in the opposite direction.
2. Apply Ampere's Law:
- According to Ampere's Law, the magnetic field B around a current-carrying conductor can be calculated using the formula:
∮B⋅dl=μ0Ienc
- Here, Ienc is the current enclosed by the Amperian loop.
3. Choose an Amperian Loop:
- We can consider different Amperian loops: one inside the inner conductor, one in the space between the inner and outer conductors, and one outside the outer conductor.
4. Evaluate the Magnetic Field in Different Regions:
- Inside the Inner Conductor:
- The magnetic field B is zero because there is no current enclosed by the loop.
- Between the Inner and Outer Conductor:
- The enclosed current is I−I=0 (since the currents are equal and opposite), thus:
B=0
- Outside the Outer Conductor:
- The enclosed current is also zero because the currents cancel each other out, leading to:
B=0
5. Conclusion:
- Since the magnetic field is zero in all regions (inside the inner conductor, between the conductors, and outside the outer conductor), we conclude that the magnetic field is indeed zero in the entire coaxial cable setup.
Final Answer:
The magnetic field is zero in all regions: inside the inner conductor, between the two conductors, and outside the outer conductor.
---
- We have a coaxial cable consisting of a central conductor and an outer conductor. The central conductor carries a current I in one direction, while the outer conductor carries an equal current I in the opposite direction.
- According to Ampere's Law, the magnetic field B around a current-carrying conductor can be calculated using the formula:
∮B⋅dl=μ0Ienc
- Here, Ienc is the current enclosed by the Amperian loop.
- We can consider different Amperian loops: one inside the inner conductor, one in the space between the inner and outer conductors, and one outside the outer conductor.
- Inside the Inner Conductor:
- The magnetic field B is zero because there is no current enclosed by the loop.
- Between the Inner and Outer Conductor:
- The enclosed current is I−I=0 (since the currents are equal and opposite), thus:
B=0
- Outside the Outer Conductor:
- The enclosed current is also zero because the currents cancel each other out, leading to:
B=0
- Since the magnetic field is zero in all regions (inside the inner conductor, between the conductors, and outside the outer conductor), we conclude that the magnetic field is indeed zero in the entire coaxial cable setup.
The magnetic field is zero in all regions: inside the inner conductor, between the two conductors, and outside the outer conductor.
Topper's Solved these Questions
- MAGNETIC FIELD DUE TO CURRENTBOOK - HC VERMACHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - Exercises
61 Videos
- MAGNETIC FIELD DUE TO CURRENTBOOK - HC VERMACHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - Objective 1
12 Videos
- MAGNETIC FIELDBOOK - HC VERMACHAPTER - MAGNETIC FIELDEXERCISE - Exercises
61 Videos
- MAGNETIC PROPERTIES OF MATTERBOOK - HC VERMACHAPTER - MAGNETIC PROPERTIES OF MATTEREXERCISE - Exercises
9 Videos
Similar Questions
A coaxial cable carries the a current i in the inside conductor of radius a as shown in figure-4.80 and the outer conductor of inner radius b and outer radius c carries a current i' in opposite direction. Find the magnetic induction due to the coaxial cable at distance r from the central axis of the cable for (a) r>a, (b) a<r<b and (c) b>r>c

View SolutionThere is one long coaxial cable which consists of two concentric cylinders of radii a and b. Central conductor carries steady current I and outer conductor acts as return path for the current. (i) Calculate energy stored in the magnetic field of length l of such a cable. (ii) Calculate self-inductance for l length of this cable.
View Solution
Knowledge Check
In a coaxial, straght cable, the central conductor and the outer conductor carry equal currents in opposite directions.The magnetic field is non-zero.
 Aoutside the cableBinside the inner conductor except axis of the conductorCall the point inside the outer conductor and outside inner conductorDonly on axis of conductors
Aoutside the cableBinside the inner conductor except axis of the conductorCall the point inside the outer conductor and outside inner conductorDonly on axis of conductors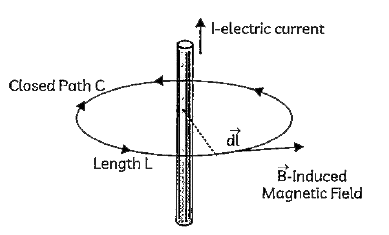
If two conductors carry current in opposite direction, then:Athere is no force between themBforce is repulsiveCforce is attractiveDindependent of forceA coaxial cable consists of a thin inner conductor fixed along the axis of a hollow outer conductor. The two conductor carry equal currents in opposite directions. Let B1andB2 be the magnetic fields in the region between the conductors and outside the conductor, respectively. Then,
AB1≠0,B2≠0BB1=B2=0CB1≠0,B2=0DB1=0,B2≠0
Two long straight conductors are held parallel to each other 7cm apart. The conductors carry currents of 9A and 16A in opposite directions. The distance of neutral point from the conductor carrying 16A current is
View SolutionThe two linear parallel conductors carrying currents in the opposite direction…………………each other.
View SolutionA coaxial cable made up of two conductors. The inner conductor is solid and is of radius R1 and the outer conductor is hollow of inner radius R2 and outer radius R3. The space between the conductors is filled with air. The inner and outer conductors are carrying currents of equal magnitudes and in opposite directions. Then the variation of magnetic field with distance from the axis is best plotted as
View SolutionThe direction of force on a current carrying conductor in a magnetic field is given by
View SolutionMagnetic lines of force due to a straight conductor carrying current are
View Solution