There is one long coaxial cable which consists of two concentric cylinders of radii a and b. Central conductor carries steady current I and outer conductor acts as return path for the current. (i) Calculate energy stored in the magnetic field of length l of such a cable. (ii) Calculate self-inductance for l length of this cable.
(i) Magnetic field intensity at a point distance r from the centre of the cable is given by
B=μ0I2πr
Energy density in terms of distance r can be written as follows:
u=B22μ0
⇒u=(μ0I2πr)22μ0
⇒u=μ0I28π2r2

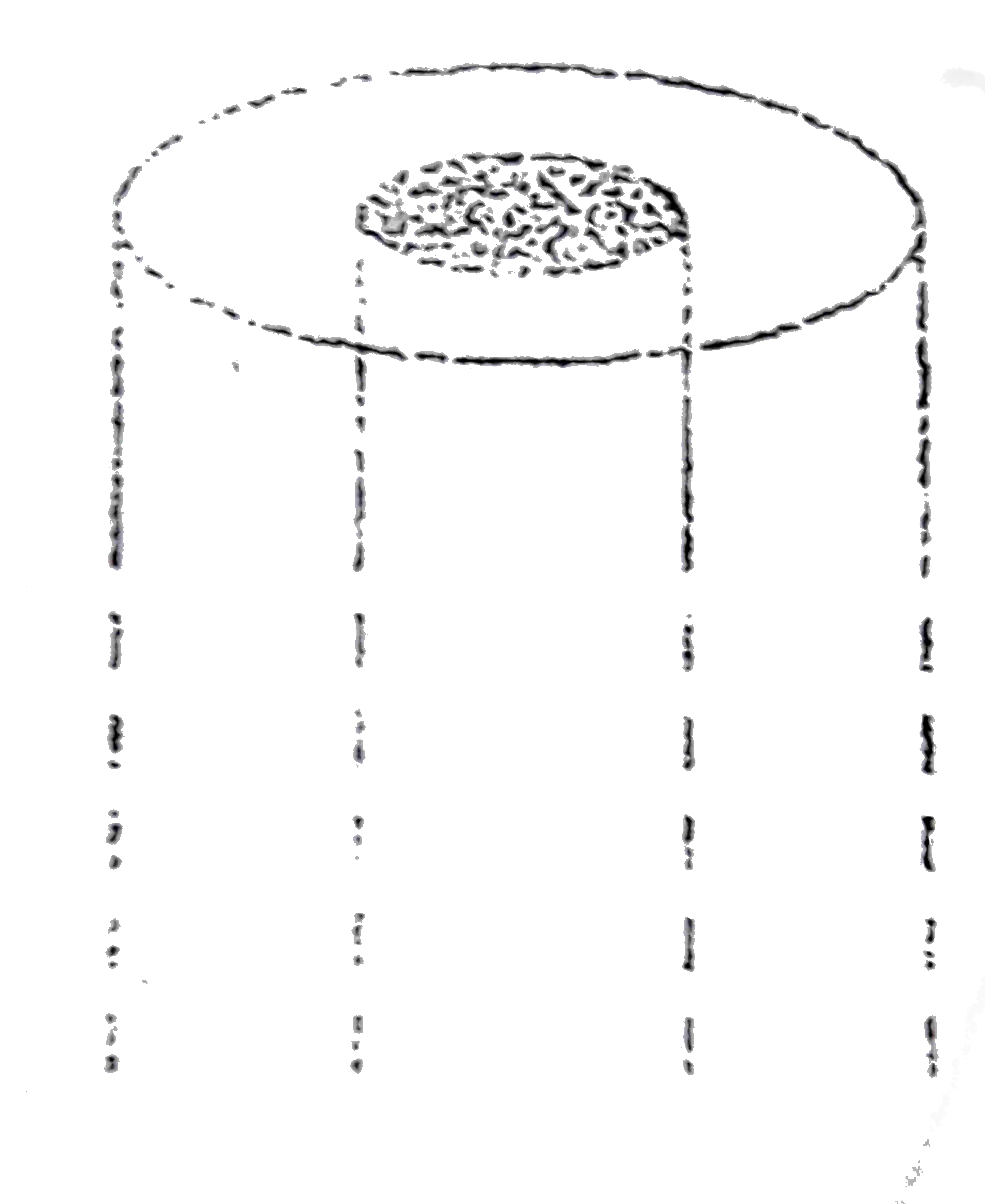
Consider a volume segment dV which is in the form of a cylindrical shell of radii r and r + dr as shown in the figure. Length of this cylindrical shell is l which is perpendicular to the plane shown in figure. Energy density can be assumed to be constant throughout this segment and hence energy stored in this segment can be written as follows:
Energy dU = udV
dU=μ0I28π2r22πrldr
dU=μ0I2l4πdrr
Total magnetic energy can be calculated by integrating above relation.
U=μ0I2l4π∫badrr
U=μ0I2l4π∫badrr
U=μ0I2l4πInba
(ii) We can use above calculated value of energy stored to calculate self-inductance of this cable. We know that energy stored in an inductor is given as follows:
U=12LI2
We can equate it with value calculated in part (i)
12LI2=μ0I2l4πInba
L=μ0l2πInba
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - REVISION EXERCISE (Very Short Answer Questions Carrying I mark)
44 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - REVISION EXERCISE (Additional Questions (Carrying I mark))
11 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - NCERT FILE (NCERT EXEMPLAR PROBLEMS SUBJECTIVE QUESTIONS) (Short answer type questions)
5 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Chapter Practice Test
15 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
Similar Questions
A long coaxial cable consists of two concentric cylinders of radii a and b. The central conductor of the cable carries a steady current I and the outer conductor provides the return path of the current. Calculate the energy stored in the magnetic field of length l of such a cable
View Solutiona long coaxial cable consists of two thin-walled conducting cylinder carries a steady current 0.1A, and the outer cylinder provides the return path for that current. The current produced a magnetic field between the two cylinders. Find the energy stored in the magnetic field for length 5m of the cable. Express answer in Nj (use 1n2=0.7).
View Solution
Knowledge Check
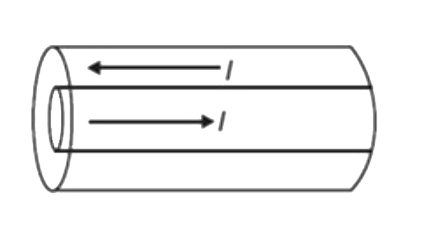
In a coaxial, straght cable, the central conductor and the outer conductor carry equal currents in opposite directions.The magnetic field is non-zero.
 Aoutside the cableBinside the inner conductor except axis of the conductorCall the point inside the outer conductor and outside inner conductorDonly on axis of conductors
Aoutside the cableBinside the inner conductor except axis of the conductorCall the point inside the outer conductor and outside inner conductorDonly on axis of conductorsThe figure shows a long coaxial cable in which a current I flows down through the inner cylinder of radius a and the same current flows back up through the outer cylinder of radius 2a. The cylinders are insulated from each other and the current is uniformly distributed over the area of the cross-section in each cylinder. The strength of the magnetic field at a distance 3a/2 from the axis of the cable is
 AB=5μ0I36πaBB=5μ0I12πaCB=7μ0I12πaDB=7μ0I36πa
AB=5μ0I36πaBB=5μ0I12πaCB=7μ0I12πaDB=7μ0I36πa
a long coaxial cable consits of two thin-walled conducting cyclider carries a steady current 0.1A, and the outer cylinder provides the return path for that current. The current produced a magnetic field between the two cylinders. Find the energy stored in the magnetic field for length 5m of the cable. Express answer in Nj (use 1n2=0.7).
View SolutionIn a coaxial, straight cable, the central conductor and the outer conductor carry equal currents in opposite directions. The magnetic field is zero.
View SolutionIn a coaxial, straight cable, the central conductor and the outer conductor carry equal currents in opposite directions. The magnetic field is zero.
View SolutionA long solid cylindrical straight conductor carries a current i0 . Find the magnetic energy stored per meter inside it.
View SolutionConsider a co axial cable which consists of an inner wilre of radius a surrounded by an outer shell of inner and outer radii b and c respectively. The inner wire caries a current I and outer shell carries an equal and opposite current. The magnetic field at a distance x from the axis where b<x<c is
View SolutionThe figure shows a long coaxial cable in which a current I flows down through the inner cylinder of radius a and the same current flows back up through the outer cylinder of radius 2a. The cylinders are insulated from each other and the current is uniformly distributed over the area of the cross-section in each cylinder. The strength of the magnetic field at a distance 3a/2 from the axis of the cable is

View Solution
