In a football game a kicker attempts a field goal. The ball remains in contact with the kicker's foot for 0.050 s, during which time it experiences an acceleration of . The ball is launched at an angle of above the ground. Determine the horizontal and vertical components of the launch velocity.
The correct Answer is:C
To solve the problem, we will break it down into steps:
Step 1: Calculate the final velocity of the ball
We know that the ball experiences a constant acceleration while in contact with the kicker's foot. We can use the formula for final velocity under constant acceleration:
v=u+at
Where:
- v = final velocity
- u = initial velocity (which is 0 since the ball starts from rest)
- a = acceleration (given as 340m/s2)
- t = time of contact (given as 0.050s)
Substituting the values:
v=0+(340m/s2)(0.050s)=17m/s
Step 2: Resolve the final velocity into horizontal and vertical components
The final velocity of the ball can be resolved into horizontal and vertical components using the angle of launch. The angle given is 51∘.
The horizontal component vx is given by:
vx=v⋅cos(θ)
And the vertical component vy is given by:
vy=v⋅sin(θ)
Where:
- θ=51∘
- v=17m/s
Calculating vx:
vx=17⋅cos(51∘)≈17⋅0.6293≈10.69m/s
Calculating vy:
vy=17⋅sin(51∘)≈17⋅0.7771≈13.21m/s
Step 3: State the final components of the launch velocity
Thus, the horizontal and vertical components of the launch velocity are:
- Horizontal component vx≈10.69m/s
- Vertical component vy≈13.21m/s
Final Answer
- Horizontal component vx≈10.69m/s
- Vertical component vy≈13.21m/s
---
We know that the ball experiences a constant acceleration while in contact with the kicker's foot. We can use the formula for final velocity under constant acceleration:
- v = final velocity
- u = initial velocity (which is 0 since the ball starts from rest)
- a = acceleration (given as 340m/s2)
- t = time of contact (given as 0.050s)
The final velocity of the ball can be resolved into horizontal and vertical components using the angle of launch. The angle given is 51∘.
- θ=51∘
- v=17m/s
Thus, the horizontal and vertical components of the launch velocity are:
- Vertical component vy≈13.21m/s
- Horizontal component vx≈10.69m/s
- Vertical component vy≈13.21m/s
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - PRACTICE QUESTIONS (MORE THAN ONE CORRECT CHOICE TYPE)3videos
MOTION IN TWO AND THREE DIMENSIONS
- BOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - PRACTICE QUESTIONS (LINKED COMPREHENSION)14videos
MOTION IN TWO AND THREE DIMENSIONS
- BOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - PROBLEMS50videos
MOTION IN TWO AND THREE DIMENSIONS
- BOOK - RESNICK AND HALLIDAYCHAPTER - MOTION ALONG A STRAIGHT LINEEXERCISE - PRACTICE QUESTIONS ( Integer Type )3videos
MOTION ALONG A STRAIGHT LINE
- BOOK - RESNICK AND HALLIDAYCHAPTER - OSCILLATIONSEXERCISE - Practice Questions57videos
OSCILLATIONS
Similar Questions
On the surface of the moon , a cricket ball is hit at some angle with the horizontal . As the ball traveles through vecuume , determine which of the quantities will not change? (a) speed , (b) acceleration , (c) horizontal and vertical components of velocity and (d) resultant velocity .
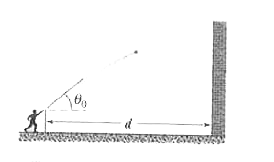
View SolutionYou throw a ball toward a wall at speed 25.0 m/s and at angle θ0=40.0∘ above the horizontal. The wall is distance d = 22.0 m from the release point of the ball. (a) How far above the release point does the ball hit the wall? What are the (b) horizontal and ( c) vertical components of its velocity as it hits the wall? (d) When it hits, has it passed the highest point on its trajectory?
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A man in a balloon, rising vertically with an acceleration of 5m/s2, releases a ball 10 s after the balloon is let go from the ground. The greatest height above the ground reached by the ball is
A125 mB250 mC375 mD500 m - Question 2 - Select One
A player in the football team tries to kick a football so that it stays in the air for a long "hang time". If the ball is kicked with an initial velocity of 25.0 m/s at an angle of 60.0∘ above the ground, what is the "hang time"?
A4.42 sB3.36 sC2.21 sD1.68 s - Question 3 - Select One
A ball is dropped on the floor from a height of 10m. It rebounds to a height of 2.5 m if the ball is in contact with floor for 0.01 s then the average acceleration during contact is nearly
A500√2m/s2 upwardsB1800√2m/s2 downwardsC1500√2m/s2 upwardsD1500√2m/s2 downwards
A football player kicks a ball at ball at an angle of 300 with the horizontal with an initial speed of 20m/s. Assuming that the ball travels in a vertical plane, calculate (a) the time at which the ball reaches the highest point (b) maximum height reached (c ) the horizontal range of the ball (d) the time for which the ball is in air. g=10m/s2.
View SolutionA volleyball is spiked so that it has an initial velocity of 15 m/s directed downward at an angle of 55∘ below the horizontal. What is the horizontal component of the ball's velocity when the opposing player fields the ball?
View SolutionA tennis ball is dropped on the floor from a height of 20m. It rebounds to a height of 5m. If the ball was in contact with the floor for 0.01s. What was its average acceleration during contact? (g=10m/s2)
View SolutionA football player kicks a 0.41 kg football initially at rest, and the ball flies through the air. If the kicker's foot was in contact with the ball for 0.051 s and the ball's initial speed after the collision is 21 m/s, what was the magnitude of the average force on the football?
View SolutionA boy throws a ball upward with velocity v0=20m/s. The wind imparts a horizontal acceleration of 4m/s2 to the left. The angle θ at which the ball must be thrown so that the ball returns to the boy's hand is : (g=10ms−2)
View Solution

