In a hydrogen atom, the radius of $n^{t h}$ bohr orbit is $r_{n}$ . The graph between log $(r_{n} / r_{1})$ and logn will be

A

ocr_image

B

ocr_image

C

ocr_image

D

ocr_image

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:A

To find the graph between log $(r_{n} / r_{1})$ and log $n$ for a hydrogen atom, we can follow these steps:
Step 1: Understand the relationship between the radius of the nth orbit and the first orbit
The radius of the nth orbit $r_{n}$ in a hydrogen atom is given by the formula:
$r_{n} = n^{2} r_{1}$
where $r_{1}$ is the radius of the first orbit.
Step 2: Express $r_{n} / r_{1}$
From the formula above, we can express the ratio of the radii:
$\frac{r_{n}}{r_{1}} = n^{2}$
Step 3: Take the logarithm of both sides
Now, we take the logarithm of both sides of the equation:
$log (\frac{r_{n}}{r_{1}}) = log (n^{2})$
Step 4: Apply the logarithmic identity
Using the logarithmic identity $log (a^{b}) = b \cdot log (a)$ , we can simplify the right side:
$log (\frac{r_{n}}{r_{1}}) = 2 \cdot log (n)$
Step 5: Rearranging the equation
This can be rearranged to show the relationship between $log (\frac{r_{n}}{r_{1}})$ and $log (n)$ :
$log (\frac{r_{n}}{r_{1}}) = 2 log (n)$
Step 6: Identify the graph characteristics
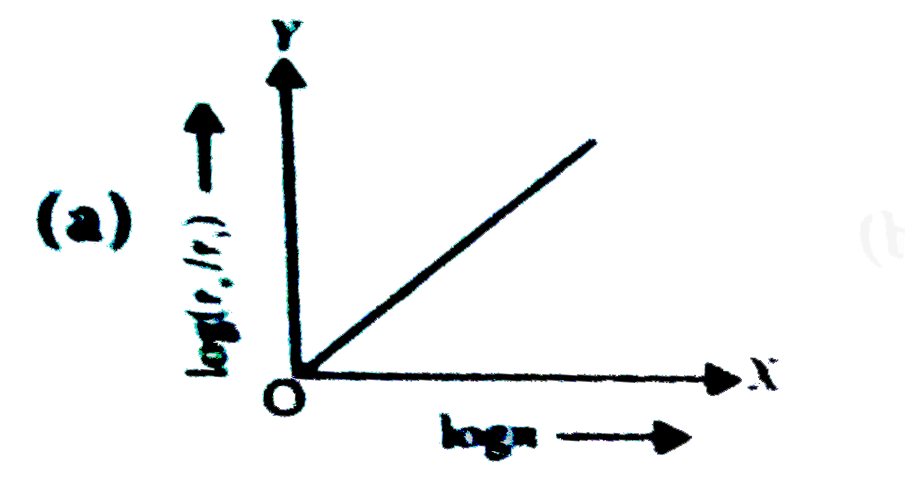
This equation is in the form of $y = m x$ , where $y = log (\frac{r_{n}}{r_{1}})$ , $x = log (n)$ , and $m = 2$ . This indicates that the graph will be a straight line passing through the origin with a slope of 2.
Conclusion
Thus, the graph between $log (\frac{r_{n}}{r_{1}})$ and $log (n)$ is a straight line passing through the origin with a slope of 2.
---

|

Updated on:7/8/2024

Class 12 PHYSICS ATOMS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
In Bohr.s model of hydrogen atom, radius of the first orbit of an electron is $r_{0}$ . Then, radius of the third orbit is:
A $\frac{r_{0}}{9}$
B $r_{0}$
C $3 r_{0}$
D $9 r_{0}$
Question 2 - Select One
From quantisation of angular momentum one gets for hydrogen atom, the radius of the $n^{t} h$ orbit as $r_{n} = (\frac{n^{2}}{m_{e}}) {(\frac{h}{2 π})}^{2} (\frac{4 π ε_{0}}{e^{2}})$
For a hydrogen like atom of atomic number Z,
Athe radius of the first orbit will be the same
B $r_{n}$ will be greater for larger Z values
C $r_{n}$ will be smaller for larger Z values
Dnone of these
Question 3 - Select One
Suppose an electron is attracted toward the origin by a force $\frac{k}{r}$ where $k$ is a constant and $r$ is the distance of the electron from the origin .By applying Bohr model to this system the radius of the $n^{t h}$ orbital of the electron is found to be $r_{n}$ and the kinetic energy of the electron to be $T_{n}$ , Then which of the following is true ?
A $T_{n} \propto \frac{1}{n^{2}}$
B $T_{n}$ is independent of n, $r_{n} \propto n$
C $T_{n} \propto \frac{1}{n}$ and $r_{n}$
D $T_{n} \propto \frac{1}{n}$ and $r_{n} \propto n^{2}$

NCERT FINGERTPS ENGLISH-ATOMS -Assertion And Reason

Home
Ask Doubt
Profile