In a one-dimensional collision between two identical particles. A and B, B is stationary and A has momentum before impact. During impact, B gives an impulse J to A. Find the coefficient of restitution between A and B?
To find the coefficient of restitution (e) between two identical particles A and B during a one-dimensional collision, we can follow these steps:
Step 1: Understand the Initial Conditions
- Particle A has an initial momentum p.
- Particle B is stationary, so its initial momentum is 0.
Step 2: Define Impulse
- During the collision, particle B gives an impulse J to particle A.
- By the definition of impulse, the change in momentum of A due to the impulse is given by:
J=p′A−pA
where p′A is the momentum of A after the collision and pA is the momentum of A before the collision.
Step 3: Write the Momentum Equations
- Before the collision:
- Momentum of A: p
- Momentum of B: 0
- After the collision:
- Momentum of A: p′A=p+J
- Momentum of B: p′B=J (since B receives an equal and opposite impulse)
Step 4: Calculate the Final Momenta
- For particle A:
p′A=p+J
- For particle B:
p′B=J
Step 5: Define the Coefficient of Restitution
The coefficient of restitution e is defined as the ratio of the relative velocity of separation to the relative velocity of approach:
e=Velocity of separationVelocity of approach
Step 6: Calculate the Velocities
- Velocity of approach before collision:
vapproach=pm−0=pm
(where m is the mass of the particles, and since they are identical, we can use m for both).
- Velocity of separation after collision:
vseparation=p′Bm−p′Am=Jm−p+Jm=J−(p+J)m=−pm
Step 7: Substitute into the Coefficient of Restitution Formula
Substituting the velocities into the coefficient of restitution formula:
e=−pmpm=−pp=−1
Step 8: Adjust for the Impulse
However, we need to account for the impulse J given to A. The correct expression for e considering the impulse is:
e=2J−pp
This is derived from the relationship between the momenta and the impulses exchanged during the collision.
Final Answer
Thus, the coefficient of restitution e between particles A and B is given by:
e=2J−pp
- Particle A has an initial momentum p.
- Particle B is stationary, so its initial momentum is 0.
- During the collision, particle B gives an impulse J to particle A.
- By the definition of impulse, the change in momentum of A due to the impulse is given by:
J=p′A−pA
where p′A is the momentum of A after the collision and pA is the momentum of A before the collision.
- Before the collision:
- Momentum of A: p
- Momentum of B: 0
- After the collision:
- Momentum of A: p′A=p+J
- Momentum of B: p′B=J (since B receives an equal and opposite impulse)
- For particle A:
p′A=p+J
- For particle B:
p′B=J
The coefficient of restitution e is defined as the ratio of the relative velocity of separation to the relative velocity of approach:
e=Velocity of separationVelocity of approach
- Velocity of approach before collision:
vapproach=pm−0=pm
(where m is the mass of the particles, and since they are identical, we can use m for both).
vseparation=p′Bm−p′Am=Jm−p+Jm=J−(p+J)m=−pm
Substituting the velocities into the coefficient of restitution formula:
e=−pmpm=−pp=−1
However, we need to account for the impulse J given to A. The correct expression for e considering the impulse is:
e=2J−pp
This is derived from the relationship between the momenta and the impulses exchanged during the collision.
Thus, the coefficient of restitution e between particles A and B is given by:
e=2J−pp
Topper's Solved these Questions
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - More a than one option is Correct
1 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Medical entrances gallery
27 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
Similar Questions
In a one dimensional collision between two identical particles A and B, B is stationary and A has momentum p before impact. During impact, B gives impulse J to A.
View SolutionIn one dimentional collision between two idential particles 'A' and 'B'. 'B' is stationary and 'A' has momentum 54 kg-m/ sec before impact. During impact , 'B' gives an impulse 3kg-m/sec to 'A' . The coefficient of restitution between A and B is
View Solution
Knowledge Check
In an elastic one dimensional collision between two particles the relative veloctiy of approach before collision is
Agreater than the relative velocity of separation after collisionBless than the relative velocity of separation after collisionCequal to the relative velocity of separation after collisionDless than the relative velocity of separation if the incoming particle is heavier than the target particle
In a head on collision between two identical particles A and B moving on a horizontal surface, B is stationary and A has momentum P0 before impact. Due to the impact, A recrieves an impulse I. Then coefficient of restitution between the two is :
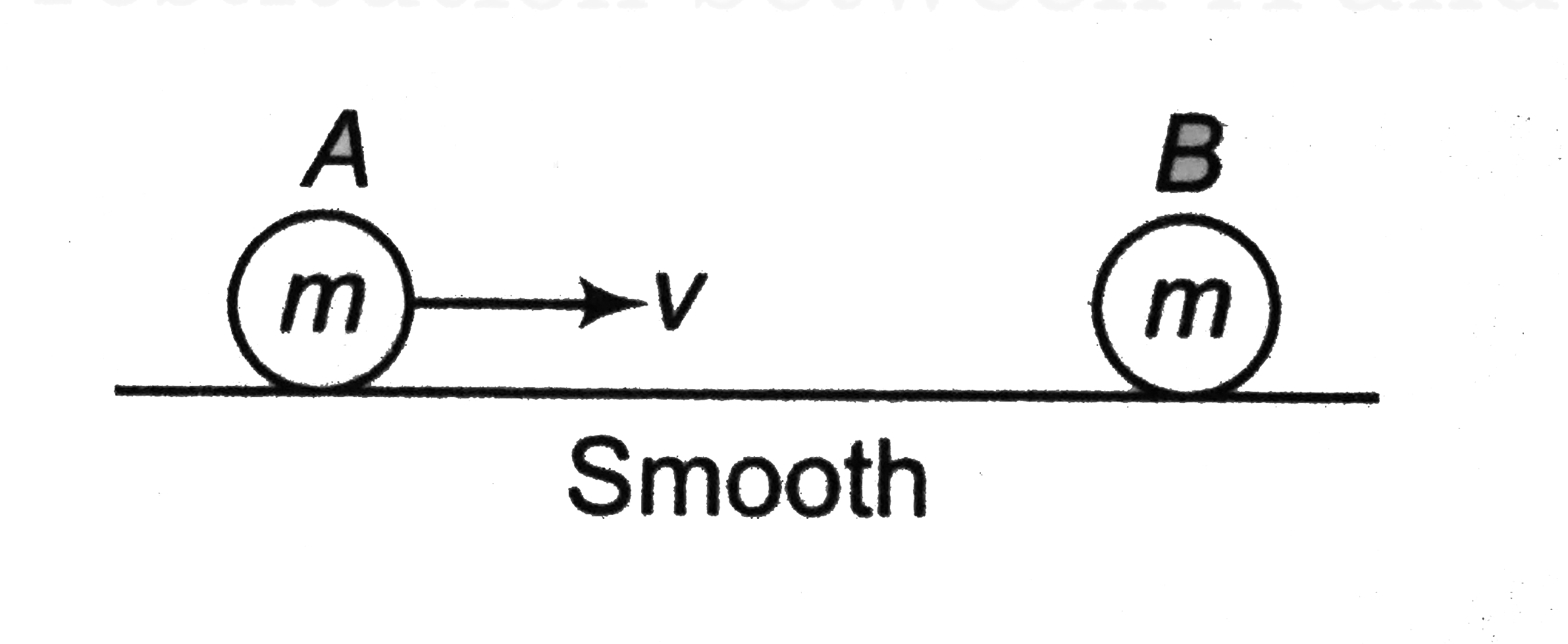
View SolutionIn the figure shown, coefficient of restitution between A and B is e=12, then
View SolutionA body X with a momentum p collides with another identical stationary body Y one dimensionally. During the collision, Y gives an impulse J to body X. Then coefficient of restitution is
View SolutionFind the single arithmetic mean between: (a−b)and(a+b)
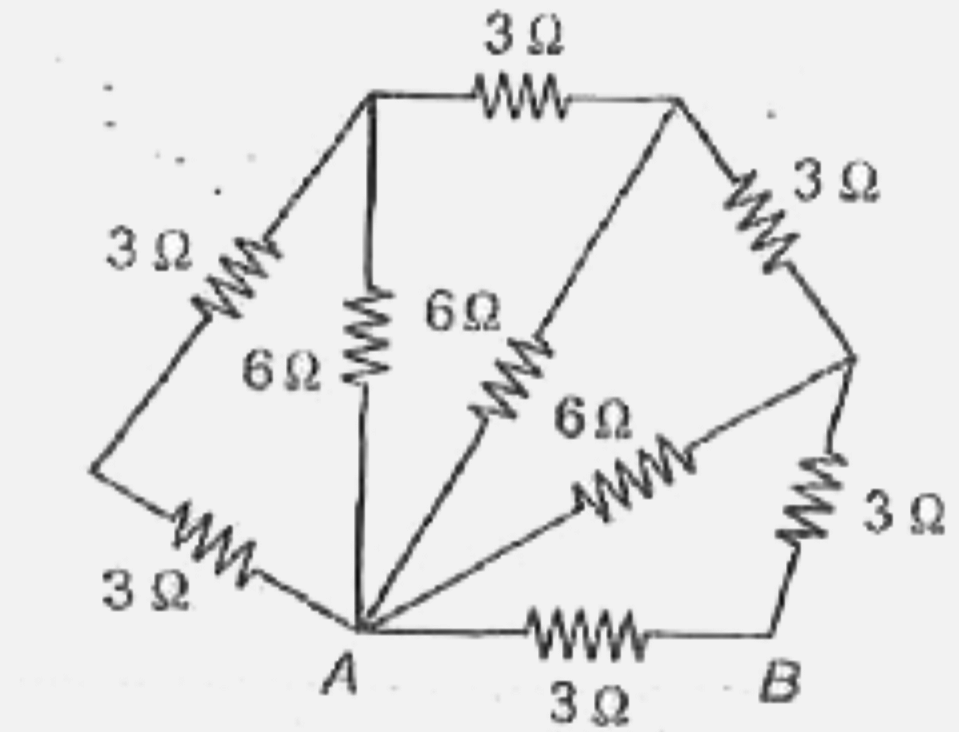
View SolutionFind the equivalent resistance between A and B in the give figure .
View SolutionA sphere A of mass m, travelling with speed v, collides directly with a stationary sphere B. If A is brought to rest and B is given a speed V, find (a) the mass of B (b) the coefficient of restitution between A and B?
View SolutionTwo billiard balls of same size and mass are in contact on a billiard table. A third ball of same mass and size strikes them symmetrically and remains at rest after the impact. Find the coefficient of restitution between the balls?
View Solution
