In a two diamensional motion of a particle, the particle moves from point A, with position vector ${\to r}_{1}$ to point B, with position vector ${\to r}_{2}$ . If the magnitudes of these vectors are, respectively, $r_{1} = 3$ and $r_{2} = 4$ and the angles they make with the x-axis are $θ_{1} = 75^{\circ}$ and $θ_{2} = 15^{\circ}$ , respectively, then find the magnitude of the displacement vector.

A

15

B

$\sqrt{13}$

C

17

D

$\sqrt{15}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

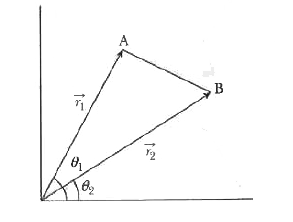
Displacement $= - - \to A B$ , angle between ${\to r}_{1}$ and ${\to r}_{2}$ is $θ = 75^{\circ} - 15^{\circ} = 60^{\circ}$
From (Figure), $A B^{2} = r_{1}^{2} + r_{2}^{2} - 2 r_{1} r_{2} cos θ$
$= 3^{2} + 4^{2} - 2 \times 3 \times 4 {cos 60}^{\circ} = 13$
$\Rightarrow A B = \sqrt{13}$

|

Updated on:21/07/2023

Class 11 PHYSICS VECTOR

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
In a two diamensional motion of a particle, the particle moves from point A, with position vector ${\to r}_{1}$ to point B, with position vector ${\to r}_{2}$ . If the magnitudes of these vectors are, respectively, $r_{1} = 3$ and $r_{2} = 4$ and the angles they make with the x-axis are $θ_{1} = 75^{\circ}$ and $θ_{2} = 15^{\circ}$ , respectively, then find the magnitude of the displacement vector.

A15
B $\sqrt{13}$
C17
D $\sqrt{15}$
Question 2 - Select One
In a two-dimensional motion of a particle, the particle moves from point A of position vector ${\to r}_{1},$ to point B of vectors are , respectively, $r_{1} = 3 and r_{2} = 4$ and the angles they make with the x axis are $θ_{1} = 75^{\circ} and θ_{2} = 15^{\circ},$ respectively, then find the magnitude of the displacement vector.

A15
B $\sqrt{13}$
C $17$
D $\sqrt{15}$
Question 3 - Select One
The displacement of a particle from a point having position vector $2 ˆ i + 4 ˆ j$ to another point having position vector $5 ˆ j + 1 ˆ j$ is
A3 units
B $3 \sqrt{2}$ units
C5 units
D $5 \sqrt{3}$ units

CENGAGE PHYSICS-VECTORS-Exercise Single Correct

Home
Ask Doubt
Profile