In an experiment for measuring 'g', a body is thrown vertically up in an evacuated tube and allowed to come back. If is the time interval between the two passages of the object across a lower level and the time interval between two passages across an upper level and H the distance between two levels as shown in Fig. 5.70 show that:
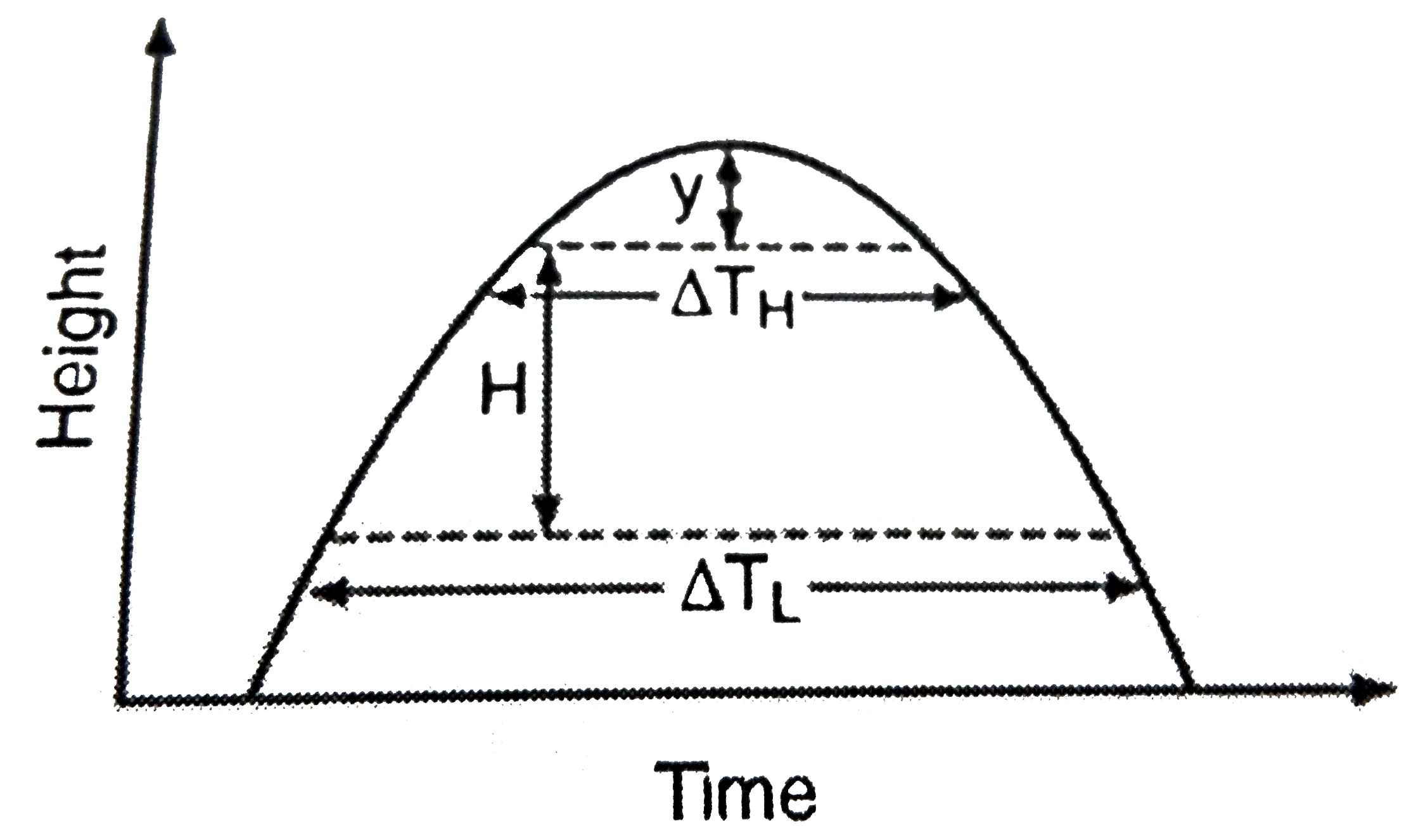
Time taken by a body to go up and come down a height h is giving by t=2, . So , if y is the height of highest point above higher level, time taken to go up and come down while for lower level
.
or
Topper's Solved these Questions
Similar Questions
An onject is thrown vertically upward with some speed. It crosses 2 points p,q whith are separated by (h) metre. If (t) is the time between (p) and highest point and coming back and tq is the time between q and highest point and coming back, relate acceleration due to gravity tp,tqandh.
View SolutionTwo particles are thrown from same point with same velocity with some time interval between them.Horizontal component of projection velocity is u.The Relative angular velocity of the line joining the two particles when they are at same horizontal level is (n)gu ,then value of n is
View Solution
Knowledge Check
A particle is projected with velocity 2√gh so that it just clears two walls of equal height h which are at a distance 2h from each other. Show that the time of passing between the walls is 2√h/g.
A2hgB√2hgC√hgD2√hgA particle is projected with velocity 2√gh so that it just clears two walls of equal height h which are at a distance 2h from each other. Show that the time of passing between the walls is 2√h/g.
A√hgB√2hgC2√hgD2hgA motorboat covers the distance between two spots on the river banks t1=8g and t2=12h in down stream and upstream respectively. The time required for the boat to cover this distance in still water will be
A6.9hB9.6hC69sD96s
If A(0,2,3),B=(2,−1,5) are two vertices of ΔABC whose orthocentre is H .Then distance between the orthocentres of ΔHAC and ΔHBC is
View SolutionFrom airport two aeroplanes start at the same time . If the speed of first aeroplane due North is 500 km/h and that of other due East is 650 km/h , then find the distance between two aeroplanes after 2 hours .
View SolutionTwo rods of the same proper length l0 move toward each other parallel to a common horizontal axis. In the reference frame fixed to one of the rods the time interval between the moments, when the right and left ends of the rods coincide, is equal to Δt. What is the velocity of one rod relative to the other?
View SolutionAt two points of an inertial reference frame separated by a distance along the x-axis of l=x2−x1 two events take place slmultaneously. Find the time interval between the events in an !arbitrary inertial reference frame.
View SolutionIf a satellite is travelling in the same direction as the rotation of earth i.e., west to east, what is the interval between two successive times at which it will appear vertically overhead to an observer at a fixed point on the equator? (R = 6400 km, h = 1400 km)
View Solution
