In an uniform electric field of strength E, a charge particle Q moves point A to point B in the direction of the field and back from B to A. Calculate the ratio of the work done by the electric field in taking the charge particle from A to B and from B to A.
To solve the problem of calculating the ratio of work done by the electric field when a charged particle moves from point A to point B and back from point B to point A, we can follow these steps:
1. Understanding the Electric Field:
- We have a uniform electric field E directed from point A to point B.
- A charge Q is moved from A to B in the direction of the electric field.
2. Work Done from A to B:
- When the charge Q moves from A to B, the work done by the electric field W1 can be calculated using the formula:
W1=F⋅d⋅cos(θ)
- Here, F is the force acting on the charge due to the electric field, d is the distance between A and B, and θ is the angle between the force and the displacement.
- Since the force F=Q⋅E acts in the same direction as the displacement, θ=0∘ and cos(0)=1.
- Therefore, the work done from A to B is:
W1=Q⋅E⋅d⋅1=Q⋅E⋅d
3. Work Done from B to A:
- When the charge Q moves back from B to A, the work done by the electric field W2 is calculated similarly:
W2=F⋅d⋅cos(θ)
- In this case, the force F=Q⋅E is still in the direction of the electric field, but the displacement is in the opposite direction. Thus, θ=180∘ and cos(180)=−1.
- Therefore, the work done from B to A is:
W2=Q⋅E⋅d⋅(−1)=−Q⋅E⋅d
4. Calculating the Ratio:
- We need to find the ratio of the work done from A to B and from B to A:
W1W2=Q⋅E⋅d−Q⋅E⋅d
- Simplifying this gives:
W1W2=−1
Final Result:
The ratio of the work done by the electric field in taking the charge particle from A to B and from B to A is:
W1W2=−1
- We have a uniform electric field E directed from point A to point B.
- A charge Q is moved from A to B in the direction of the electric field.
- When the charge Q moves from A to B, the work done by the electric field W1 can be calculated using the formula:
W1=F⋅d⋅cos(θ)
- Here, F is the force acting on the charge due to the electric field, d is the distance between A and B, and θ is the angle between the force and the displacement.
- Since the force F=Q⋅E acts in the same direction as the displacement, θ=0∘ and cos(0)=1.
- Therefore, the work done from A to B is:
W1=Q⋅E⋅d⋅1=Q⋅E⋅d
- When the charge Q moves back from B to A, the work done by the electric field W2 is calculated similarly:
W2=F⋅d⋅cos(θ)
- In this case, the force F=Q⋅E is still in the direction of the electric field, but the displacement is in the opposite direction. Thus, θ=180∘ and cos(180)=−1.
- Therefore, the work done from B to A is:
W2=Q⋅E⋅d⋅(−1)=−Q⋅E⋅d
- We need to find the ratio of the work done from A to B and from B to A:
W1W2=Q⋅E⋅d−Q⋅E⋅d
- Simplifying this gives:
W1W2=−1
The ratio of the work done by the electric field in taking the charge particle from A to B and from B to A is:
W1W2=−1
Topper's Solved these Questions
- ELECTROSTATICS AND CURRENT ELECTRICITY BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ELECTROSTATICS AND CURRENT ELECTRICITY EXERCISE - SHORT ANSWER QUESTIONS (2 MARKS)
59 Videos
- ELECTROSTATICS AND CURRENT ELECTRICITY BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ELECTROSTATICS AND CURRENT ELECTRICITY EXERCISE - SHORT ANSWER QUESTIONS (3 MARKS )
23 Videos
- ELECTRONIC DEVICES BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ELECTRONIC DEVICES EXERCISE - NUMERICALS
7 Videos
- SAMPLE QUESTION PAPER 2019BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - SAMPLE QUESTION PAPER 2019EXERCISE - Section C
19 Videos
Similar Questions
Knowledge Check
If the test charge is moved back to R from P, then work done by electric field is
A3 JBzeroCcannot be determinedDNone of the aboveA charged particle moving in an electric field
Amust undergo change in velocityBmust undergo change in speedCmay not undergo change in velocityDmay not undergo change in speedIn the electric field of a point chargde q, a cetrain charge is carried from point A to B,C,D and E. Then the ork done
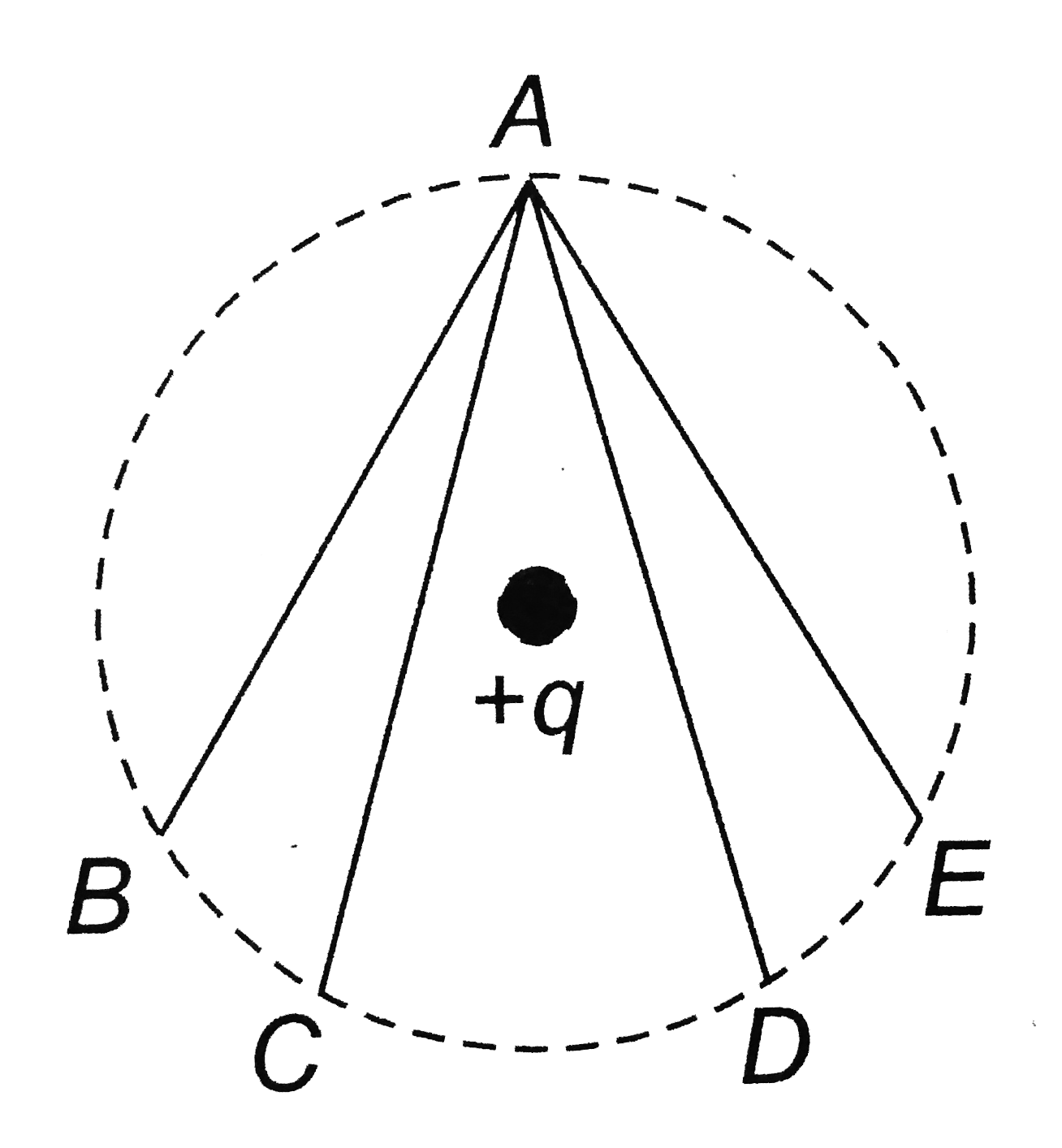 Ais least along the path ABBis least along the path ADCis zero along all the paths AB,AC,ADandAEDis least along AE
Ais least along the path ABBis least along the path ADCis zero along all the paths AB,AC,ADandAEDis least along AE
The electric field of a point charge is uniform. Is it true or false?
View SolutionA charged particle always move in the direction of electric field. Is this statement true or false?
View SolutionA point charge q is rotated along a circle in the electric field generated by anotherj point charge Q. The work done by the electric field on the rotatin charge in one complete revolution is
View SolutionIf a particle moves on an equipotential surface of an external field, work done by the electric field on the charge is
View SolutionIn the electric field of charge Q, another charge is carried from A to B, A to C, A to D and A to E, then work done will be :
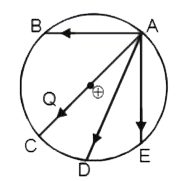
View Solution
