In Fig. 16-13, two strings have been tied together with a knot and then stretched between two rigid supports. The strings have linear densities $μ_{1} = 1.4 \times 10^{- 4} kg/m and μ_{2} = 2.8 . \times 10^{- 4} kg/m .$ Their lengths are $L_{1} = 3.0 m and L_{2} = 2.0 m,$ and string 1 is under a tension of 400 N. Simultaneously, on each string a pulse is sent from the rigid support end, toward the knot. Which pulse reaches the knot first.

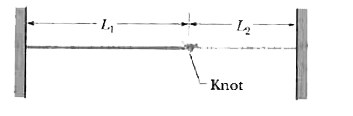
Figure 18-13 Two strings, of lengths $L_{1} and L_{2}$ , tied together with a knot and stretched between two rigid supports.

Video Solution

Text Solution

Verified by Experts

(1) The time t taken by a pulse to travel a length L is t=L//v, where v is the constant speed of the pulse.
(2) The speed of a pulse on a strectched string depends on the string.s tension $τ$ and linear density $μ$ , and is given by equation $v = \sqrt{τ / v}$ .
(3) Because the two strings are stretched together, they must both be under the same tension $τ (= 40 N) .$
Calculations: Putting these three ideas together gives us, as the time for the pulse on string 1 to reach the knot,
$t_{1} = \frac{L_{1}}{v_{1}} = L_{1}, \sqrt{\frac{u_{1}}{τ}} = (3.0 m) \frac{\sqrt{(1.4 \times 10^{- 4} k g / m)}}{400 N}$
$= 1.77 \times 10^{- 3} s$
Similarly, the data for the pulse on string 2 give us
$t_{2} = L_{2} \sqrt{\frac{u_{2}}{τ}} = 1.67 \times 10^{- 3} s$
Thus, the pulse on string 2 reaches the knot first.
Now look back at the second point. The linear density of string 2 is greater than that of string 1, so the pulse on string 2 must be slower than that on string 1. Could we have guessed the answer from that lact alone? No, because from the first point we see that the distance traveled by a pulse also matters.

Updated on:21/07/2023