Two strings with linear mass densities μ1=0.1kg/m and μ2=0.3kg/m are joined seamlesly. They are under tension of 20N. A travelling wave of triangular shape is moving from lighter to heavier string.
The reflection coefficient at interface is zero
The reflection coefficient at interface is 2−√3
The transmission coefficient at interface is 1
The transmission coefficient at inerface is √3−1
The correct Answer is:B, D
V1=√Tμ1=10√2
V2=√Tμ2=10√2√3
Reflection coeffcient
R=V2−V1V1+V2=2−√3
Transmission coefficient
T=2V2V1+V2=√3−1
Topper's Solved these Questions
- TEST PAPERSBOOK - FIITJEECHAPTER - TEST PAPERSEXERCISE - PHYSICS
747 Videos
- SIMPLE HARMONIC MOTIONBOOK - FIITJEECHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Assignment Problems (Objective) (Level-II)
20 Videos
- WORK, ENERGY AND POWER BOOK - FIITJEECHAPTER - WORK, ENERGY AND POWER EXERCISE - COMPREHENSIONS ( Comprehension-II)
5 Videos
Similar Questions
In Fig. 16-13, two strings have been tied together with a knot and then stretched between two rigid supports. The strings have linear densities μ1=1.4×10−4 kg/m andμ2=2.8.×10−4kg/m. Their lengths are L1=3.0mandL2=2.0m, and string 1 is under a tension of 400 N. Simultaneously, on each string a pulse is sent from the rigid support end, toward the knot. Which pulse reaches the knot first.
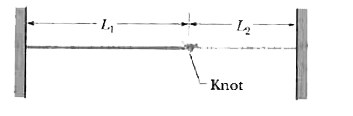
Figure 18-13 Two strings, of lengths L1andL2, tied together with a knot and stretched between two rigid supports.
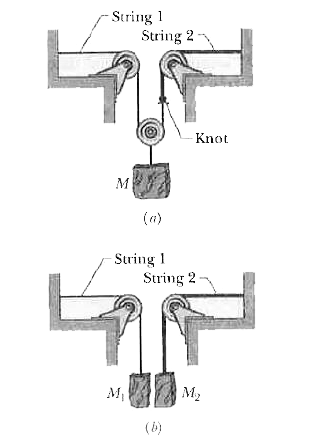
View SolutionIn fig. 16-47a, string 1 has a linear density of 3.00 g/m, and string 2 has a linear density of 5.00 g/m. They are under tension due to the hanging block of mass M= 800 g. Calculate the wave speed on (a) string 1 and (b) string 2 (Hint: When a string loops halfway around a pulley, it pulls on the pulley witii a net force that is twice the tension in the string) Next the block is divided into two blocks (with M1+M2=M) and the apparatus in tearranged as shown in fig. 16-47b. Find (c) M1and(d)M2 such that the wave speeds in the two strings are equal.
View Solution
Knowledge Check
The linear mass density μ of the string is (where, mass of the string = m, length, of the string g = L)
AmLBmLCmL2DmL3The linear mass density 'μ' of the string is (where, mass of the string = m, length of the string = L)
AmLBmLCmt2DmL3
Two blocks with masses m1=0.2 kg and m2=0.3 kg hang one under other as shown in figure. Find the tensions in the strings (massless) in the following situations :
(g=10m/s2)
they move upward at 5 m/s
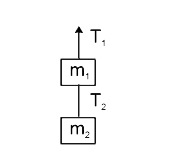
View SolutionTwo blocks with masses m1=0.2 kg and m2=0.3 kg hang one under other as shown in figure. Find the tensions in the strings (massless) in the following situations :
(g=10m/s2)
the blocks are at rest
View SolutionPower supplied to a vibrating string: A string with linear mass density μ=5.00×10−2kg/m is under a tension of 80.0 N. how much power must be supplied to the string to generate sinusoidal waves at a frequency of 60.0 HZ and an amplitude of 6.00 cm?
View SolutionTwo strings A and B are connected together end to end as shown in the figure. The ratio of mass per unit length μBμA=4. The tension in the string is same. A travelling wave is coming from the string A towards string B. if the fraction of the power of the incident wave that goes in sting B is n9 the value of n is:

View SolutionTwo blocks with masses m1=0.2 kg and m2=0.3 kg hang one under other as shown in figure. Find the tensions in the strings (massless) in the following situations :
(g=10m/s2)
if maximum allowable tension is 10 N. What is maximum possible upward acceleration ?
View SolutionTwo strings under the same tension T but with different values of the mass per unit length μ1 .and μ2 are connected together. A traveling wave pulse of amplitude 1cm is incident on the boundary,going from μ1 to μ2 .If μ2=0.1μ1 ,what is approximately the amplitude of the transmitted pulse?
View Solution
