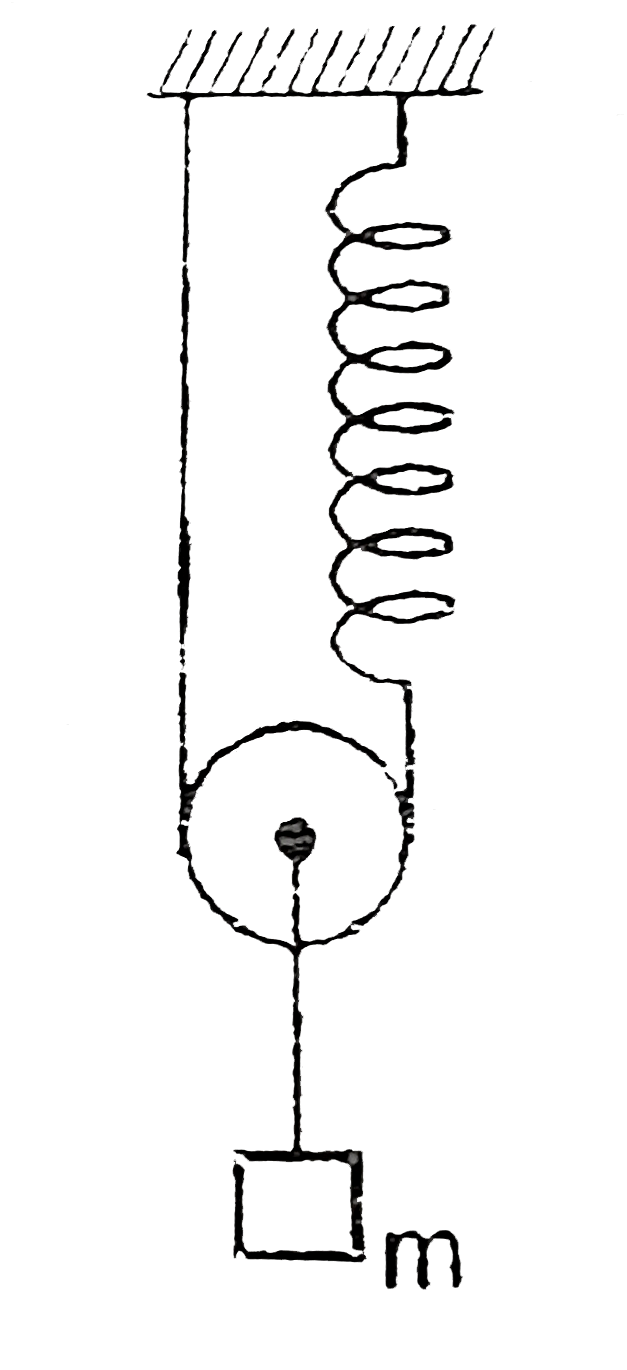
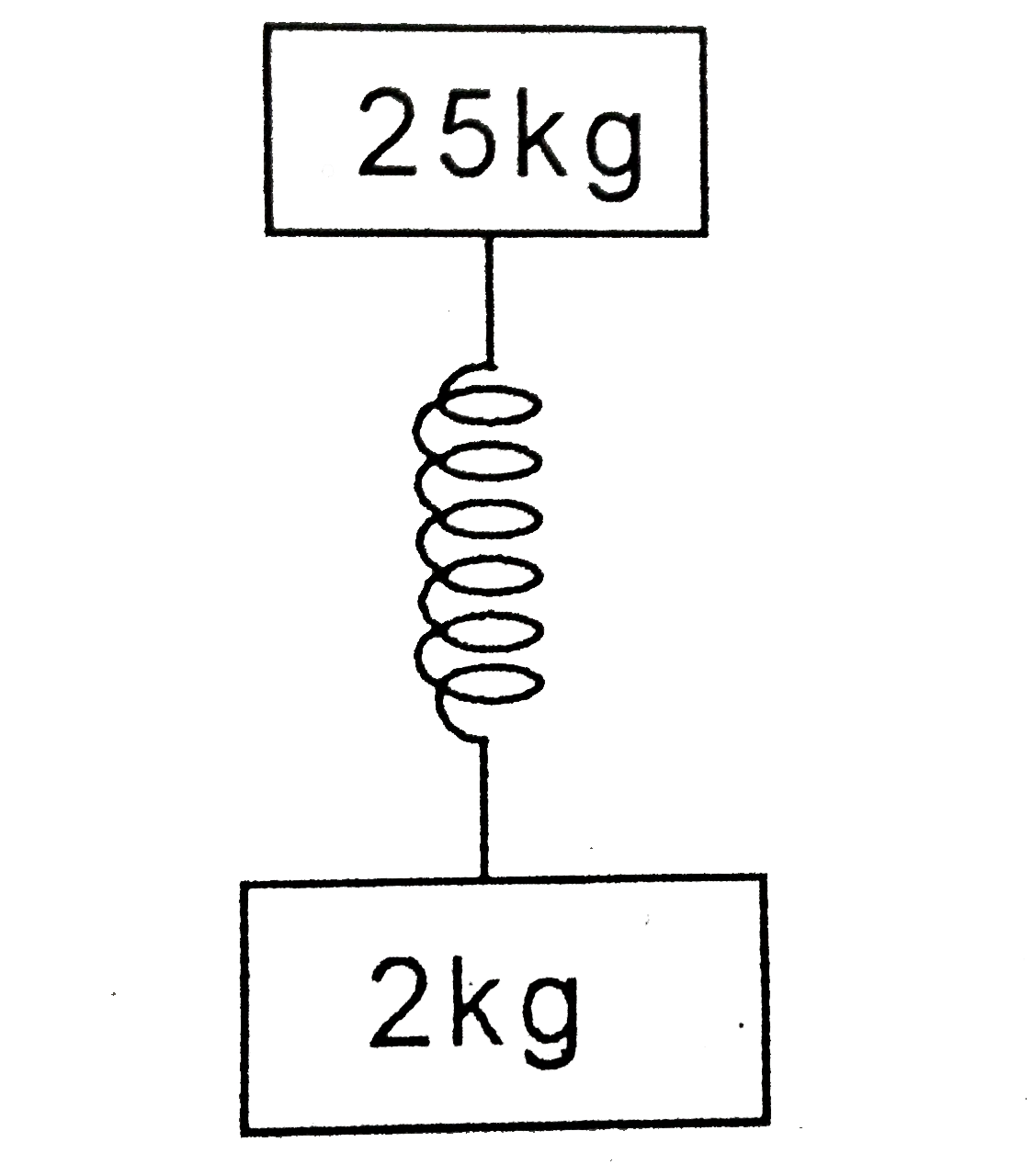
In figure, block A is released from rest, when spring is at its natural unstretched length. For block B of mass M to leave contact with the ground at some stage, the minimum mass of A must be
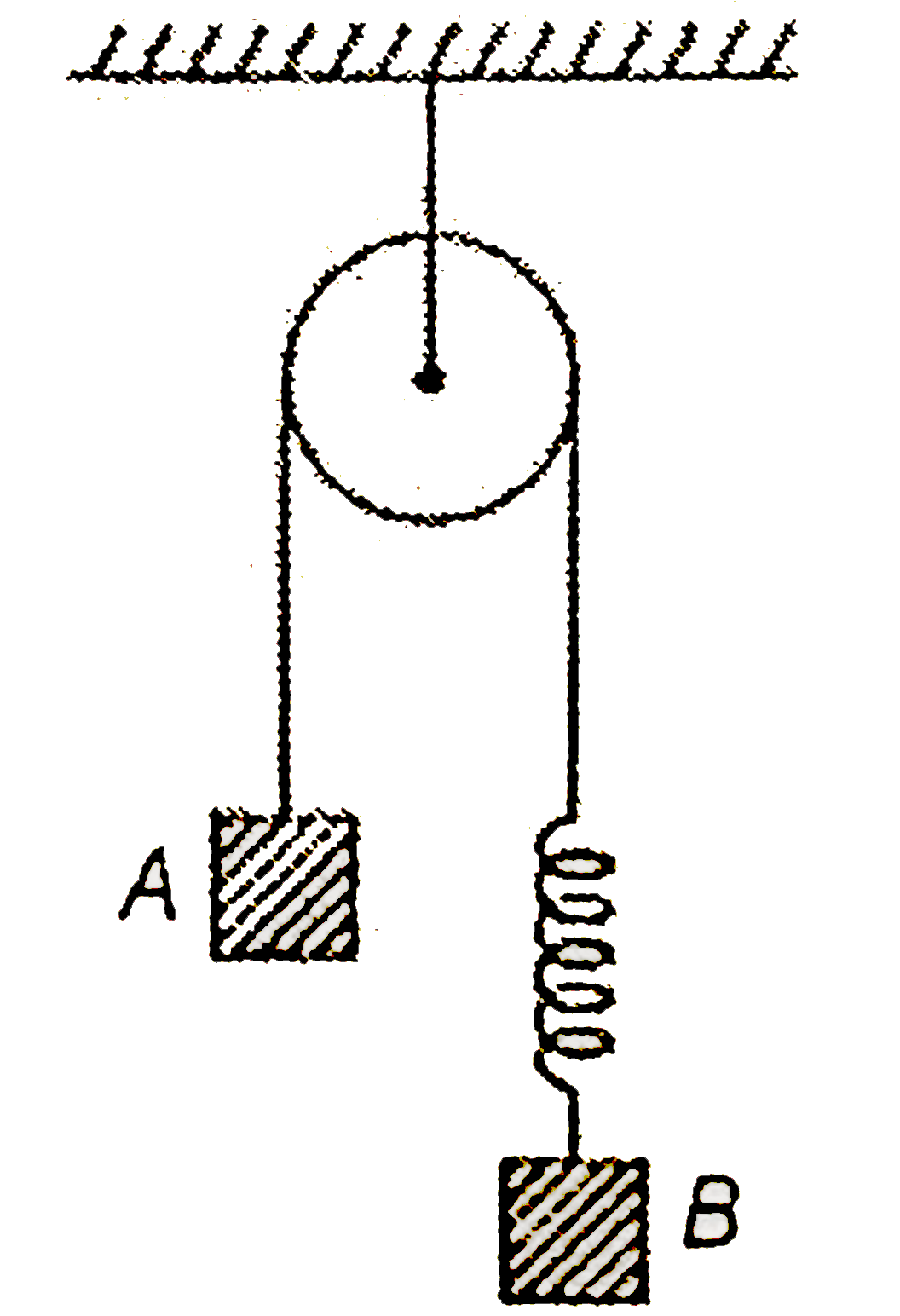
The correct Answer is:B
Let xmax=maximum extension of spring
Decrease in potential energy of A
= Increase in elastic potential energy of spring
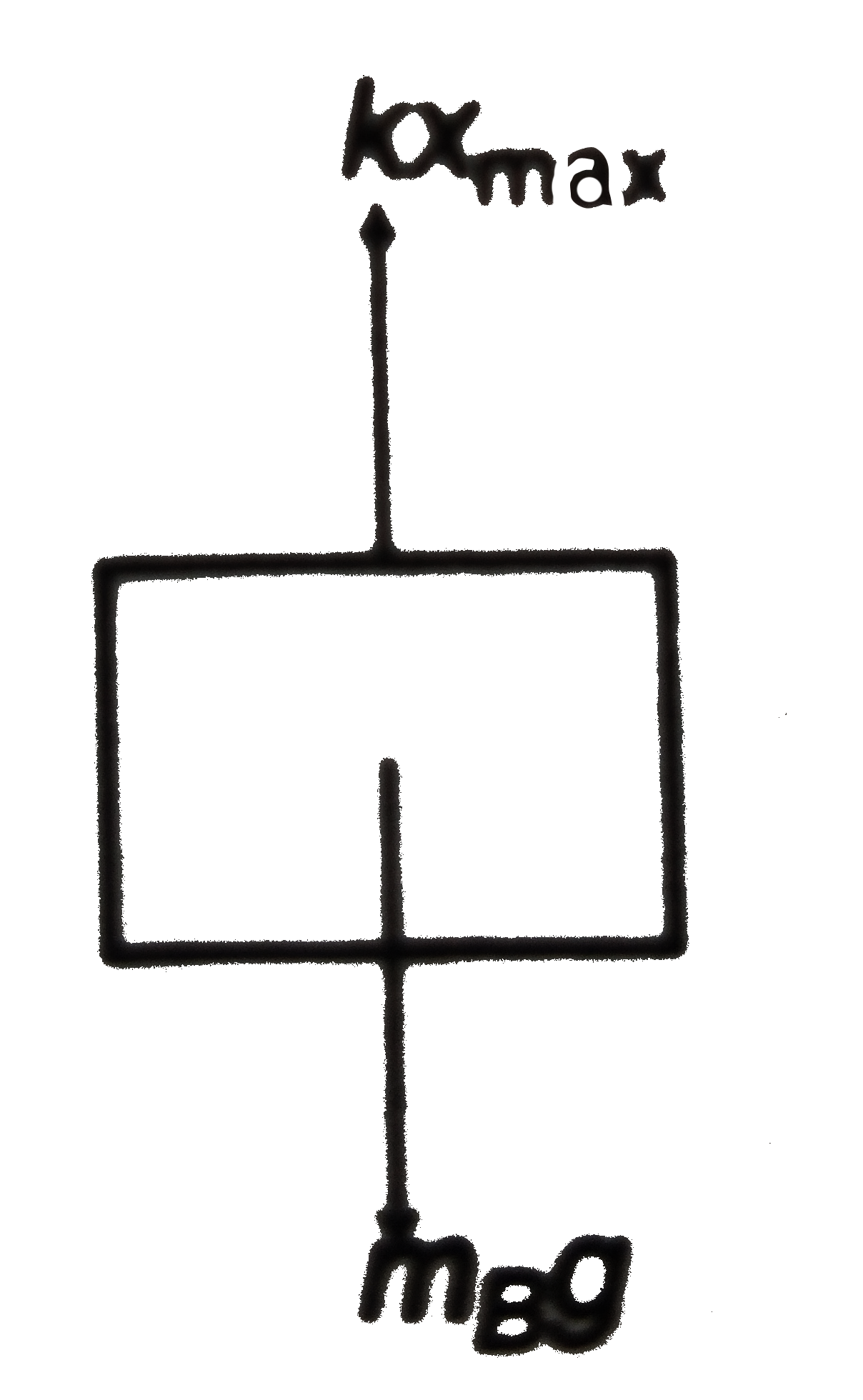
∴mAgxmax=12kmax_(max)2
∴xmax=2mAgk
To just lift the block B,
kxmax=mBg
∴2mAg=mBg=mg
∴mA=m2
.
Similar Questions
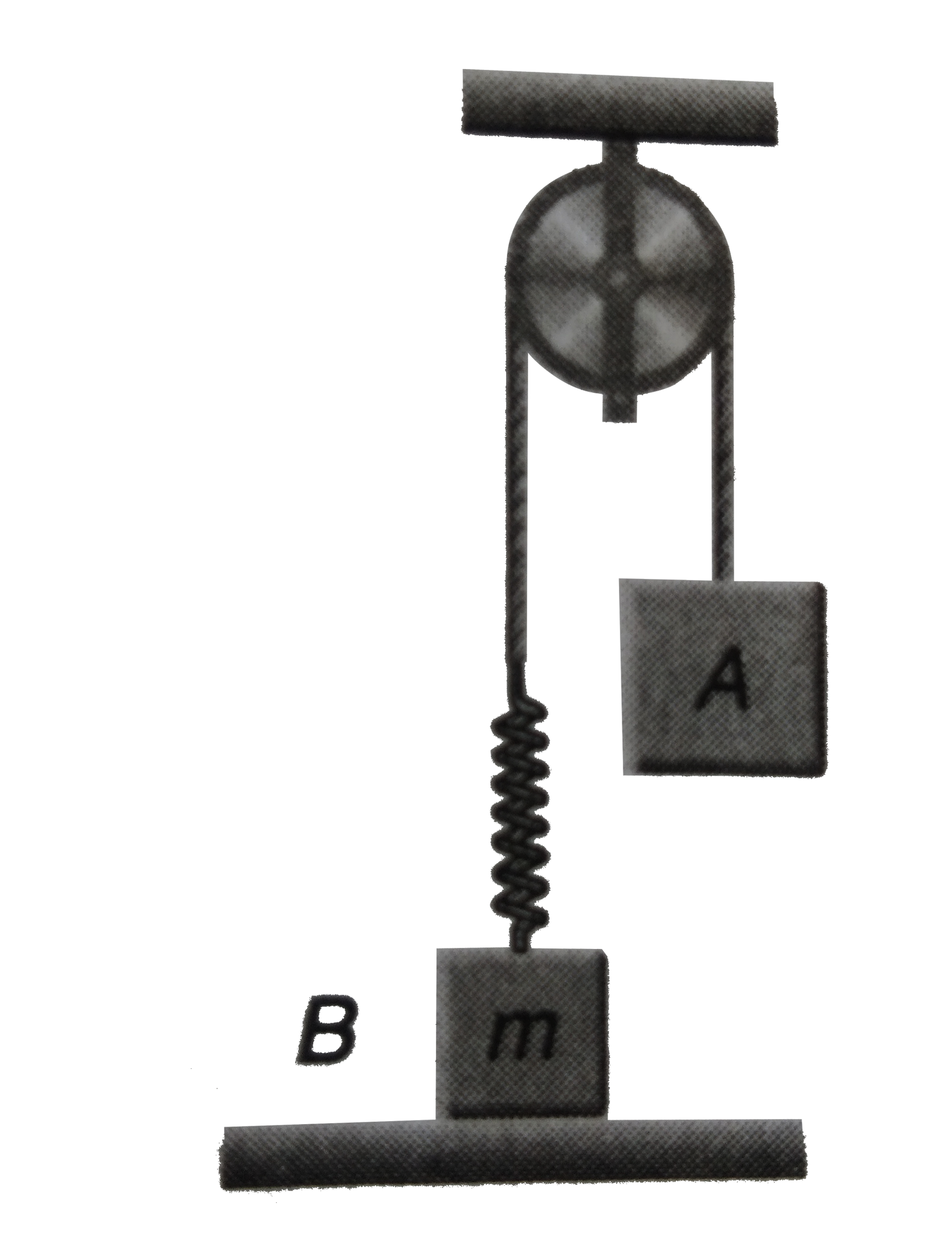
In the figure, block A is released from rest when the spring is its natural length for the block B of mass m to leave contact with the ground at some stage what should be the minimum mass of block A?
. View SolutionBlock m is released from rest when spring is in its natural length ( assume pulley is ideal and block does not strike on ground during it's motion in vertical plane )
than :
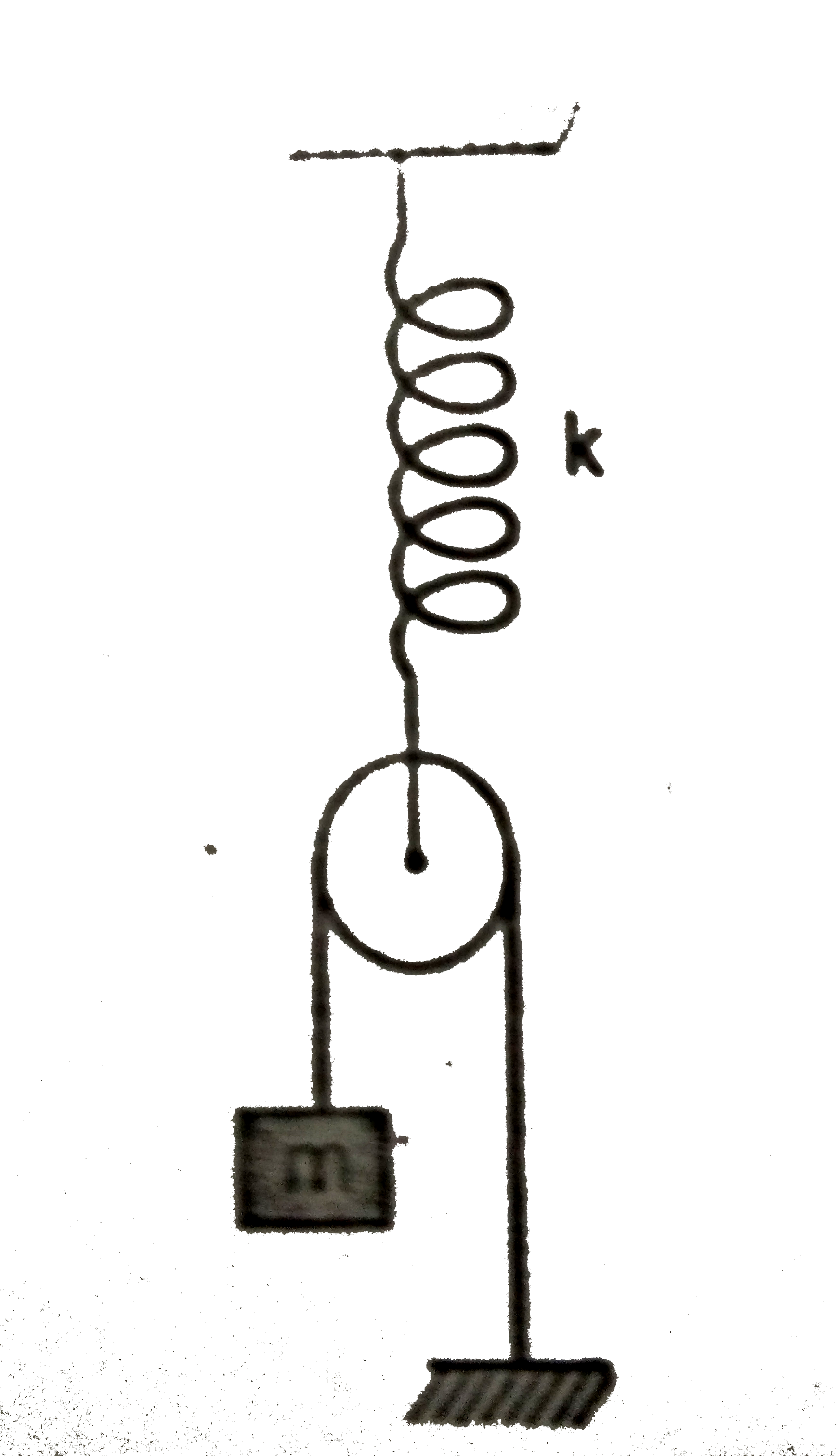View SolutionThe block of mass m is released when the spring was in its natrual length. Spring constant is k. Find the maximum elongation of the spring.
View SolutionThe system is released from rest with spring intially in its natural length. If mass of the block m = 10 kg. and spring constant k=100N/m, then maximum extension in spring is :
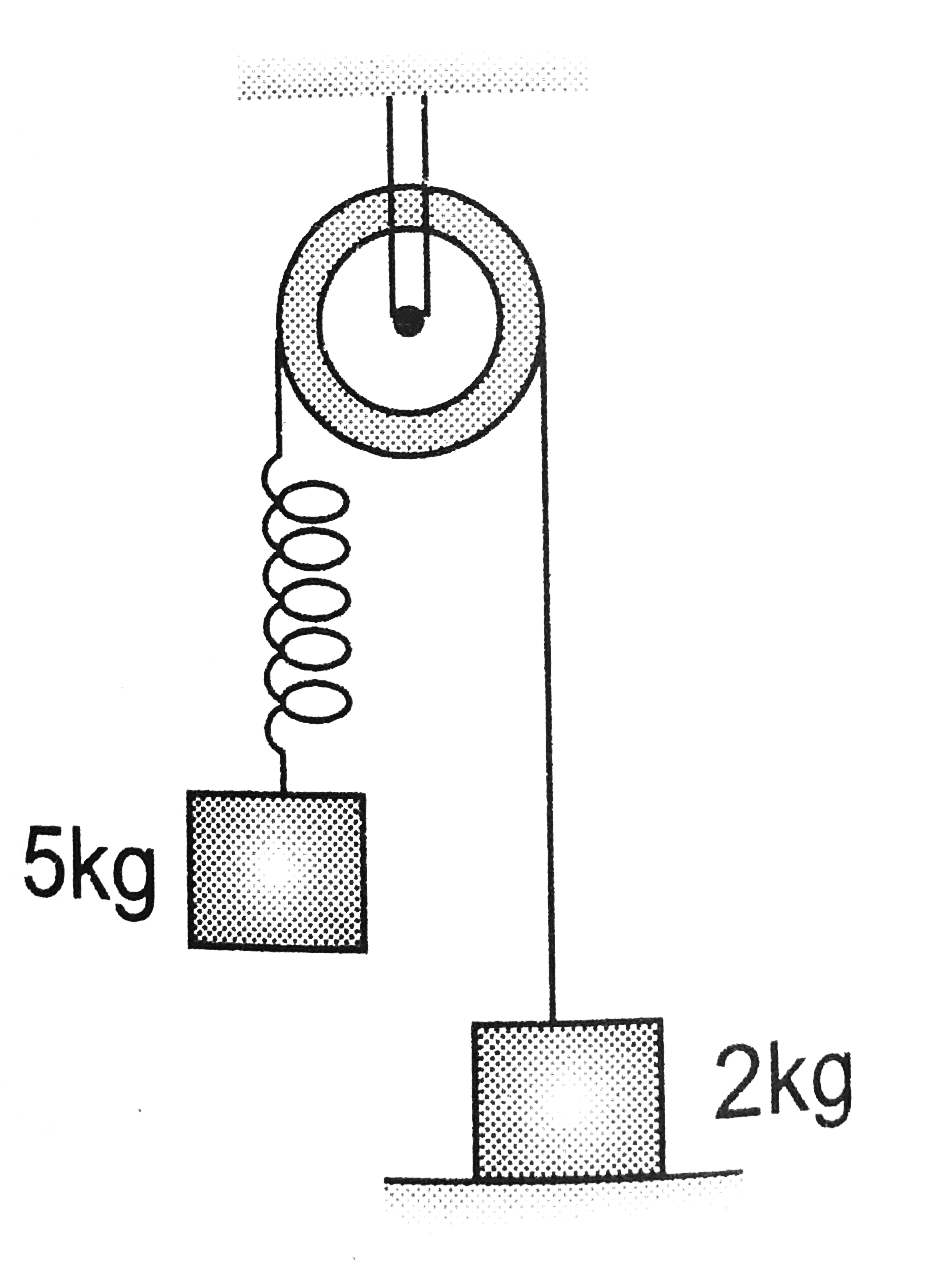
View SolutionSystem shown in figure is released from rest . Pulley and spring is mass less and friction is absent everywhere. The speed of 5kg block when 2kg block leaves the constant of with ground is (force constant of spring k=40N/mandg=10m/s2)
View SolutionA block suspended from a spring is released when the spring is unstretched. Then choose the correct options.
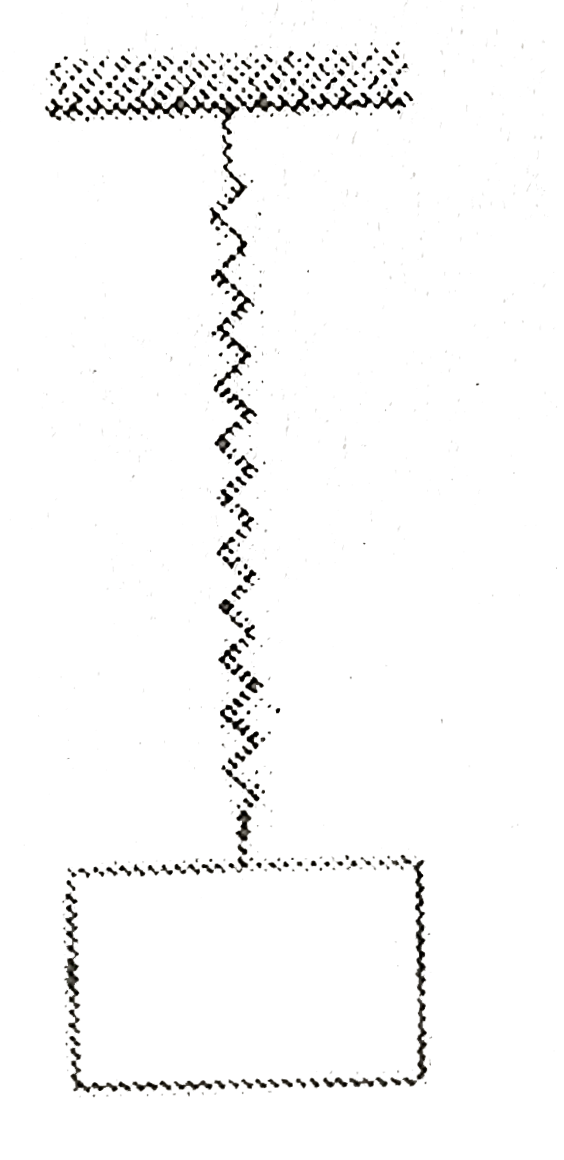View SolutionThe system is released from rest with both the springs in unstretched positions. Mass of each block is 1 kg and force constant of each spring is 10N/m.
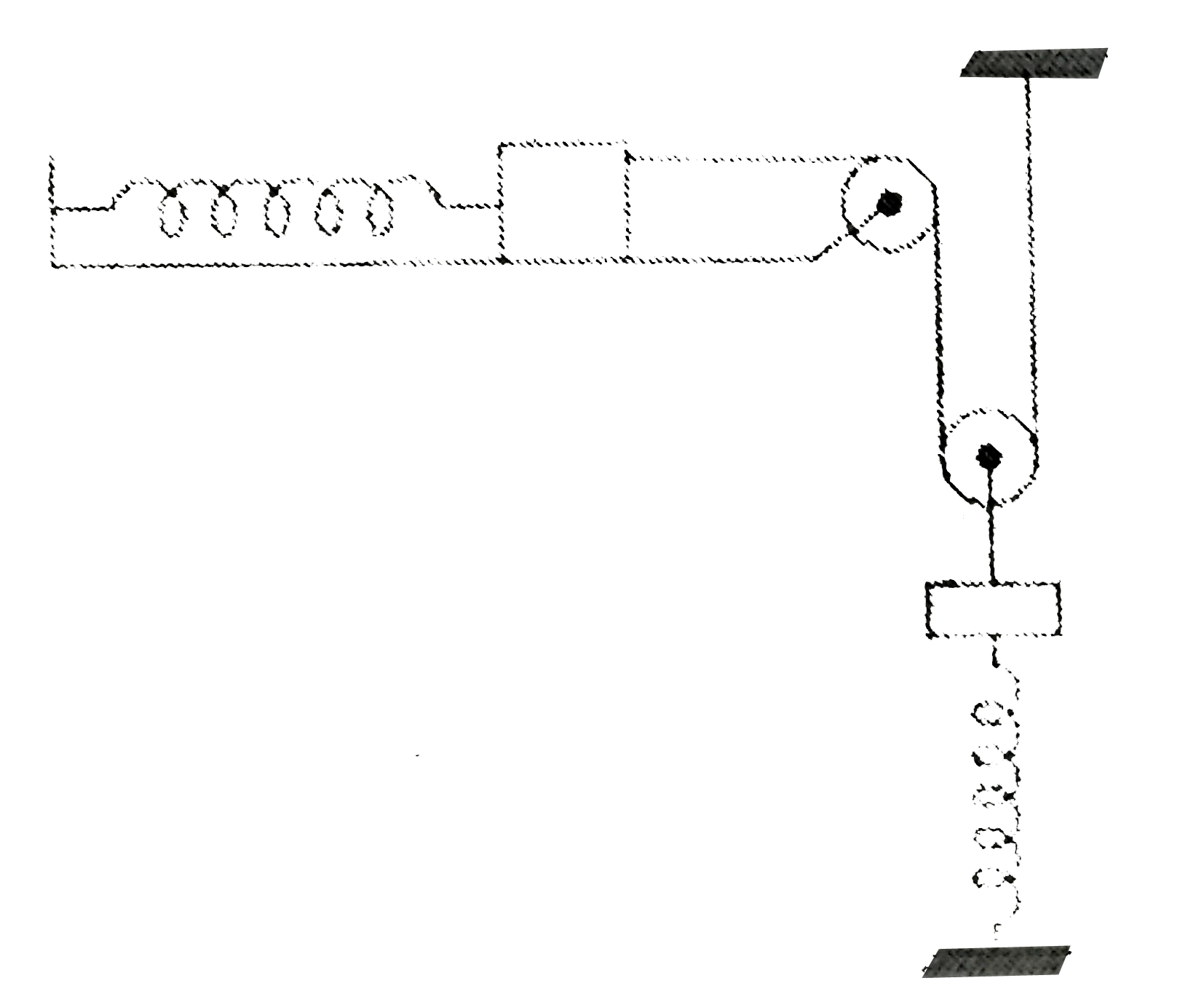
In the equilibrium position, speed of the block placed horizontally isView SolutionInitialy spring in its natural length now a block at mass 0.25 kg is released then find out maximum force by system on floor ?
View SolutionFind the maximum tension in the spring if initially spring at its natural length when block is released from rest.
View SolutionA block of mass m, attacted to a string of spring constant k, oscillates on a smooth horizontal table. The other end of the spring is fixed to a wall. The block has a speed v when the spring is at its natural length. Before coming to an instantaneous rest. If the block moves a distance x from the mean position, then
View Solution