Initialy spring in its natural length now a block at mass 0.25 kg is released then find out maximum force by system on floor ?
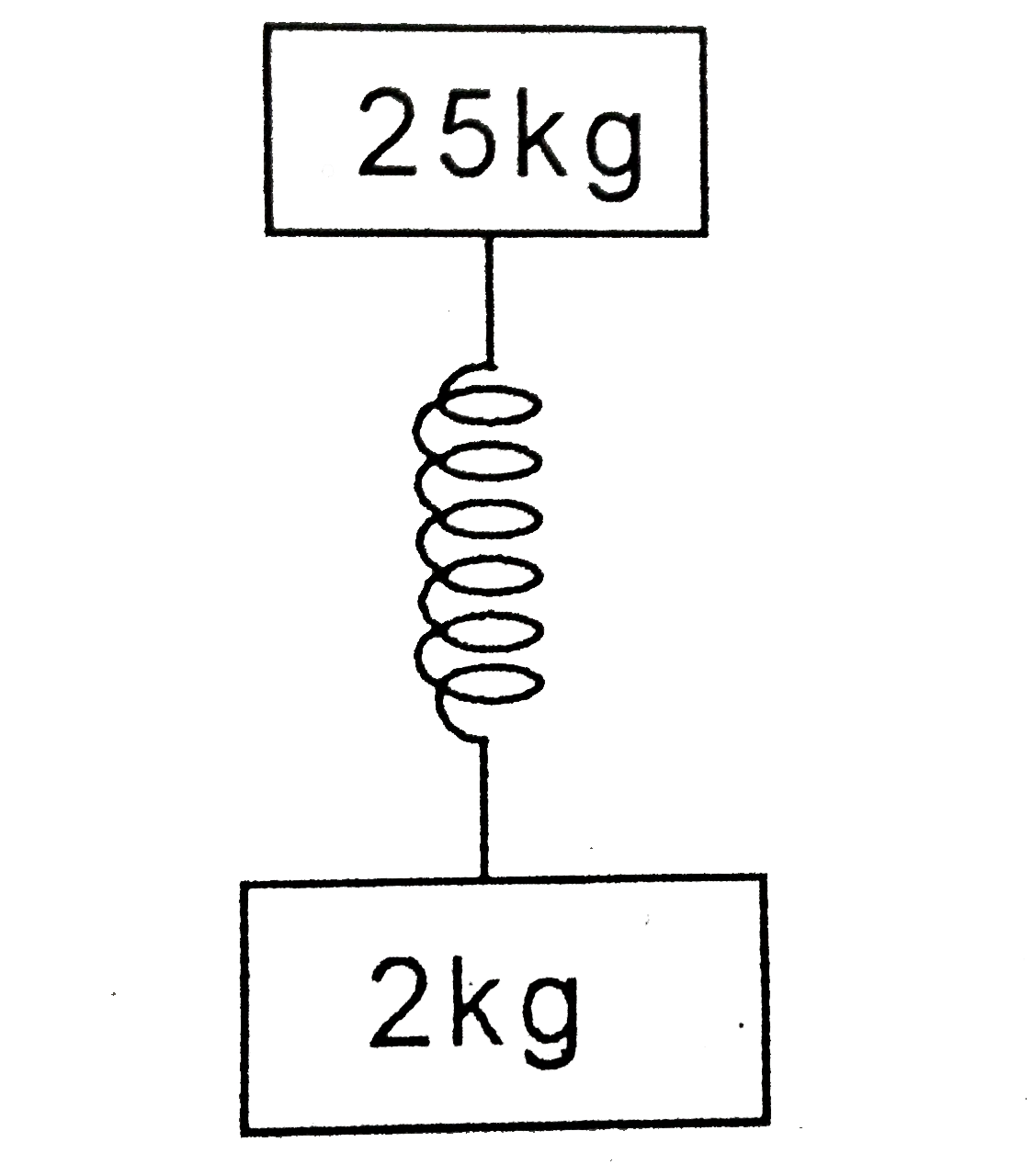
15 N
20 N
25 N
30 N
The correct Answer is:C
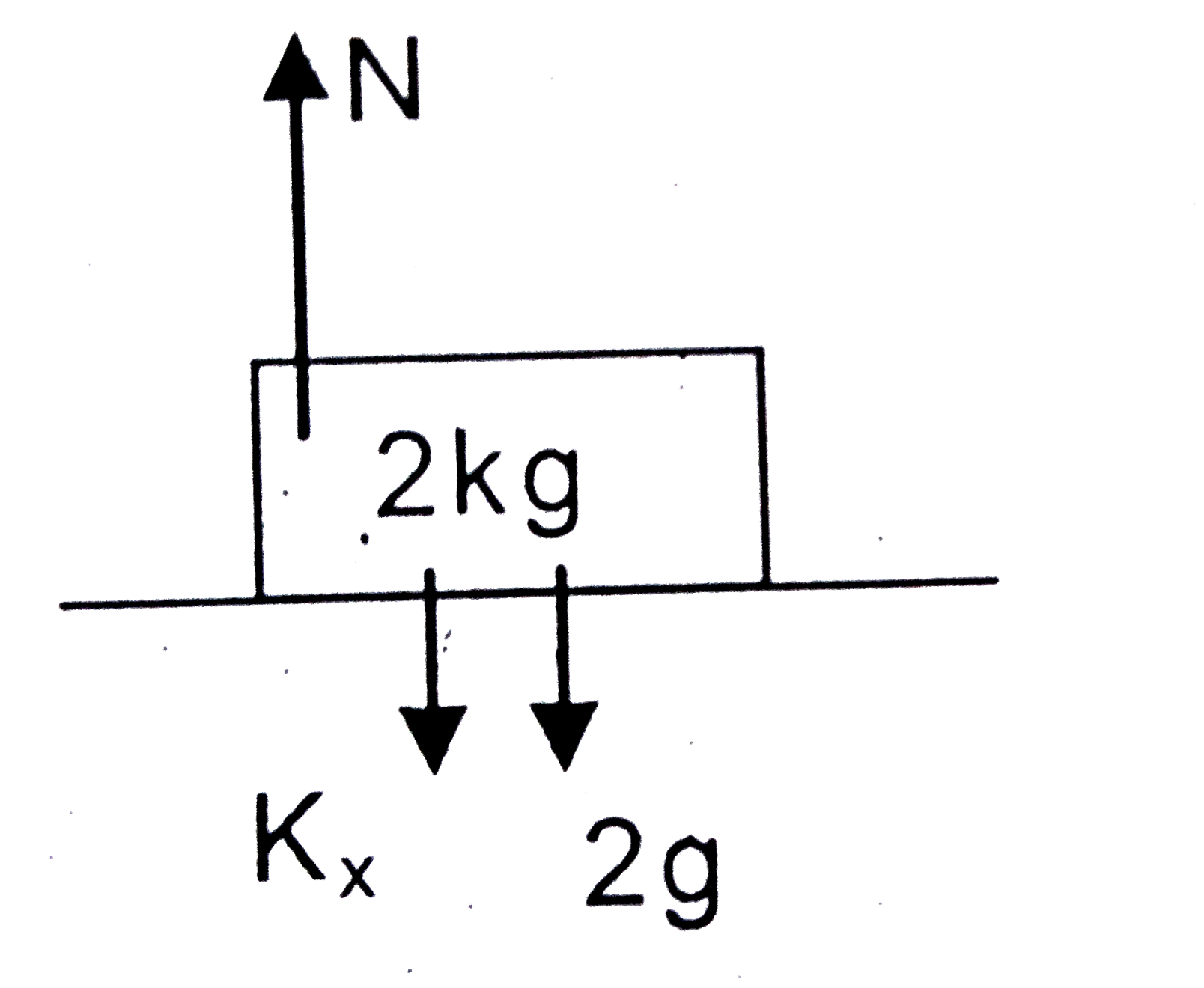
Work - energy theory on (25) kg
12Kx2=mgx
Kx=2mg
Kx=2×.25×10
=5N
= 25 N
N=kx+2g
=5+20=25N
Similar Questions
A block of mass m is attached with a spring in vertical plane as shown in the figure. If initially spring is in its natural length and the block is released from rest, then maximum extension in the spring will be
View SolutionInitialy spring is in the natural length and blocks A&B are at rest. Find maximum value of constant force F that can be applied on B such that block A remains at rest. (g=10m/s2) (given answer in Newton)
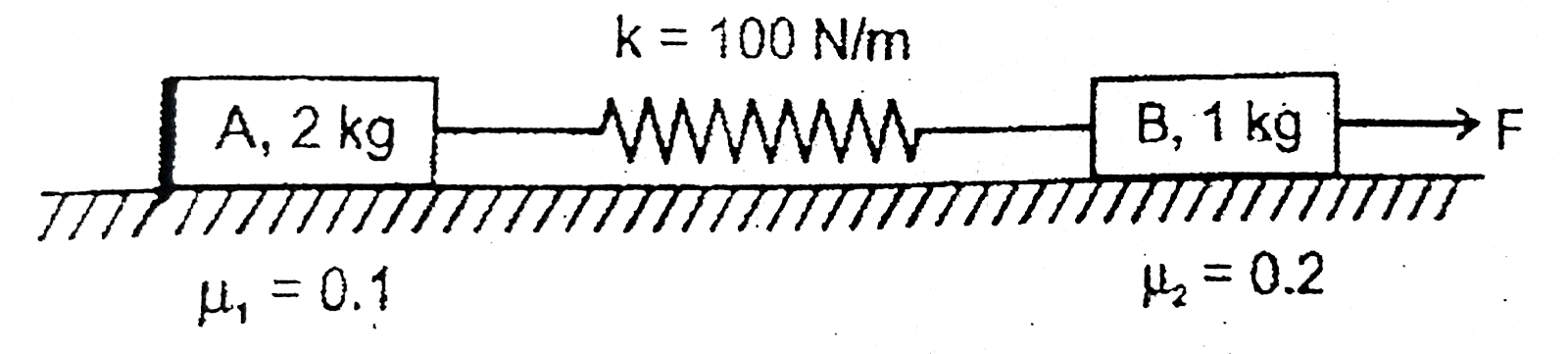
View SolutionA block of mass m is attached to a spring of force constant k whose other end is fixed to a horizontal surface. Initially the spring is in its natural length and the block is released from rest. The average force acting on the surface by the spring till the instant when the block has zero acceleration for the first time is

View SolutionBlock A of mass m is placed on a plank B. A light support S is fixed on plank B and is attached with the block A with a spring of spring constant K. Consider that initially spring is in its natural length. If the plank B is given an acceleration a, then maximum compression in the spring is xmak. Find the value of x (All the surface are smooth)

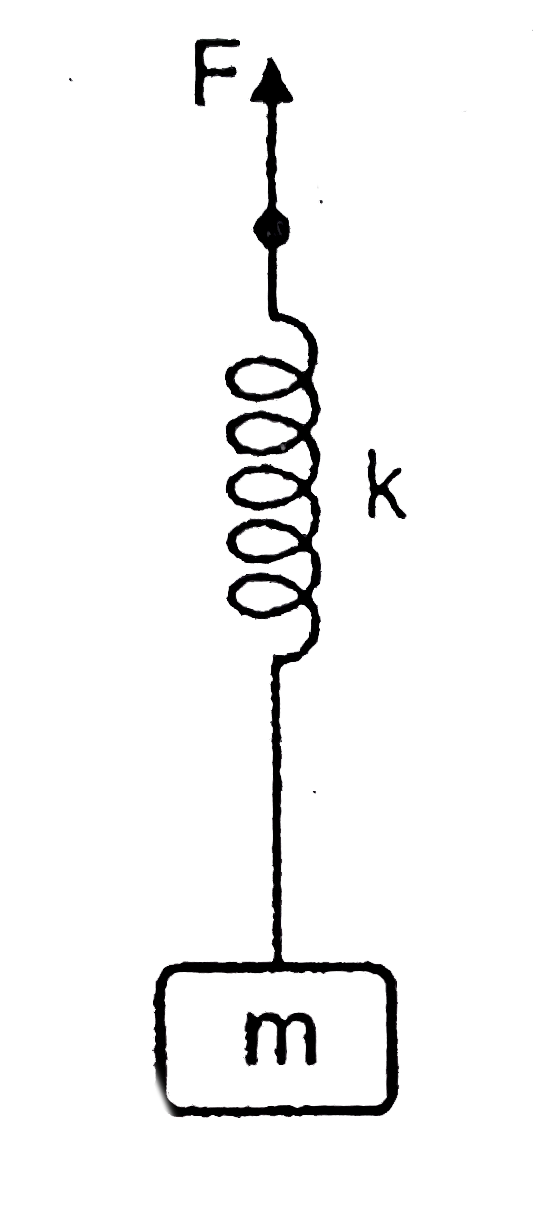
View SolutionA block of mass m is connected to a spring (spring constant k). Initially the block is at rest and the spring is in its natural length. Now the system is released in gravitational field and a variable force F is applied on the upper end of the spring such that the downward acceleration of the block is given as a=g−αt, where t is time elapses and α=1m/s2, the velocity of the point of application of the force is:
View SolutionIn the figure, block A is released from rest when the spring is its natural length for the block B of mass m to leave contact with the ground at some stage what should be the minimum mass of block A?
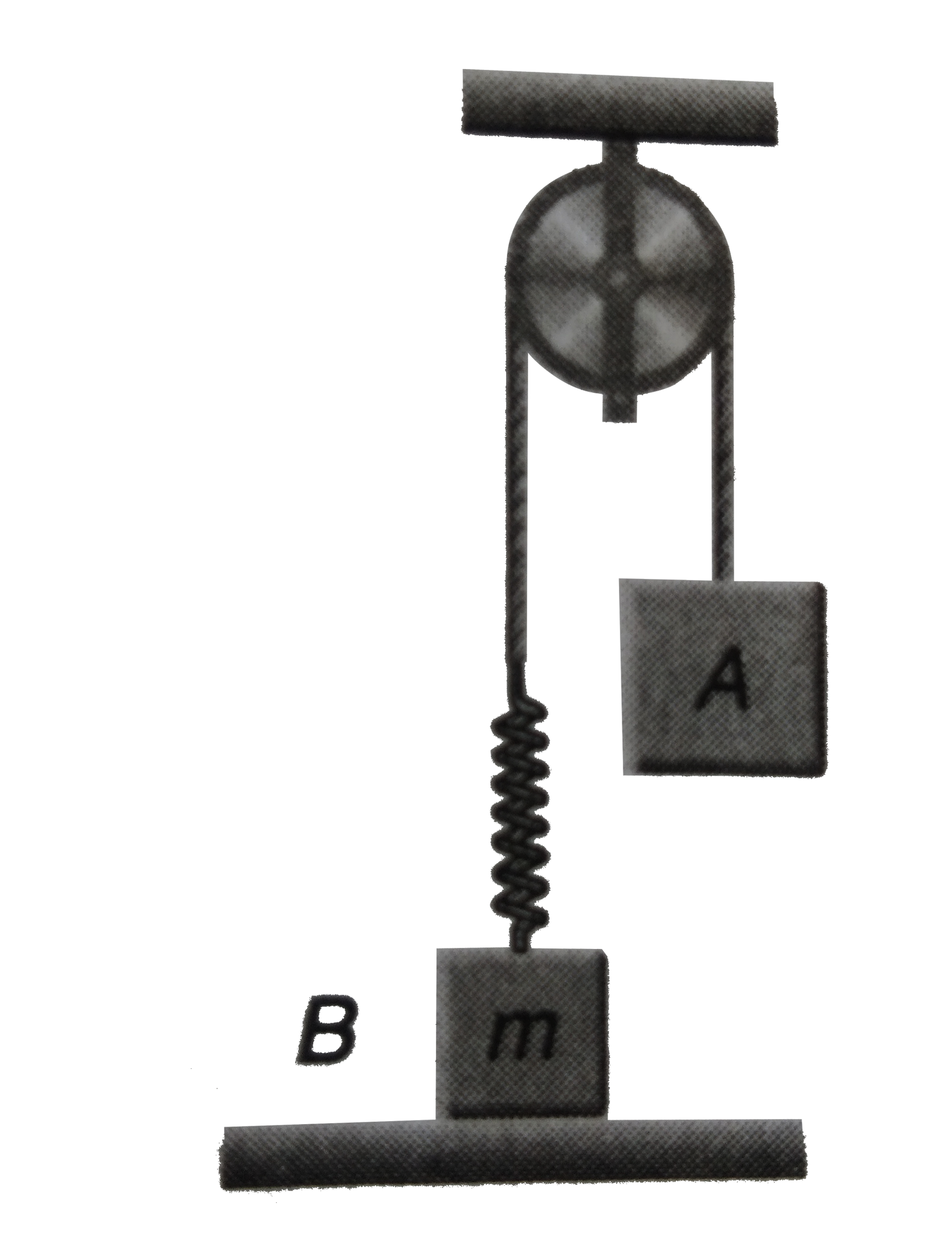 .
.
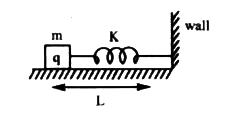
View SolutionA point mass m and charge q is connected with a spring of negligible mass with natural length L. Initially spring is in its natural length. Now a horizontal uniform electric fiedl E is switched on as shown. Find
(a) the maximum separation between the mass and the wall
(b) Find the separation of the point mass and wall at the equilibrium position of mass.
(c ) FInd the enery stored in the spring at the equilibrium position of the point mass
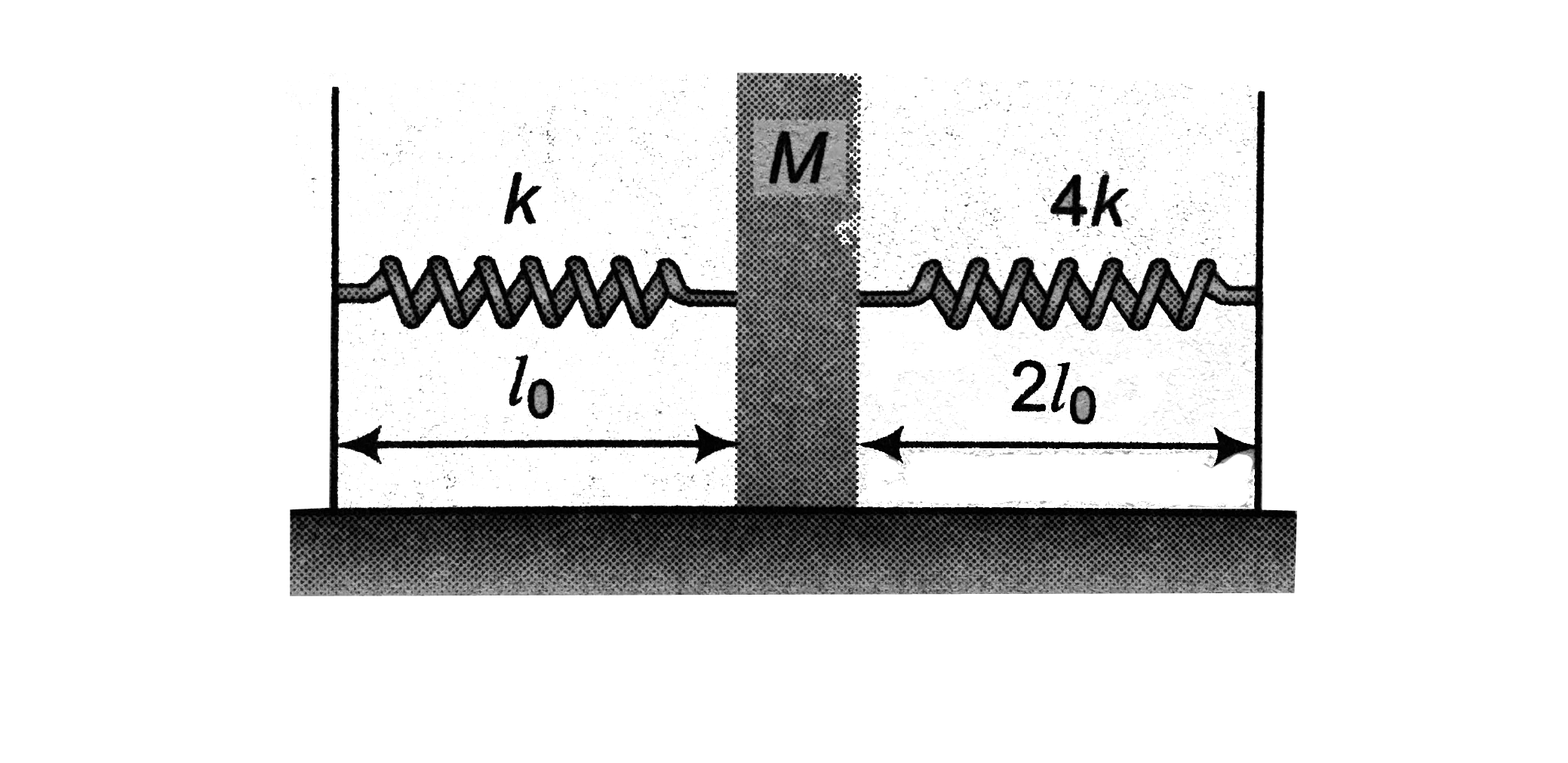
View SolutionA block of mass M is kept on a smooth surface and touches the two springs as shown in the figure but not attached to the springs. Initially springs are in their natural length. Now, the block is shifted (l0/2) from the given position in such a way that it compresses a spring and released. The time - period of oscillation of mass will be
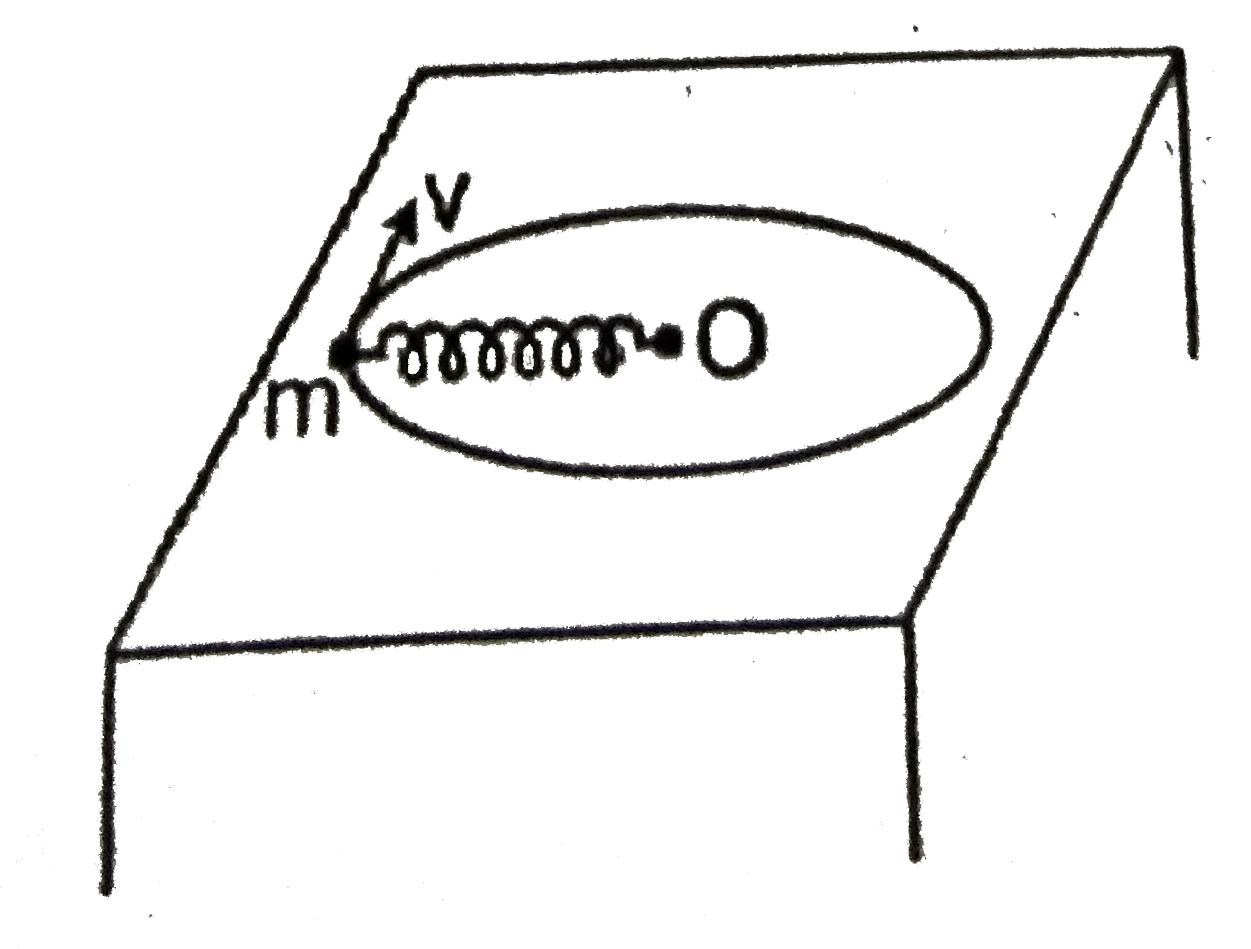
View SolutionMass m is connected with an ideal spring of natural length l whose other end is fixed on a smooth horizontal table. Initially spring is in its natural length l. Mass m is given a velocity 'v' perpendicular to the spring and released . The velocity perpendicular to the spring when its length l+x , will be
View SolutionFind the maximum tension in the spring if initially spring at its natural length when block is released from rest.

View Solution
