In the above problem, the charges on different surfaces if a charge q is placed at the centre of the shell with all other conditions remaining the same.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Total charges on $A + C$ is $3 q$ . Therefore,
$- q + q_{1} - q_{2} + q_{3} = 3 q$ ………i
$V_{B} = 0$
$∴ k (\frac{q_{1} - q}{2 R}) + k (\frac{q_{2} - q_{1}}{2 R}) + k (\frac{q_{3} - q_{2}}{3 R}) = 0$ ………ii
$V_{A} = V_{C}$
$∴ k (\frac{q_{1} - q}{R}) + k (\frac{q_{2} - q_{1}}{2 R}) + k (\frac{q_{3} - q_{2}}{3 R})$
$= k (\frac{q_{1} - q}{3 R} + k (\frac{q_{2} - q_{1}}{3 R}) + k (\frac{q_{3} - q_{2}}{3 R})$ .....iii
in the above equations $k = \frac{1}{4 π ε_{0}}$
solving above there equations we can finnd $q_{1}, q_{2}$ and $q_{3}$ .

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTRIC CHARGES AND FIELDS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A charge $Q$ is placed at the centre of a spherical conducting shell. Choose the correct option.
AOnly $- Q$ will appear on its inner surface
BOnly $+ Q$ will appear on its outer surface
CA charge $- Q$ will appear on its surface and $+ Q$ on its outer surface
DA charge $+ Q$ will appear on its inner surface and $- Q$ on its outer surface
Question 2 - Select One
A thin metallic spherical shell contains a charge $Q$ on it. A point charge $q$ is placed at the center of the shell and another charge $q_{1}$ is placed outside it as shown in figure. All the three charges are positive

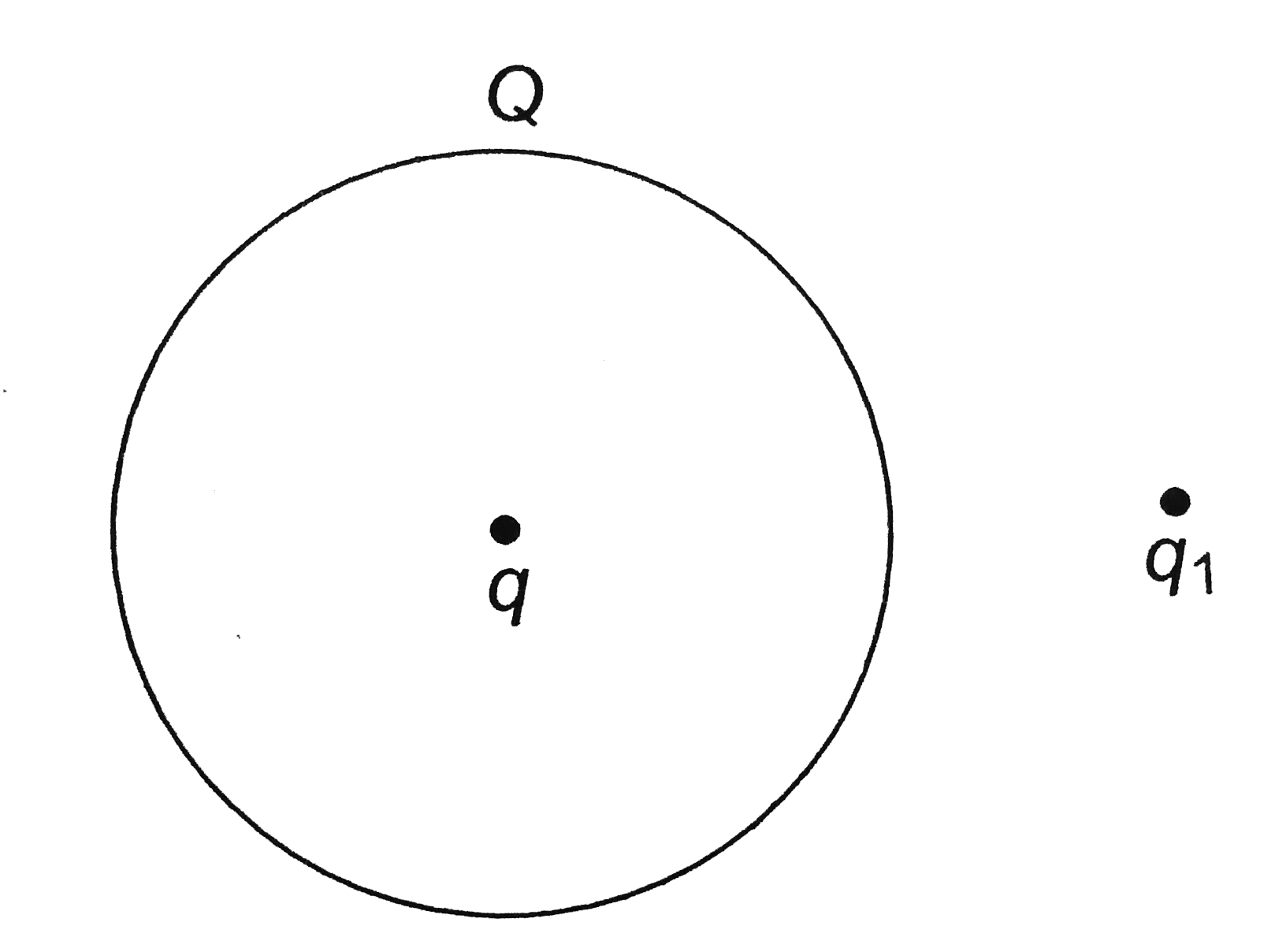
The force on the charge at the centre is
Atowards left
Btowards right
Cupward
Dzero
Question 3 - Select One
A thin. Metallic spherical shell contains s charge Q on it. A point charge q is placed at the centre of the shell and another charge $q_{1}$ is placed outside it as shown in figure (30-Q1). All the three charges are positive. The force on the charge at the centre is

Atowards left
Btowards right
Cupward
Dzero.

DC PANDEY-ELECTROSTATICS-Level 1 Subjective

Home
Ask Doubt
Profile