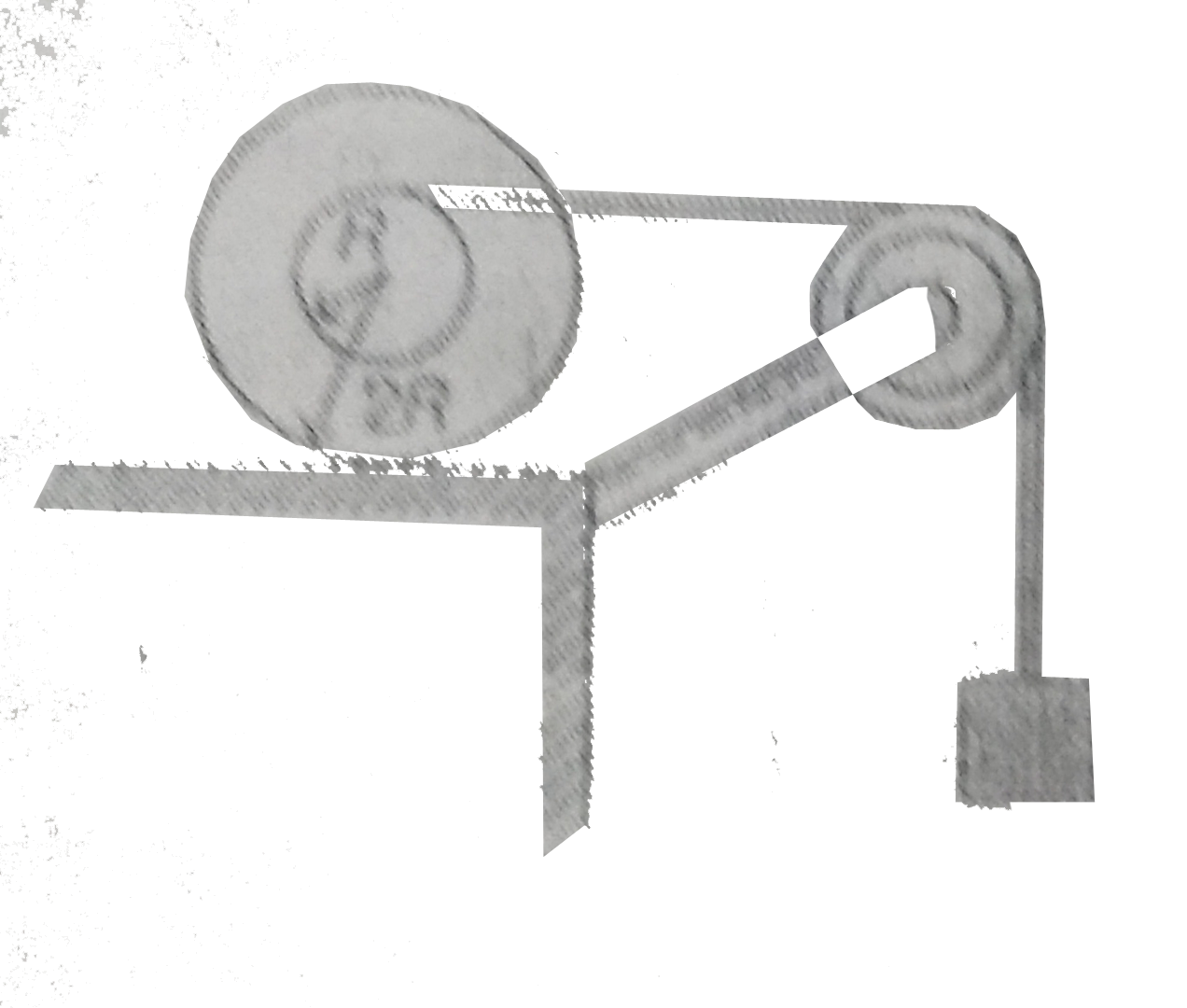
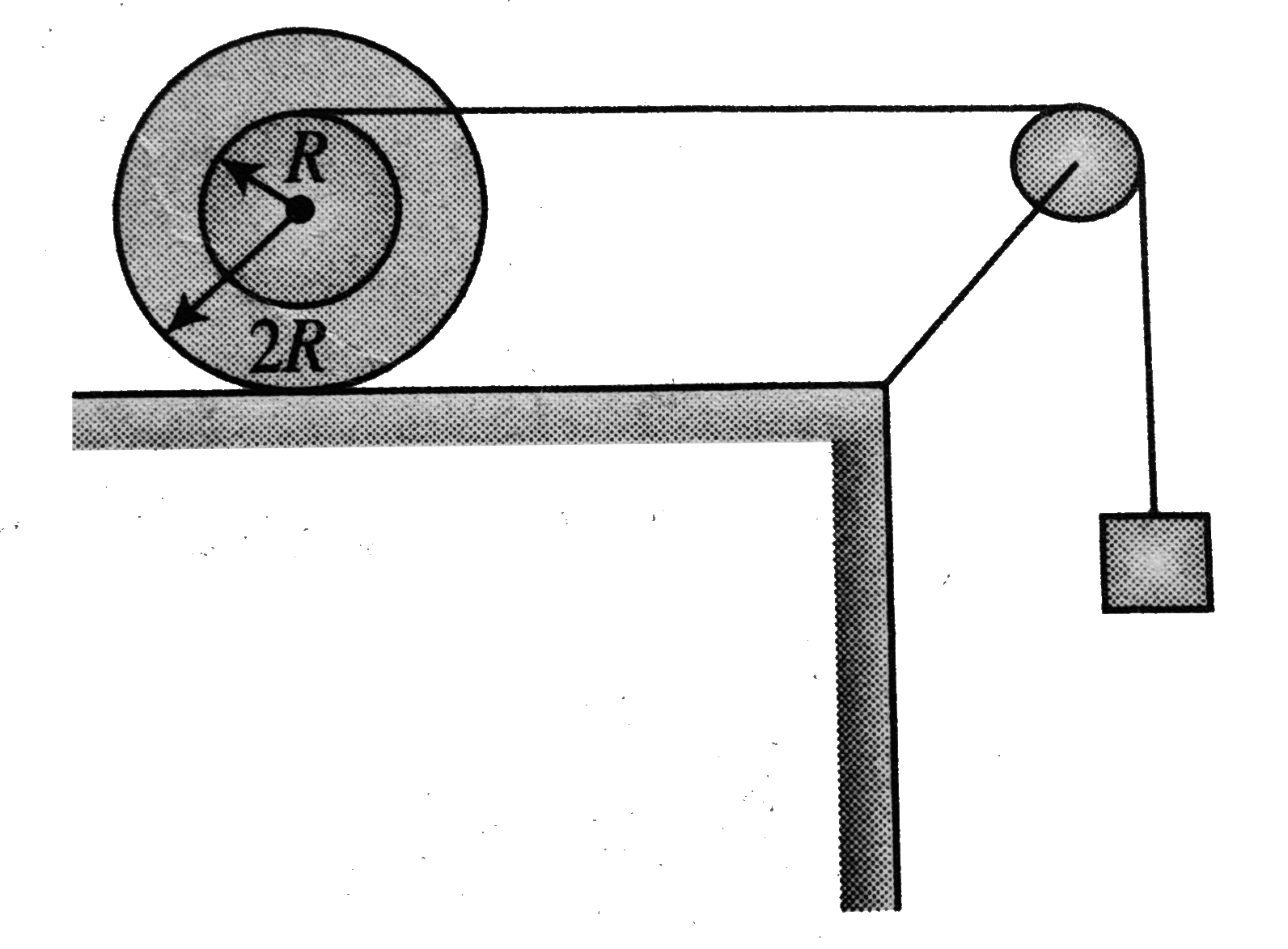
In the figure shon mass of both, the spherical body and blocks is m. Moment of inertia of the spherical body about centre of mass is 2mR2. The spherical body rolls on the horizontal surface. There is no sliiping between any two surfaces in contact. The ratio of kinetic energy of the spherical body to that of block is
34
13
23
12
The correct Answer is:C
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - DC PANDEYCHAPTER - ROTATIONAL MOTIONEXERCISE - More than one option is correct
36 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEYCHAPTER - ROTATIONAL MOTIONEXERCISE - Comprehension Type Questions
41 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEYCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions
2 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEYCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
Similar Questions
In the figure shown mass of both, the spherical body and block is m. Moment of inertia of the spherical body about centre of mass is 2mR2. The spherical body rolls on the horizontal surface. There is no slipping at any surfaces in contact. The ratio of kinetic energy of the spherical body to that of block is
View SolutionA body is in pure rolling over a horizontal surface. If the rotational kinetic energy of that body is 40% of its total kinetic energy, then the body is
View Solution
Knowledge Check
In the figure shown mass of both, the spherical body and block is m. Moment of inertia of the spherical body about centre of mass is 2mR2. The spherical body rolls on the horizontal surface. There is no slipping at any surfaces in contact. The ratio of kinetic energy of the spherical body to that of block is
 A3/4B1/3C2/3D1/2
A3/4B1/3C2/3D1/2For a body rolling along a level surface, without slipping the translational and rotational kinetic energies are in the ratio 2:1. The body is
AHollow sphereBsolid cylinderCringDsolid sphereA body of mass m kept on the floor of a lift moving downwards is pulled horizontally. If μ is the coefficient of friction between the surface in contact, then
Africtional resistance offered by the floor is 2 μmg, when lift moves up with a uniform velocity of 5 m/s.Brictional resistance offered by the floor is μumg, when lift moves lift moves up with uniform velocity of 3 m/s.Cfrictional resistance offered by the floor is 4.8μmg, when lift accelerates down with an acceleration of 4.8m/s2Dfrictional resistance offered by the floor must lie in the range 0≤f<∞
A body is in pure rolling over a horizontal surface. If the rotational kinetic energy of that body is 40% of its total kinetic energy, then the body is
View Solution" The potential energy of a body of mass "m" on the surface of earth is "
View SolutionIn the given figure F=10N,R=1m, mass of the body is 2kg and moment of inertia of the body about an axis passing through O and perpendicular to the plane of the body is 4kgm2. O is the centre of mass of the body.
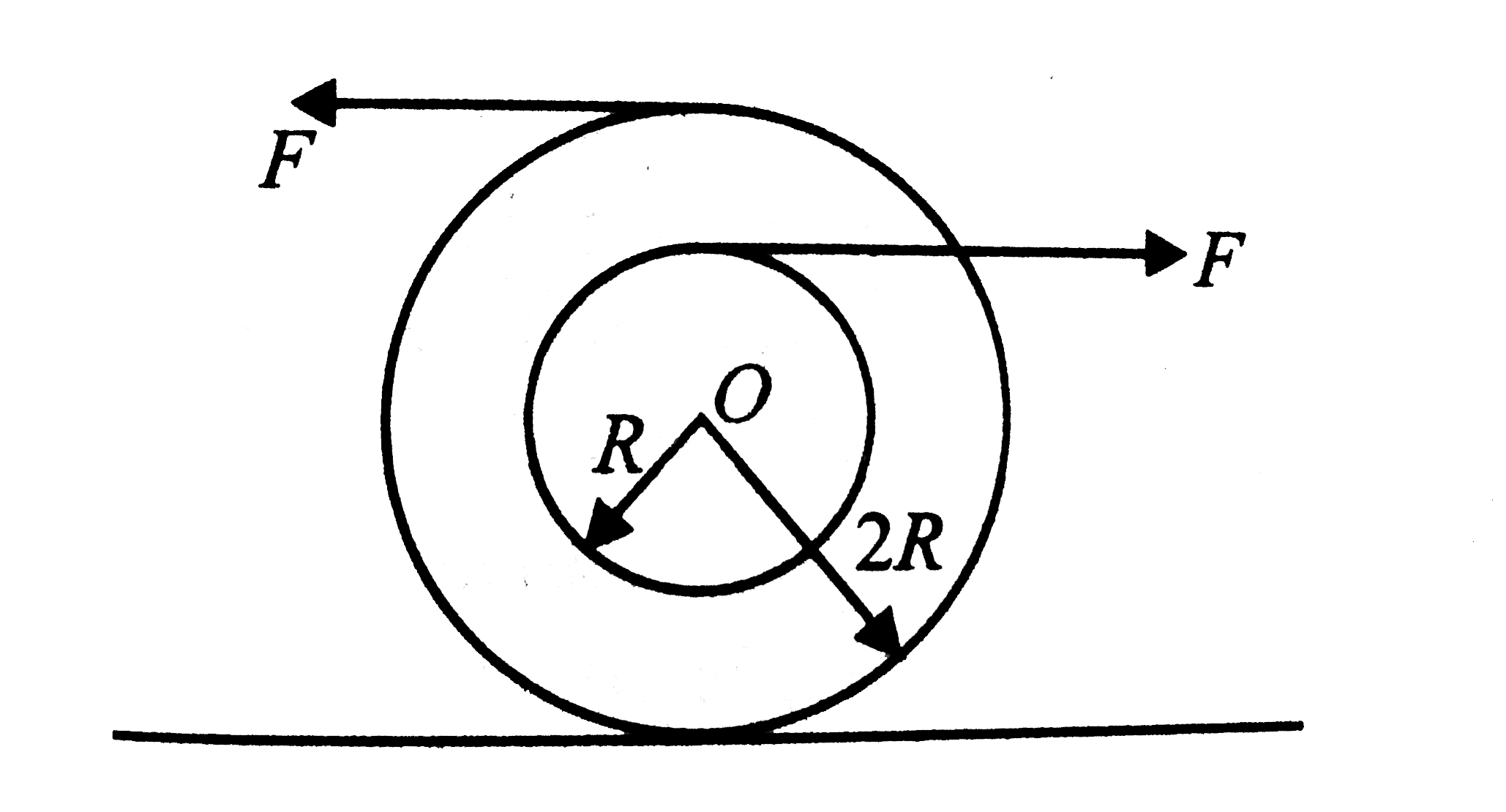
If the ground is sufficiently rough to ensure rolling, what is the kinetic energy of the body now in the given time interval of 2s?
View SolutionA body of mass m is placed at the centre of the spherical shell of radius R and mass M. The gravitation potential on the surface of the shell is
View SolutionIn the given figure F=10N,R=1m, mass of the body is 2kg and moment of inertia of the body about an axis passing through O and perpendicular to the plane of the body is 4kgm2. O is the centre of mass of the body.

If the ground is sufficiently rough to ensure rolling, what is the kinetic energy of the body now in the given time interval of 2s?
View Solution
