In the following two exercises, choose the correct answer from among the given ones: The gravitational intensity at the centre of a hemispherical shell of uniform mass density has the direction indicated by the arrow (see Fig.) (i) a, (ii) b, (iii) c, (iv) 0.
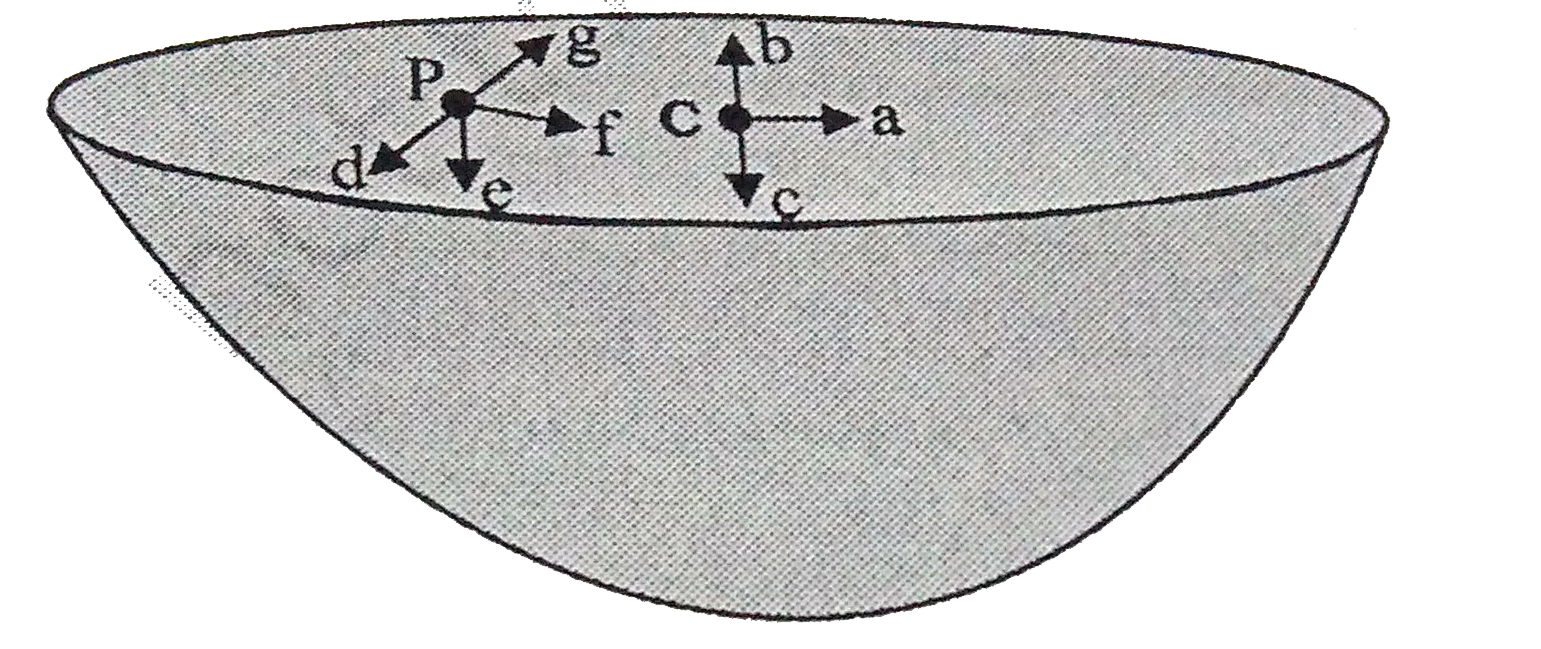
At all points inside a hollow spherical shell, potential is same. So, gravitational intensity, which is negative of gravitational potential gradient, is zero. Due to zero gravitational intensity, the gravitational forces acting on any particle at any point inside a spherical shell will be symmetrically placed. It follows from here that if we remove the upper hemispherical shell, the net gravitational force acting on a particle at P will be downwards. Since gravitational intensity is gravitational force per unit mass therefore, the direction of gravitational intensity will be along c. So, option (iii) is correct.
Topper's Solved these Questions
Similar Questions
The gravitation intensity at the centre C of the drumhead defined by a hemispherical shell has the direction indicated by the arrow [see Fig. 8.46]
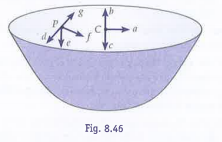
View SolutionThe gravitation intensity at the centre C of the drumhead defined by a hemispherical shell has the direction indicated by the arrow [see Fig. 8.46]

View Solution
Knowledge Check
The direction of gravitational intensity at point P of a hemispherical shell of uniform mass desity is indicated by the arrow
 AdBeCfDg
AdBeCfDgConsider the following name of contemporaries of Kanishka and select the correct answer from codes given below:
(i) Ashvaghosha
(ii) Vasumitra
(iii) Kalidas
(iv) KambanAI and IVBII and IIICI and IIDAll of these
For the above problem, the direction of the gravitational intensity at an arbitrary point P is indicated by the arrow (i) d, (ii), e, (iii) f (iv) g.
View SolutionFor the above problem, the direction of the gravitational intensity at an arbitrary point P is indicated by the arrow (i) d, (ii), e, (iii) f (iv) g.
View SolutionFrom the following sets choose the correct representations.
(i) K.G for kilogram
(ii) C for degree Celsius
(iii) m for metre
(iv) s for second
View SolutionFor a given uniform spherical shell of mass M and radius R find gravitational field at a distancer r from centre in following two cases (a) r≥R (b) r<R .
View SolutionGiven the location of the centre of mass of a (i) sphere, (ii) cylinder, (iii) ring, and (iv) cube, each of uniform mass density. Does the centre of mass of a body necessarily lie on the body ?
View SolutionA small conducting spherical shell with inner radius a and outer radius b is concentric with a larger conducting sphereical shell with inner radius c and outer radius d. The inner shell has total charge +2q and the outer shell has charge +4q.
a. Make a plot of the magnitude of the electric field versus r.
b. Calculate the electric field (magnitude and direction in terms of q) and the distance r from the common centre of the two shell for (i)r<a,(ii)a<r<b,(iii)bltrltc,(iv)cltrltd,(v)rgtd.Showuourrest–∈agraphwithradialcompo≠ntofvecEasfunctionofr.
c. What is the total charge on the
i. inner surface of the small shell,
ii. outer surface of the small shell,
iii. inner surface of the large shell,
iv. outer surface of the large shell?
View Solution
