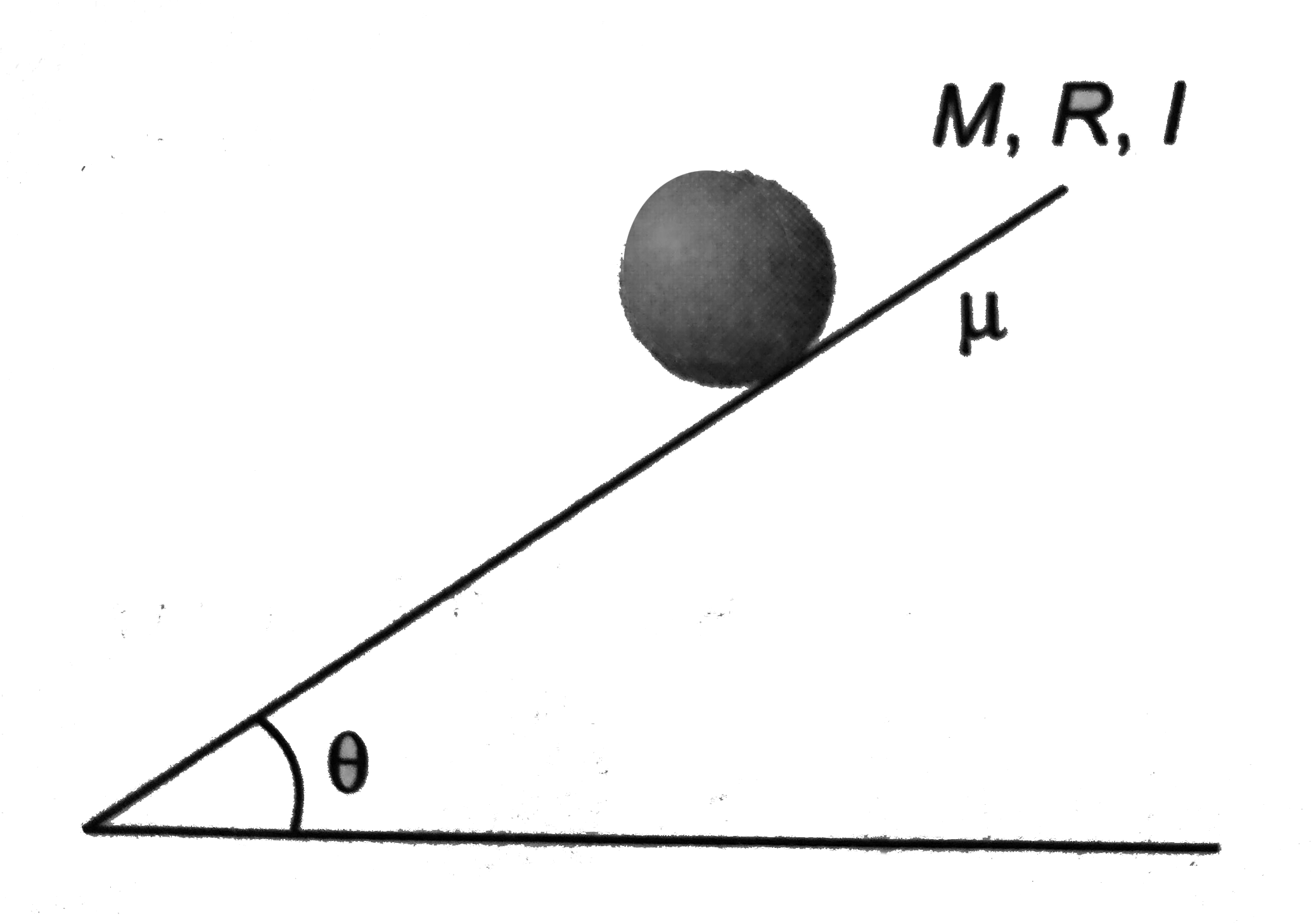
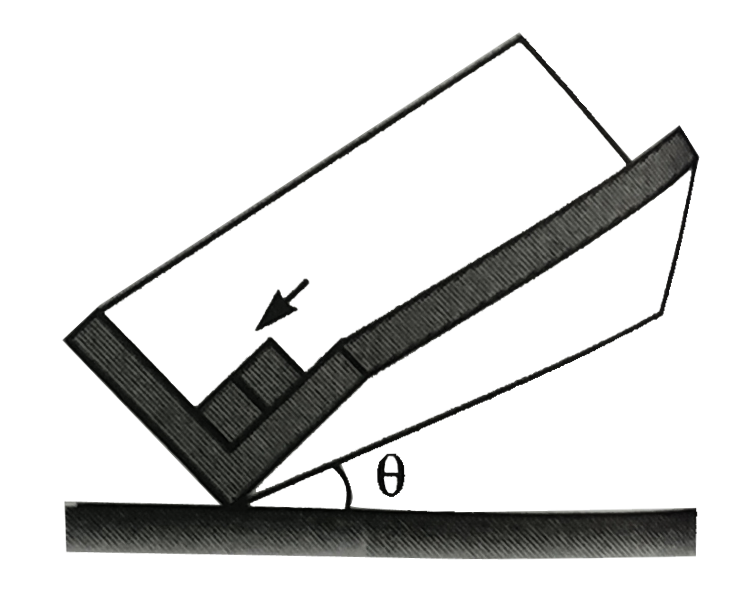
In the shown figure, M is mass of the body, R its radius an I the moment of inertial about an axis passing through centre. Find force of friction f acting on the body (upwards), its linear acceleration a (down the plane) and type of motion if:
(a) μ=0
(b). μ<μmin
(c). μ>μmin
Where μmin is the minimum value of coefficient of friction required for pure rolling
(a). If μ=0 then
f=0 and a=gsinθ=a1 (say)
and the motion is only translational.
(b). If μ<μmin then maximum value of friction will act. As friction is insufficient to provide acclerated pure rolling or to stop the relative motion. ltbr> ∴f=fmax=μmgcosθ
and a=Mgsinθ−μMgcosθm
=gsinθ−μgcosθ=a2 (say)
In this case, motion is rotation + translatio with forward slip (as a>Rα)
(c). if μ>μmin, then we have discussed in the above article that
f=Mgsinθ1+MR2I⎞⎟⎠ and a=gsinθ1+IMR2=a3 (say)
Motion i this case is rotation + translation with accelerated pure rolling.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Solved Examples25videos
ROTATIONAL MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Miscellaneous Examples2videos
ROTATIONAL MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises39videos
ROTATION
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions17videos
ROTATIONAL MOTION
Similar Questions
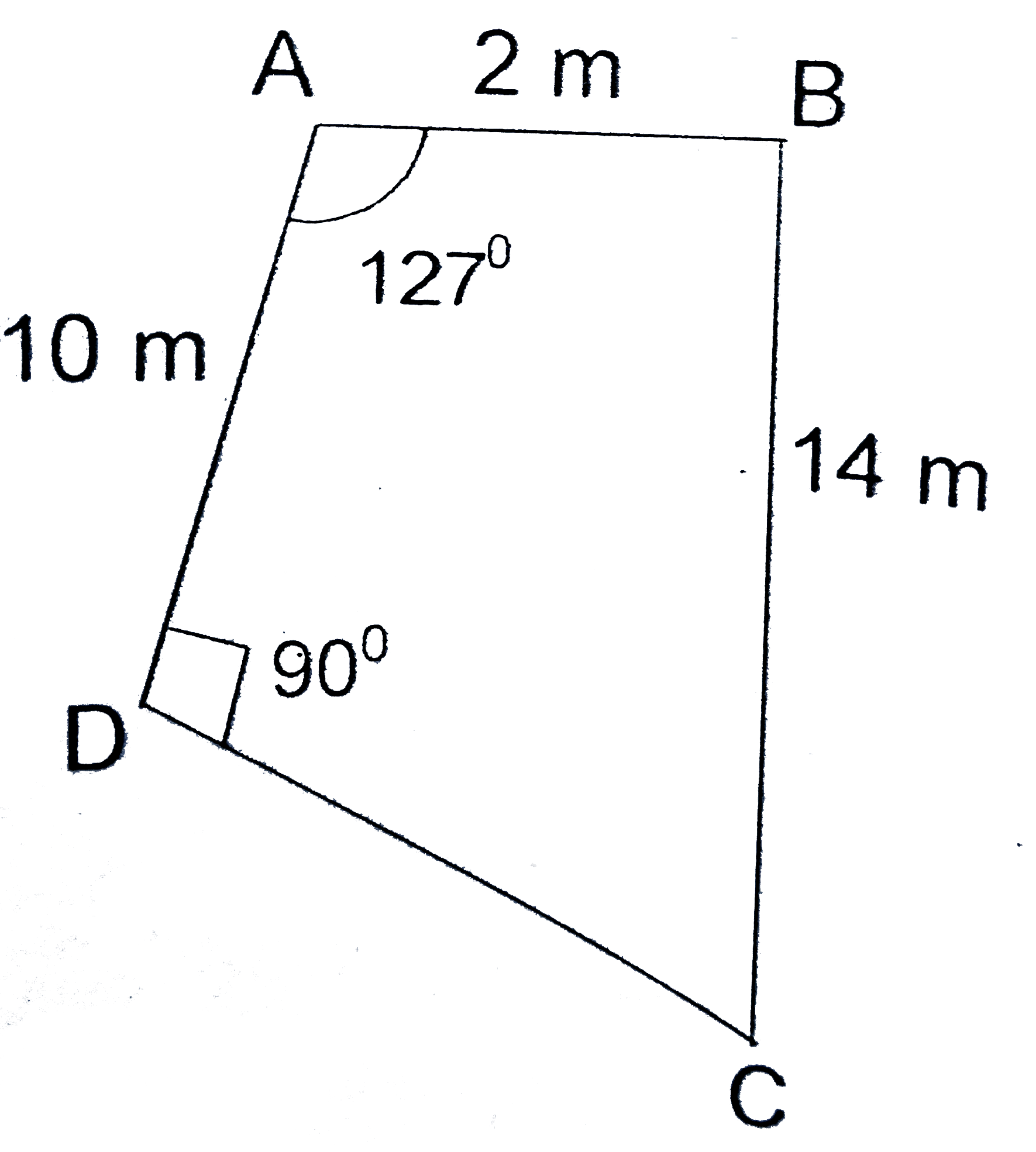
In the figure shown ABCD is a lamina of mass 12kg. The moment of inertia of the given body about an axis passing through D, and perpendicular to the plane of the body is
 View Solution
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
View Solution
For the given dimensions shown in figure, find critical value of coefficient of friction μView Solution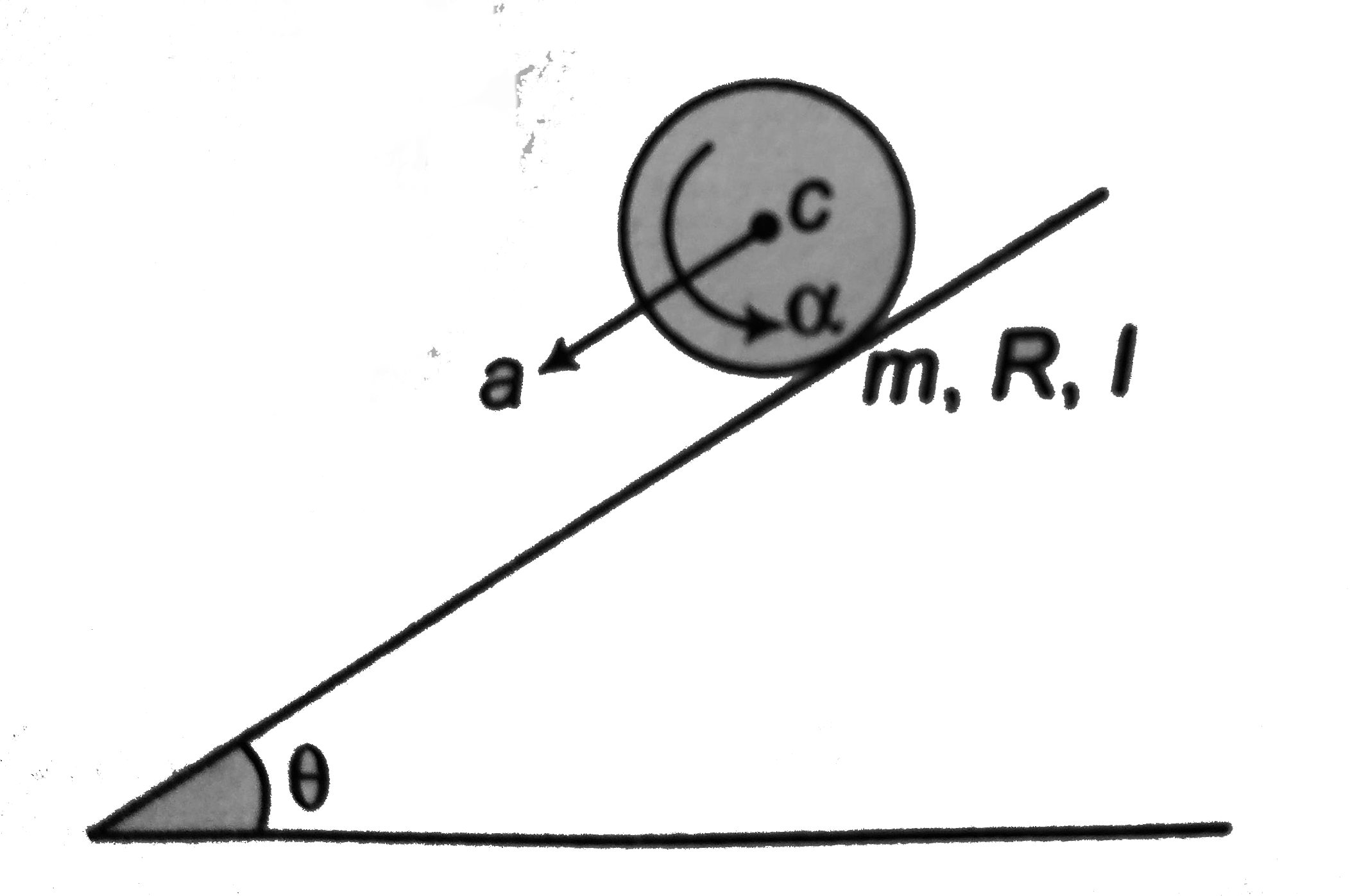
A body of mass m, radius R and moment of inertia I (about an axis passing through the centre of mass and perpendicular to plane of motion) is released from rest over a sufficiently rough ground (to provide accelerated pure rolling) find linear acceleration of the body.View SolutionFind the moment of inertia of a uniform cylinder about an axis through its centre of mass and perpendicular to its base. Mass of the cylinder is M and radius is R.
View SolutionDerive an expression for the acceleration of a solid cylinder rolling without slipping down an inclined plane. Also find the minimum coefficient of friction required for pure rolling
View SolutionIn the given figure F=10N,R=1m, mass of the body is 2kg and moment of inertia of the body about an axis passing through O and perpendicular to the plane of the body is 4kgm2. O is the centre of mass of the body.
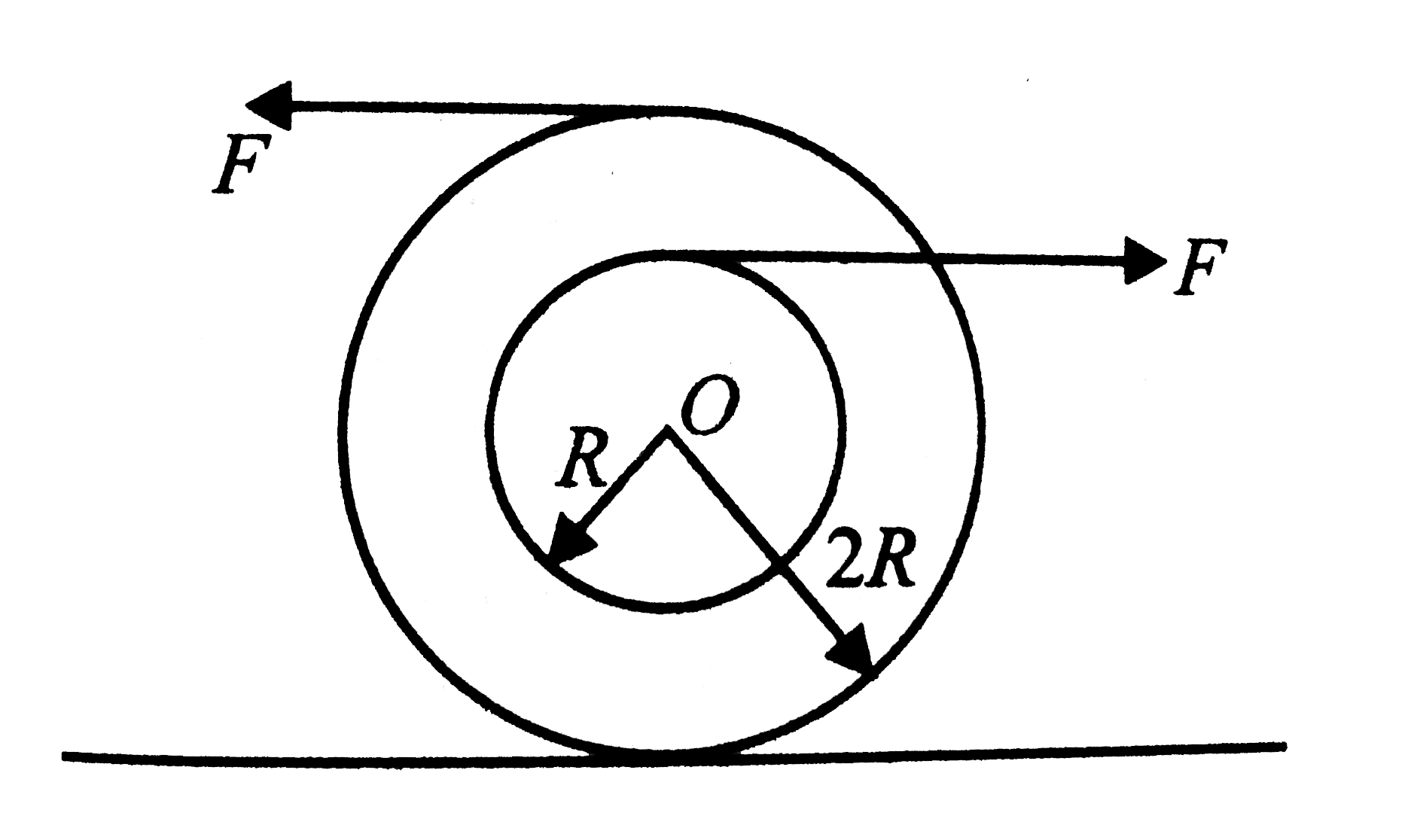
If the ground is smooth, what is the total kinetic energy of the body after 2s?View SolutionAssertion: Moment of inertia of a rigid body about any axis passing through its centre of mass is minimum
Reason: From theorem of parallel axis
I=Icm+Mr2View SolutionPoint C is the centre of mass of the rigid body shown in figure. Find the total torque acting on the body about point C.
 View Solution
View SolutionA block of mass m sides down an inclined right angled trough .If the coefficient of friction between block and the trough is μk acceleration of the block down the plane is
 View Solution
View Solution

