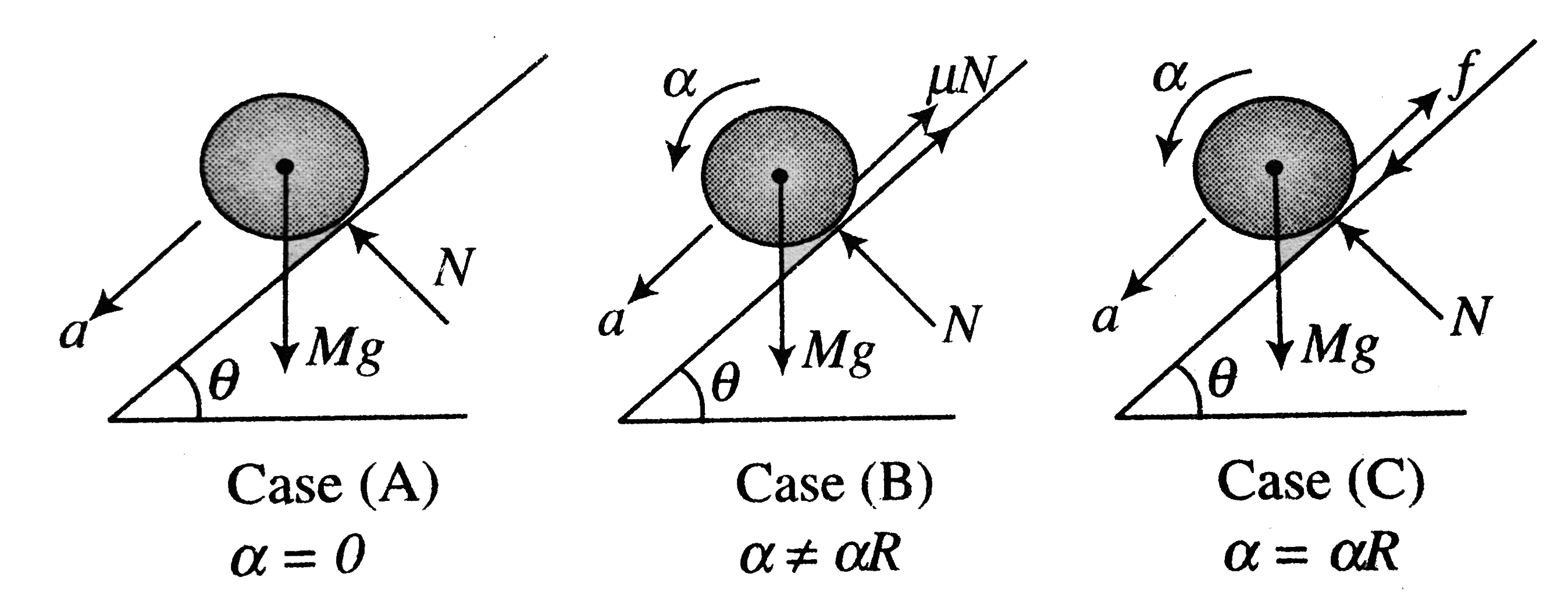
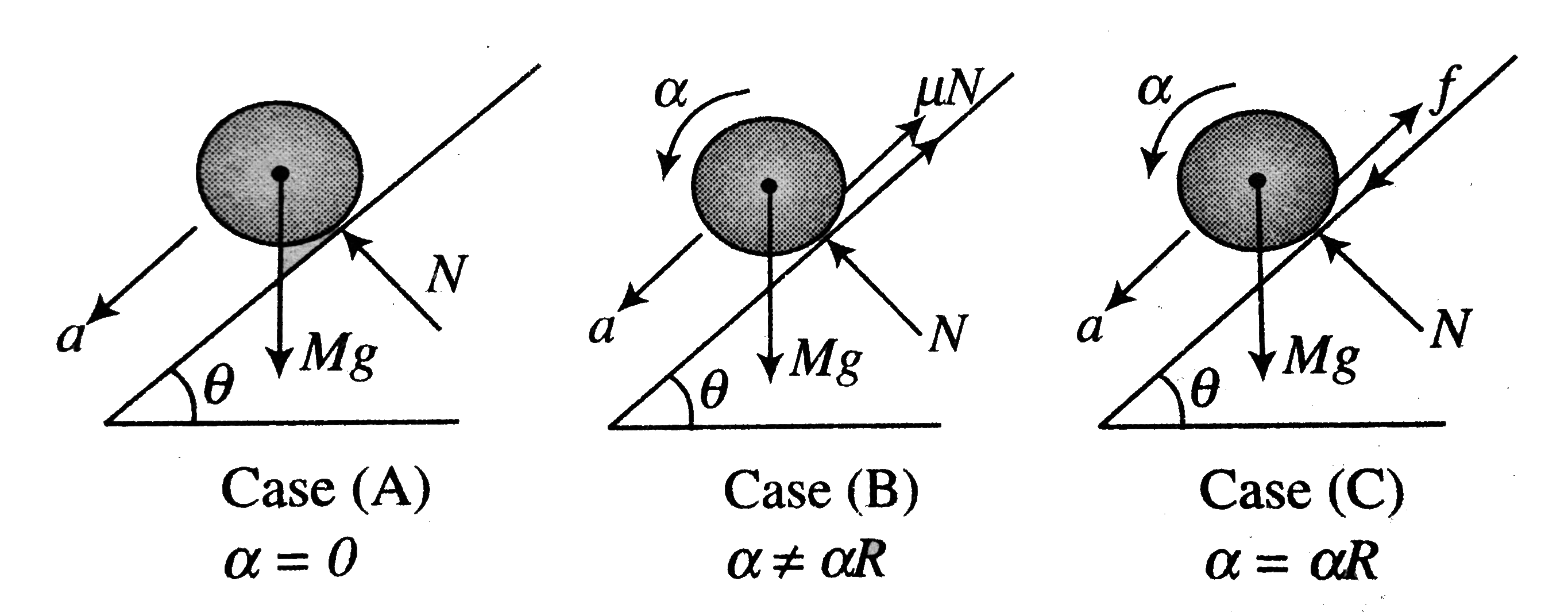
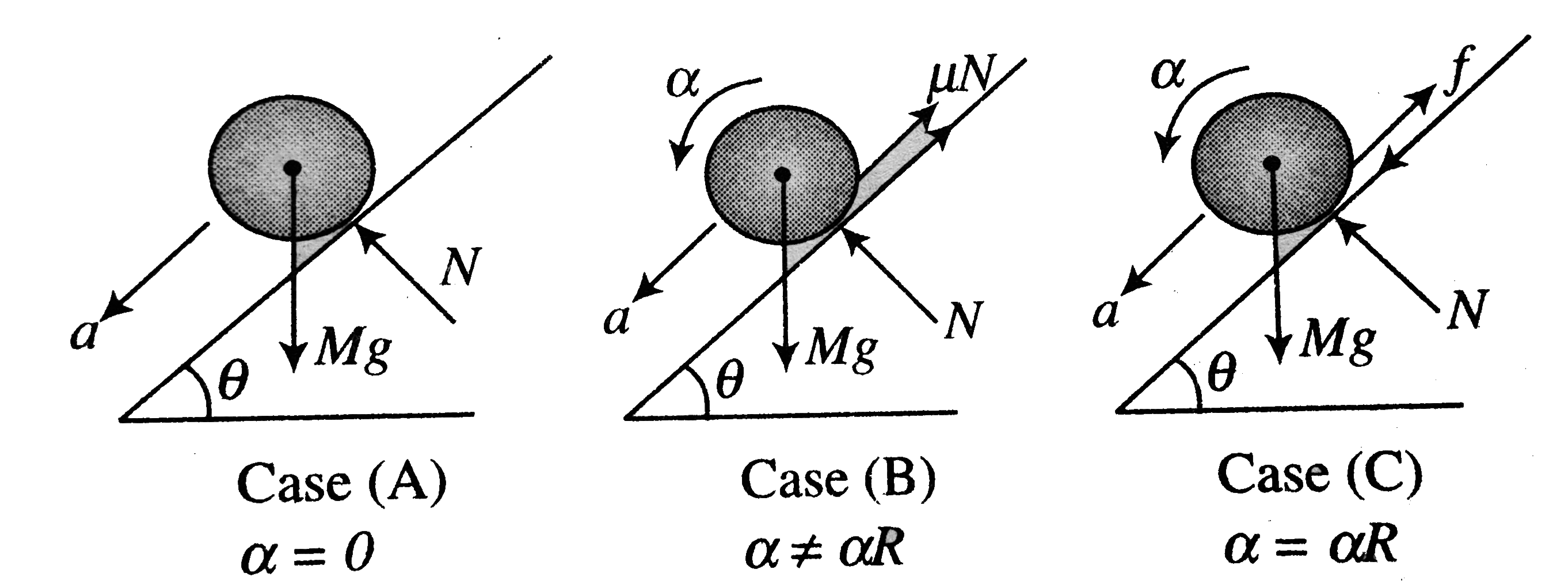
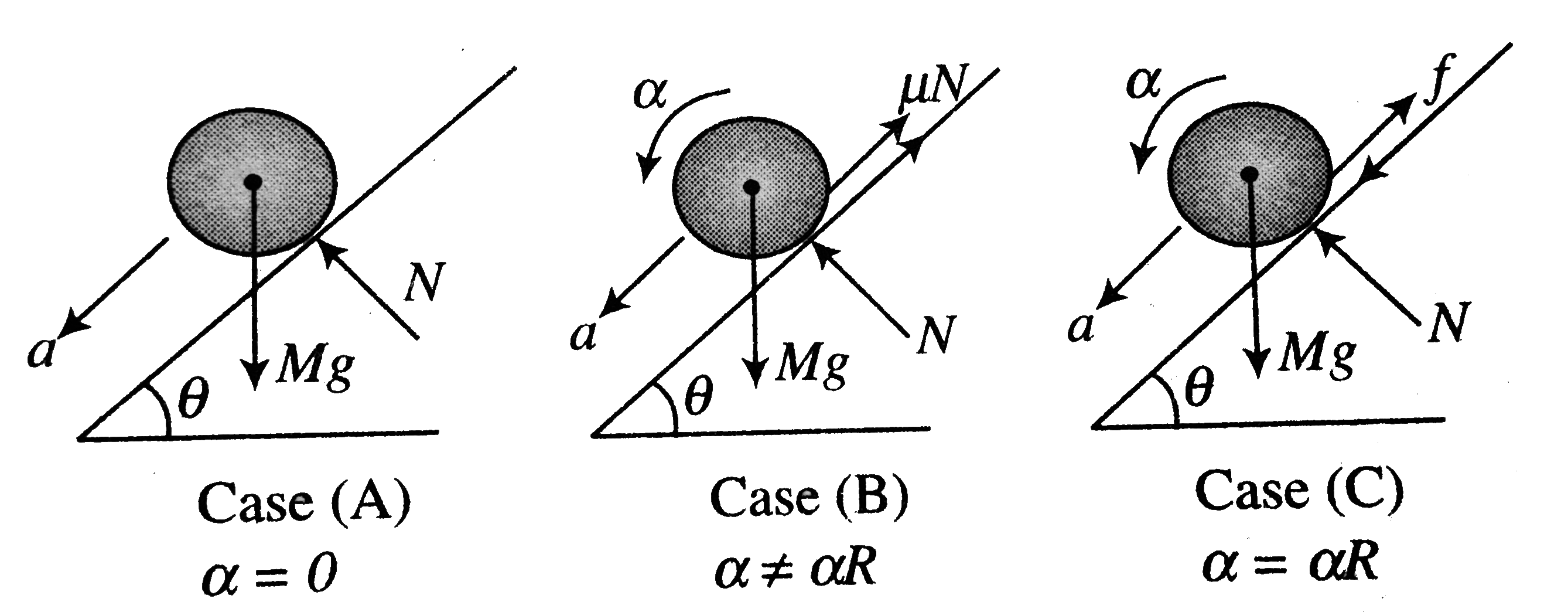
In this passage a brief idea is given of the motion of the rolling bodies on an inclined plane. We will consider three cases: objects are released on an incline plane
Case-A: which is smooth.
Case-B: where friction is insufficient to provide pure rolling.
Case-C: where friction is sufficient to provide pure rolling. Force diagram for three cases are as follows: (where symbols have their usual meanings)

We have four objects: a solid sphere, a hollow sphere, a ring and a disc, all of same radius. When these are released on an inclined plane, it may happen that all of them do not perform pure rolling. But from the information of pure rolling, if one object can be confirmed to be purely rolling then it can be said that rest all will perform pure rolling. This object whose pure rolling confirms pure rolling of all other objects is

hollow sphere

solid sphere

ring

disc

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

From passage for case $c$
$μ_{min} = \frac{tan θ (\frac{k^{2}}{R^{2}})}{(1 + \frac{k^{2}}{R^{2}})} \to (pure rolling)$
Putting the values of $k$ for different objects given in the table (in passege) we get
$μ_{min (ring)} = \frac{tan θ}{2}$
$μ_{min (Disc)} = \frac{tan θ}{3}, μ_{min (solid sphere)} = \frac{2}{3} tan θ$
$μ_{min (Hollow sphere) = \frac{2}{7} tan θ}$
$\Rightarrow μ_{min (Ring)},$ is greater than either of
$μ_{min (Ring)}, μ_{min (Solid Sphere)}, μ_{min (Hollow sphere)}$
Therefore, the pure rolling of ring will confirm pure rolling of all other bodies.

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
In this passage a brief idea is given of the motion of the rolling bodies on an inclined plane. We will consider three cases: objects are released on an incline plane
Case-A: which is smooth.
Case-B: where friction is insufficient to provide pure rolling.
Case-C: where friction is sufficient to provide pure rolling. Force diagram for three cases are as follows: (where symbols have their usual meanings)

Three solid uniform spheres are released on an inclined plane as shown in the figure. The distance between the spheres remains constant during motion in:

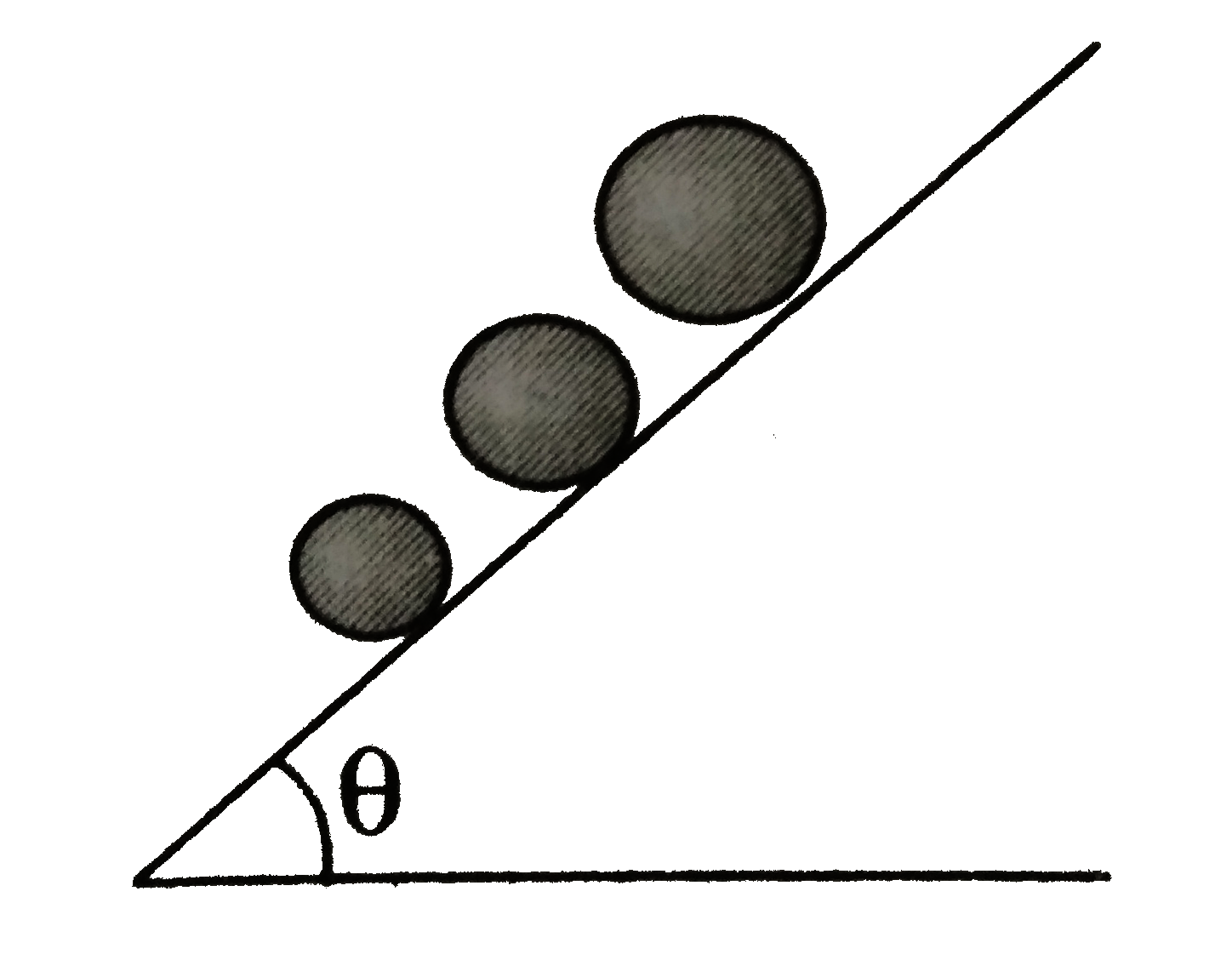Aall three masses
Bcase $A$ and $B$
Conly case $C$
Ddepends on the mass of the spheres
Question 2 - Select One
In this passage a brief idea is given of the motion of the rolling bodies on an inclined plane. We will consider three cases: objects are released on an incline plane
Case-A: which is smooth.
Case-B: where friction is insufficient to provide pure rolling.
Case-C: where friction is sufficient to provide pure rolling. Force diagram for three cases are as follows: (where symbols have their usual meanings)

If the four objects given in the above question are of same mass, same radius having the same friction coefficient and are released from the same height, then at the bottom the object which will have least kinetic energy for case $B$ will be the:
Ahollow sphere
Bsolid sphere
Cring
Ddisc
Question 3 - Select One
In this passage a brief idea is given of the motion of the rolling bodies on an inclined plane. We will consider three cases: objects are released on an incline plane
Case-A: which is smooth.
Case-B: where friction is insufficient to provide pure rolling.
Case-C: where friction is sufficient to provide pure rolling. Force diagram for three cases are as follows: (where symbols have their usual meanings)

Two children $A$ and $B$ use bicycles, having wheels of ring type and disc type respectively. During a race, bicycles are given the same velocity from the bottom of the inclined bridge to ascend the bridge without pedalling, then (assuming pure rolling).
Aboth bicycles with reach up to same height
Bbicycle of child $A$ will reach a greater height
Cbicycle of child $B$ will reach a greater height
Dbicycle of child $B$ will reach a greater height