In YDSE with d=1mm and D=1m, Slabs of (t=1μm,μ=3) and (t=0.5μm,μ=2) are introduced in front of upper and lower slits, respectively. Find the shift in the fringe pattern.
To solve the problem, we will follow these steps:
Step 1: Understand the setup
In Young's Double Slit Experiment (YDSE), we have two slits separated by a distance d=1mm=1×10−3m and a screen at a distance D=1m. Two slabs are introduced in front of the slits, which will affect the path difference of light coming from the slits.
Step 2: Identify the properties of the slabs
We have:
- Slab 1 (in front of the upper slit): thickness t1=1μm=1×10−6m and refractive index μ1=3.
- Slab 2 (in front of the lower slit): thickness t2=0.5μm=0.5×10−6m and refractive index μ2=2.
Step 3: Calculate the optical path difference introduced by the slabs
The optical path difference Δx introduced by each slab can be calculated using the formula:
Δx=(μ−1)t
For slab 1:
Δx1=(μ1−1)t1=(3−1)×1×10−6=2×10−6m
For slab 2:
Δx2=(μ2−1)t2=(2−1)×0.5×10−6=1×0.5×10−6=0.5×10−6m
Step 4: Calculate the net path difference
The net path difference Δxnet is given by:
Δxnet=Δx1−Δx2
Substituting the values:
Δxnet=2×10−6−0.5×10−6=1.5×10−6m
Step 5: Relate the path difference to fringe shift
In YDSE, the fringe shift y is related to the path difference by:
d⋅yD=Δxnet
Rearranging for y:
y=Δxnet⋅Dd
Step 6: Substitute the values to find the fringe shift
Substituting the known values:
y=1.5×10−6⋅11×10−3=1.5×10−3m=1.5mm
Final Answer
The shift in the fringe pattern is y=1.5mm.
---
In Young's Double Slit Experiment (YDSE), we have two slits separated by a distance d=1mm=1×10−3m and a screen at a distance D=1m. Two slabs are introduced in front of the slits, which will affect the path difference of light coming from the slits.
We have:
- Slab 1 (in front of the upper slit): thickness t1=1μm=1×10−6m and refractive index μ1=3.
- Slab 2 (in front of the lower slit): thickness t2=0.5μm=0.5×10−6m and refractive index μ2=2.
The optical path difference Δx introduced by each slab can be calculated using the formula:
Δx=(μ−1)t
For slab 1:
Δx1=(μ1−1)t1=(3−1)×1×10−6=2×10−6m
Δx2=(μ2−1)t2=(2−1)×0.5×10−6=1×0.5×10−6=0.5×10−6m
The net path difference Δxnet is given by:
Δxnet=Δx1−Δx2
Substituting the values:
Δxnet=2×10−6−0.5×10−6=1.5×10−6m
In YDSE, the fringe shift y is related to the path difference by:
d⋅yD=Δxnet
Rearranging for y:
y=Δxnet⋅Dd
Substituting the known values:
y=1.5×10−6⋅11×10−3=1.5×10−3m=1.5mm
The shift in the fringe pattern is y=1.5mm.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Subjective11videos
WAVE OPTICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Single Correct128videos
WAVE OPTICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Exercise 2.112videos
WAVE OPTICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - single correct Ansewer type12videos
SOURCES OF MAGNETIC FIELD
Similar Questions
In YDSE, let A and B be two slits. Films of thickness tA and tB and refractive μA and μB are placed in front of A and B, respectively. If μAtA=μAtB, then the central maxima will
View SolutionA monochromatic beam of light falls on YDSE apparatus at some angle (θ) as shown, a thin sheet of glas (R.I. = μ, thickness = t) is insdted in front of the lower slit S2. Select the correct alternative.
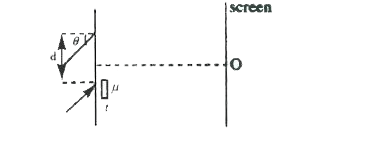 View Solution
View SolutionFigure shows a YDSE setup having identical slits S1 and S2 with d =5 mm and D = 1 m. A monochromatic light of wavelength λ=6000Å is incident on the plane of slit due to which at screen centre O, an intensity I0 is produced with fringe pattern on both sides Now a thin transparent film of 11μm thickness and refractive index μ=2.1 is placed in front of slit S1 and now interference patten is observed again on screen.
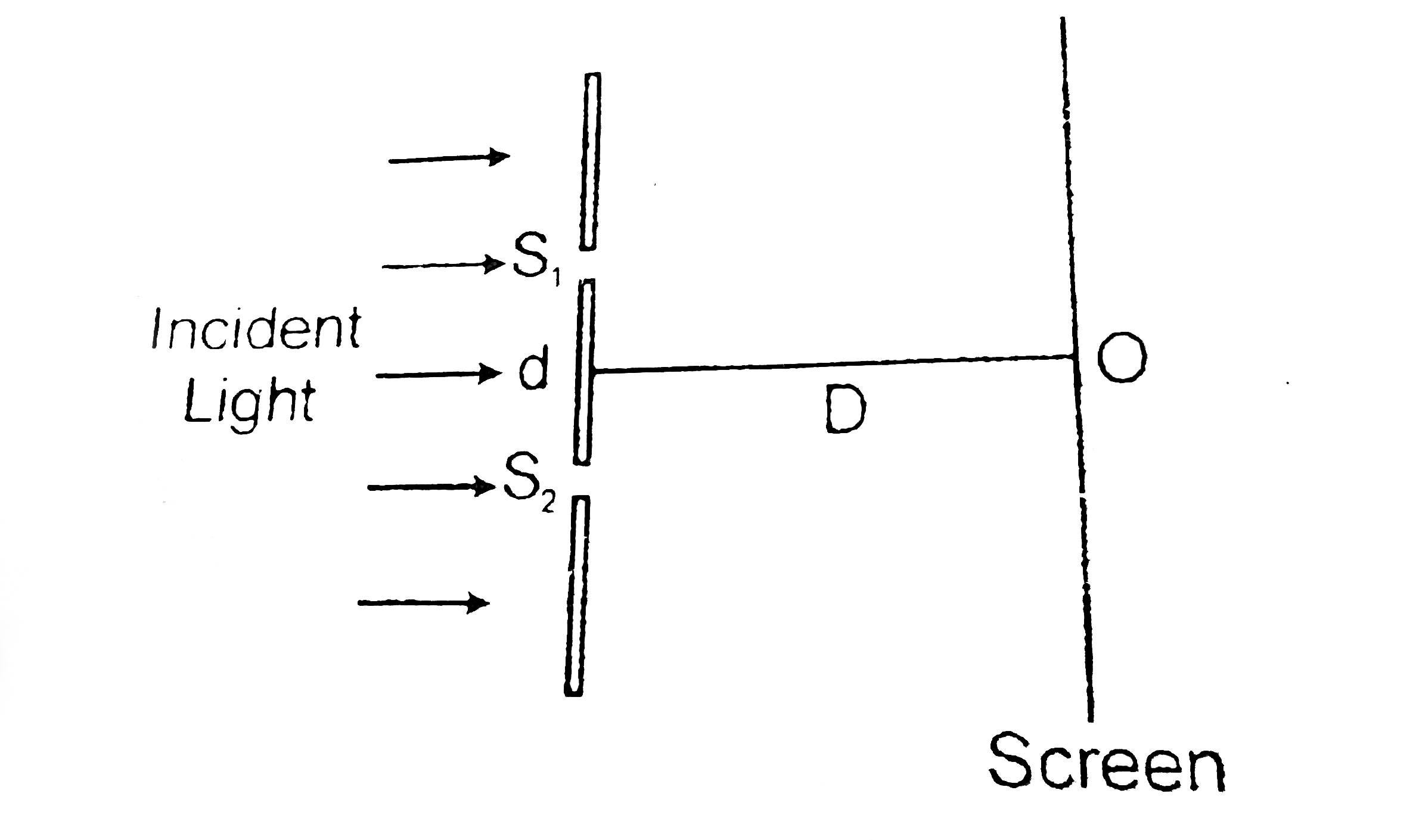
Due to placement of film in front of S1, how many bright fringes cross the point O of screen excluding the one which was at O earlier :View SolutionFigure shows a YDSE setup having identical slits S1 and S2 with d =5 mm and D = 1 m. A monochromatic light of wavelength λ=6000Å is incident on the plane of slit due to which at screen centre O, an intensity I0 is produced with fringe pattern on both sides Now a thin transparent film of 11μm thickness and refractive index μ=2.1 is placed in front of slit S1 and now interference patten is observed again on screen.
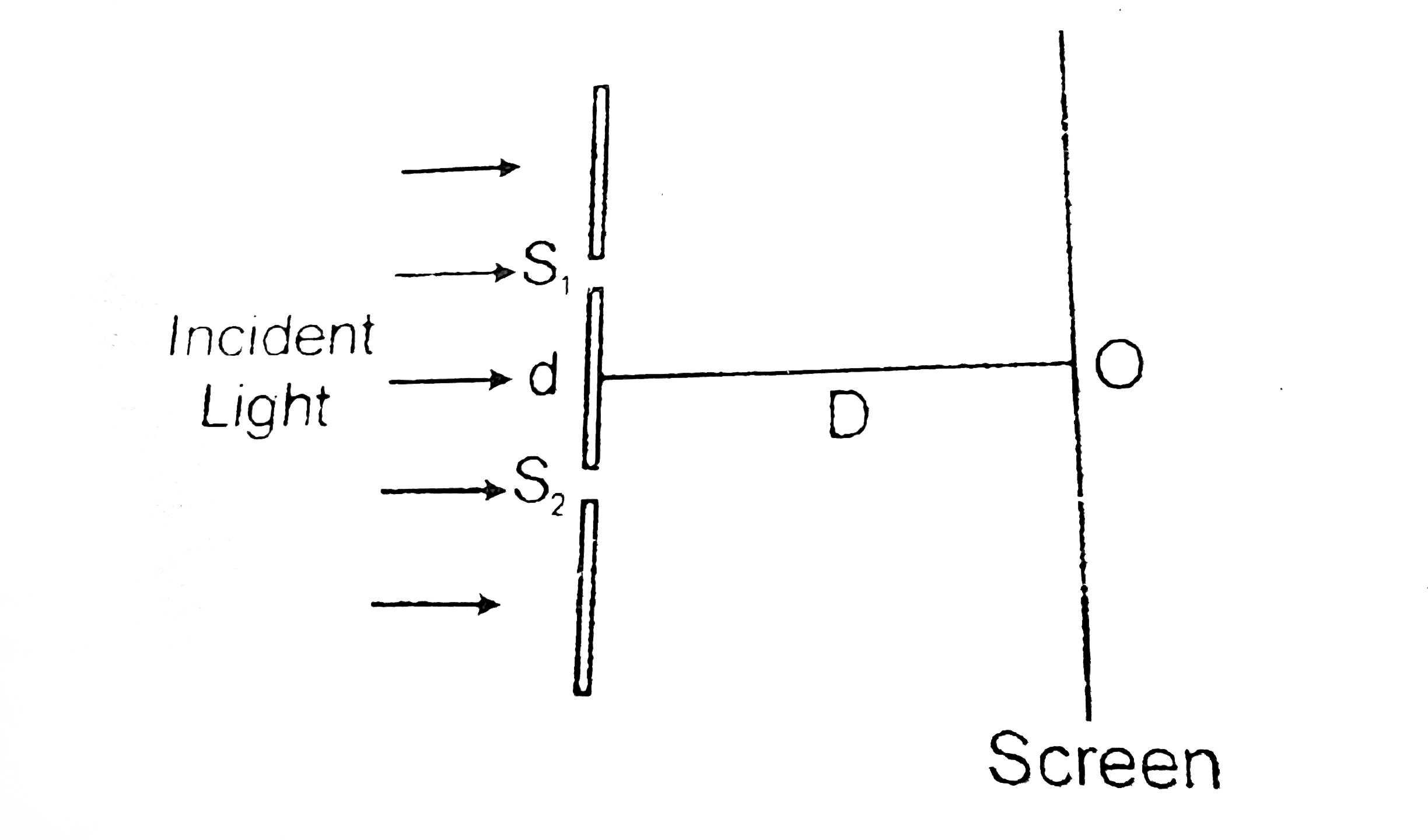
After placing the film of slit S1, the intensity at point O screen is :View SolutionFigure shows a YDSE setup having identical slits S1 and S2 with d =5 mm and D = 1 m. A monochromatic light of wavelength λ=6000Å is incident on the plane of slit due to which at screen centre O, an intensity I0 is produced with fringe pattern on both sides Now a thin transparent film of 11μm thickness and refractive index μ=2.1 is placed in front of slit S1 and now interference patten is observed again on screen.

After placing the film of slit S1, the intensity at point O screen is :View SolutionIn a modified YDSE, the region between the screen and slits is immersed in a liquid whose refractive index varies with time as μ1=(5/2)−(T/4) until it reaches s steady state value of 5/4. A glass plate of thickness 36 μm and refractive index 3/2 is introduced in front of one of the slits.
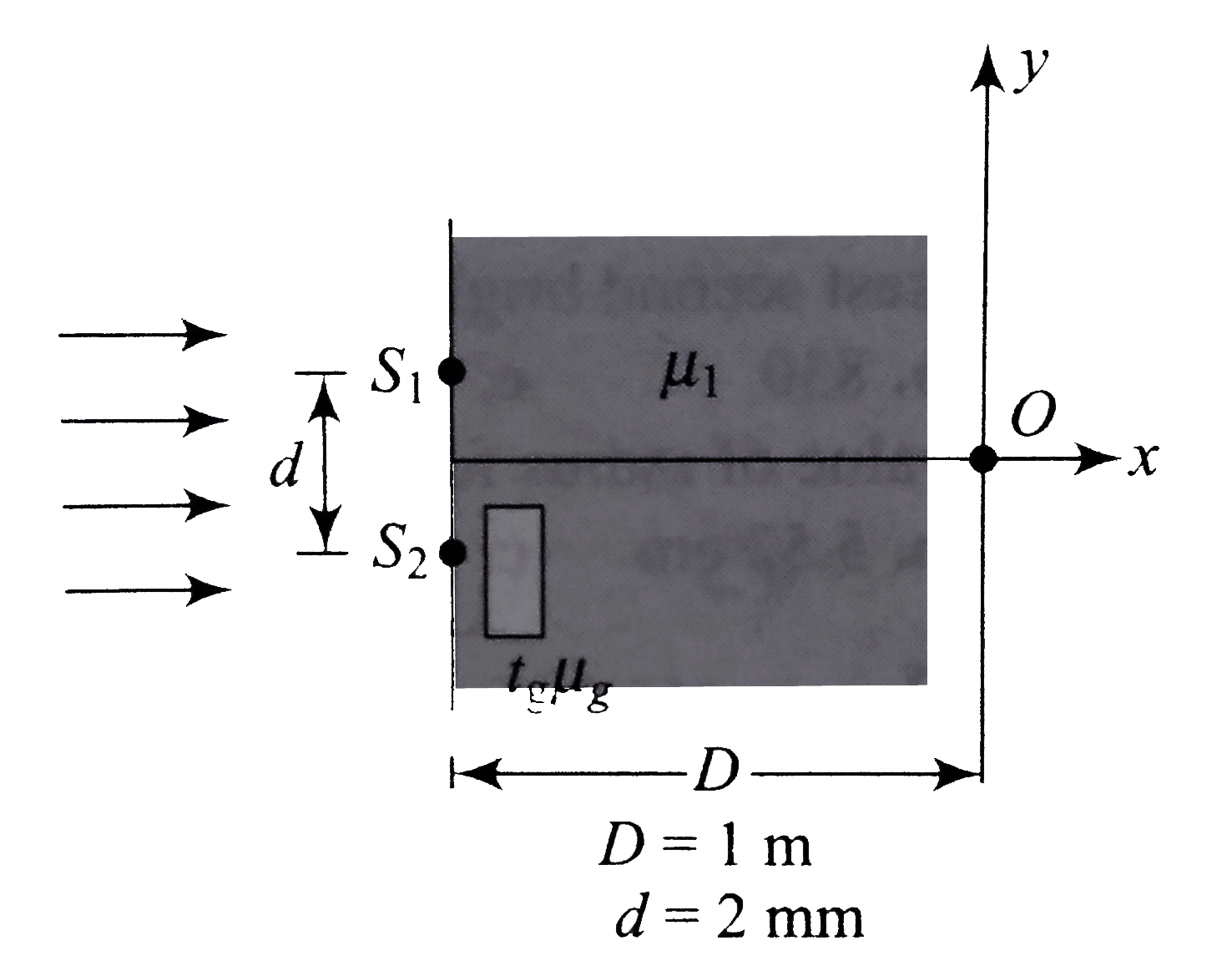
Find the time when central maxima is at point O. located symmetrically on the x-asix.View SolutionIn a doulble slit experiment when a thin film of thickness t having refractive index μ is introduced in from of one of the slits, the maximum at the centre of the fringe pattern shifts by one width. The value of t is (λ is the wavelength of the light used)
View SolutionIn Young's double slit experiment S1 and S2 are two slits. Films of thickness t1 and t2 and refractive indices μ1 and μ2 are placed in front of S1 and S2 respectively. If μ1t1=μ2t2, then the central maximum will :
 View Solution
View SolutionIn a modified YDSE, the region between the screen and slits is immersed in a liquid whose refractive index varies with time as μ1=(5/2)−(T/4) until it reaches s steady state value of 5/4. A glass plate of thickness 36 μm and refractive index 3/2 is introduced in front of one of the slits.

What is the speed of the central maxima when it is atView SolutionA glass slab (μ=1.5) of thickness 6 cm is placed over a paper. What is the shift in the letters?
View Solution

