In Young's experiment performed with light or wavelength 5500 Å what should be the separation between the two slits so that the highest order of maximum intensity in the interference pattern is 2 ? You may assume D>>d.
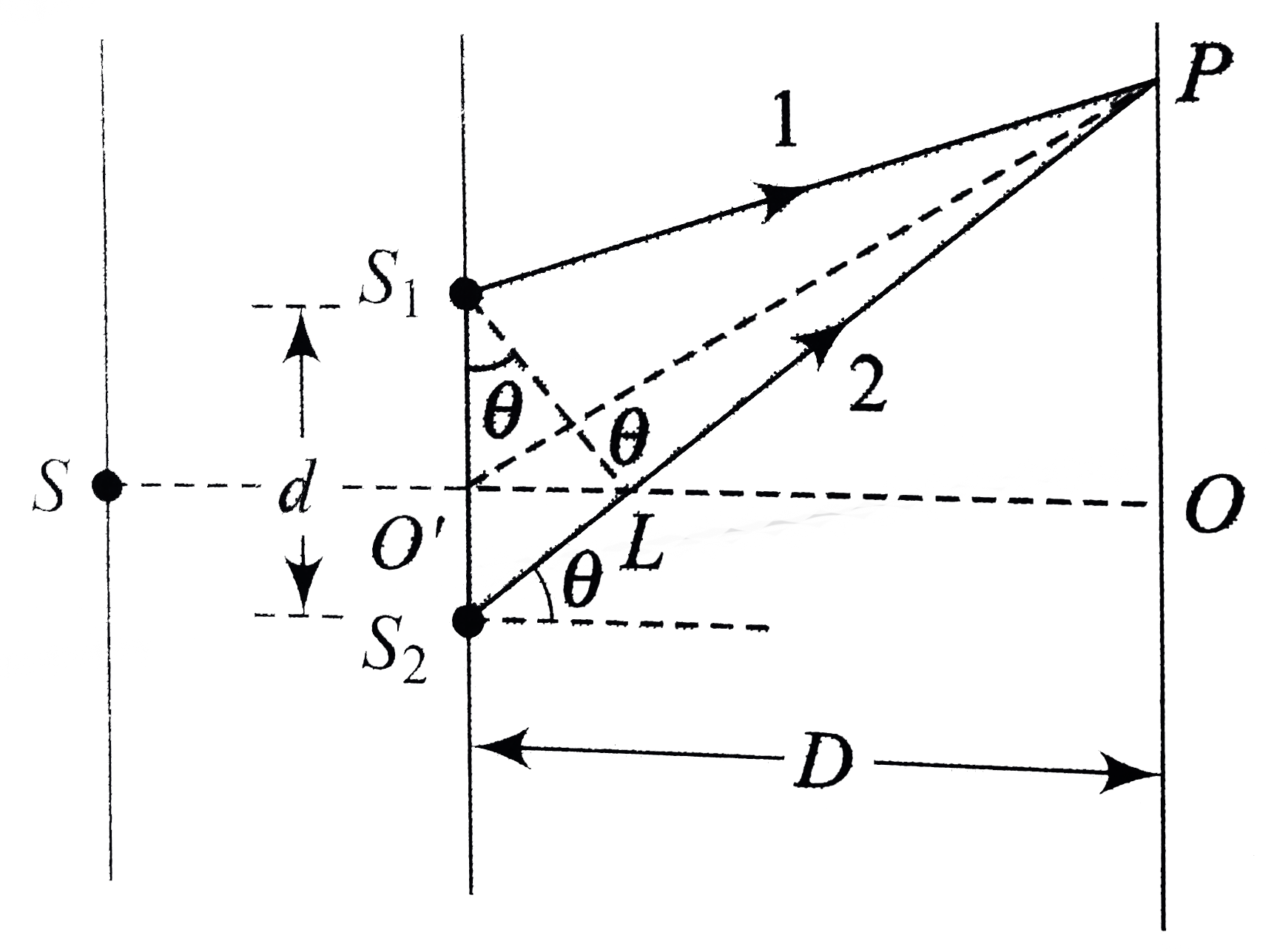
S2L=dsinθ or Δx=dsinθ
For intensity at P to be maximum,
Δxdsinθ=nλ
⇒sinθ=nλd
Now, sinθ≤1⇒nλd≤1 or n≤dλ⇒nmax=dλ
In this problem, nmax=2 and λ=5500Å=5500×10−10m.
∴d=nmaxλ=2×5500×10−16m
Topper's Solved these Questions
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Solved Examples
10 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Exercise 2.1
12 Videos
- THERMODYNAMICSBOOK - CENGAGE PHYSICSCHAPTER - THERMODYNAMICSEXERCISE - QUESTION BANK
34 Videos
- WAVESBOOK - CENGAGE PHYSICSCHAPTER - WAVESEXERCISE - QUESTION BANK
38 Videos
Similar Questions
In Young's experiment, light of wavelength 600 nm falls on the double slits separated by 0.1 mm. What is the highest order of maximum intensity in the interference pattern obtained on a screen kept 3 m from the slits? How does the highest order change if the distance of screen from the slits is changed?
View SolutionIn Young's double slit experiment, the widths of two slits are n the ratio 4:1. The ratio of maximum and minimum intensity in the interference pattern will be :
View Solution
Knowledge Check
Young’s double slit experiment is performed with light of wavelength 550 nm . The separation between the slits is 1.10mm and screen is placed at distance of 1 m . What is the distance between the consecutive bright or dark fringes
A1.5mmB1.0mmC0.5mmDNone of theseIf the width ratio of the two slits in Young's double slit experiment is 4:1, then the ratio of intensity at the maxima and minima in the interference patternn will be
A22121B(22121)2C441D121The intensity of the light coming from one of the slits in a Young's double slit experiment is double the intensity from the other slit. Find the ratio of the maximum intensity to the minimum intensity in the interference fringe pattern observed.
A34B40C25D38
Can we perform Young's doubel slilt experiment with shound waves? To get a resonable fringe pattern what should be the order of separation between the slits? How can the bright fringes an the dark fringes be detected in this case?
View SolutionIn Young's double-slit experiment using light of wavelength 5000Å, what phase difference corresponds to the 11th dark fringe from the center of the interference pattern?
View SolutionTwo slits 4.0×10−6 m apart are illuminated by light of wavelength 600nm . What is the highest order fringe in the interference pattern?
View SolutionYoung's interference experiment is performed with monochromatic light. The separation between the slits is 0.500 mm, and the interference pattern on a screen 3.30 m away shows the first side maximum 3.40 mm form the center of the pattern. What is the wavelength ?
View SolutionThe two slits in Young's experiment have widths in a rations 100:1 . Find the ratio of light intensity at the maxima and minima in the interference pattern .
View Solution
