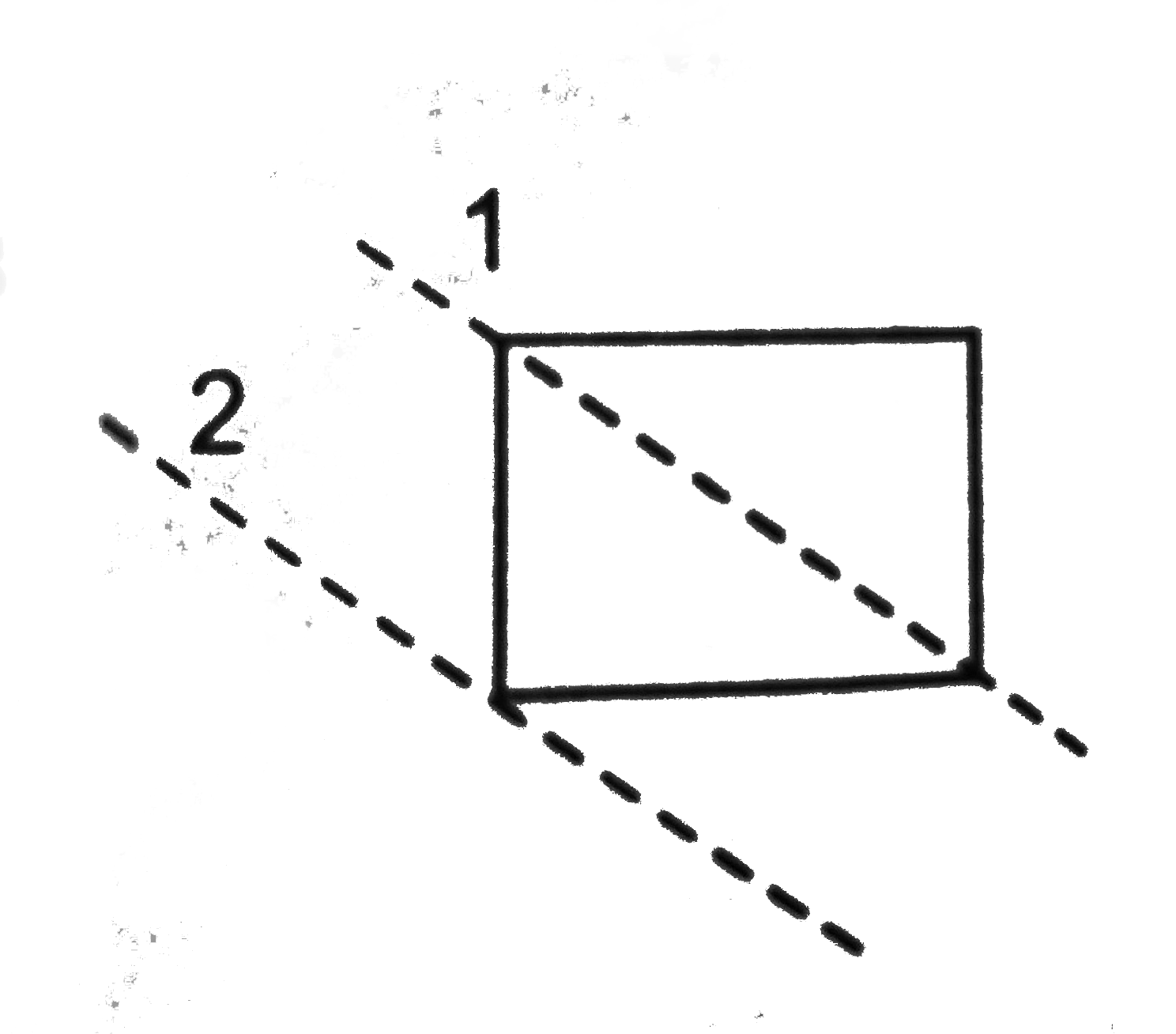
Let I1 and I2 be the moment of inertia of a uniform square plate about axes shown in the figure. Then the ratio I1:I2 is
1:17
1:127
1:712
1:7
The correct Answer is:D
I1=ma212
I2=I1+mr2
=ma212+ma22
=712ma2
∴I1:I2=1:7
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 1 Subjective
32 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 2 Single Correct
26 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Assertion And Reason
11 Videos
- ROTATIONBOOK - DC PANDEYCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEYCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
Similar Questions
A solid sphere of mass M and radius R is divided into two unequal parts. The first parts has a mass of 7M8 and is converted into a uniform disc of radius 2R. The second part is converted into a uniform solid sphere. Let I1 be the moment of inertia of the uniform disc about its axis and I2 be the moment of inertia of the sphere made from remaining part about its axis. The ratio I1/I2 is 140x. Find the value of x.
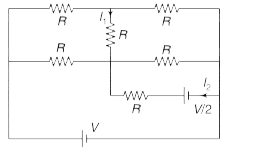
View SolutionIn the circuit shown in the figure. find I1andI2.
View Solution
Knowledge Check
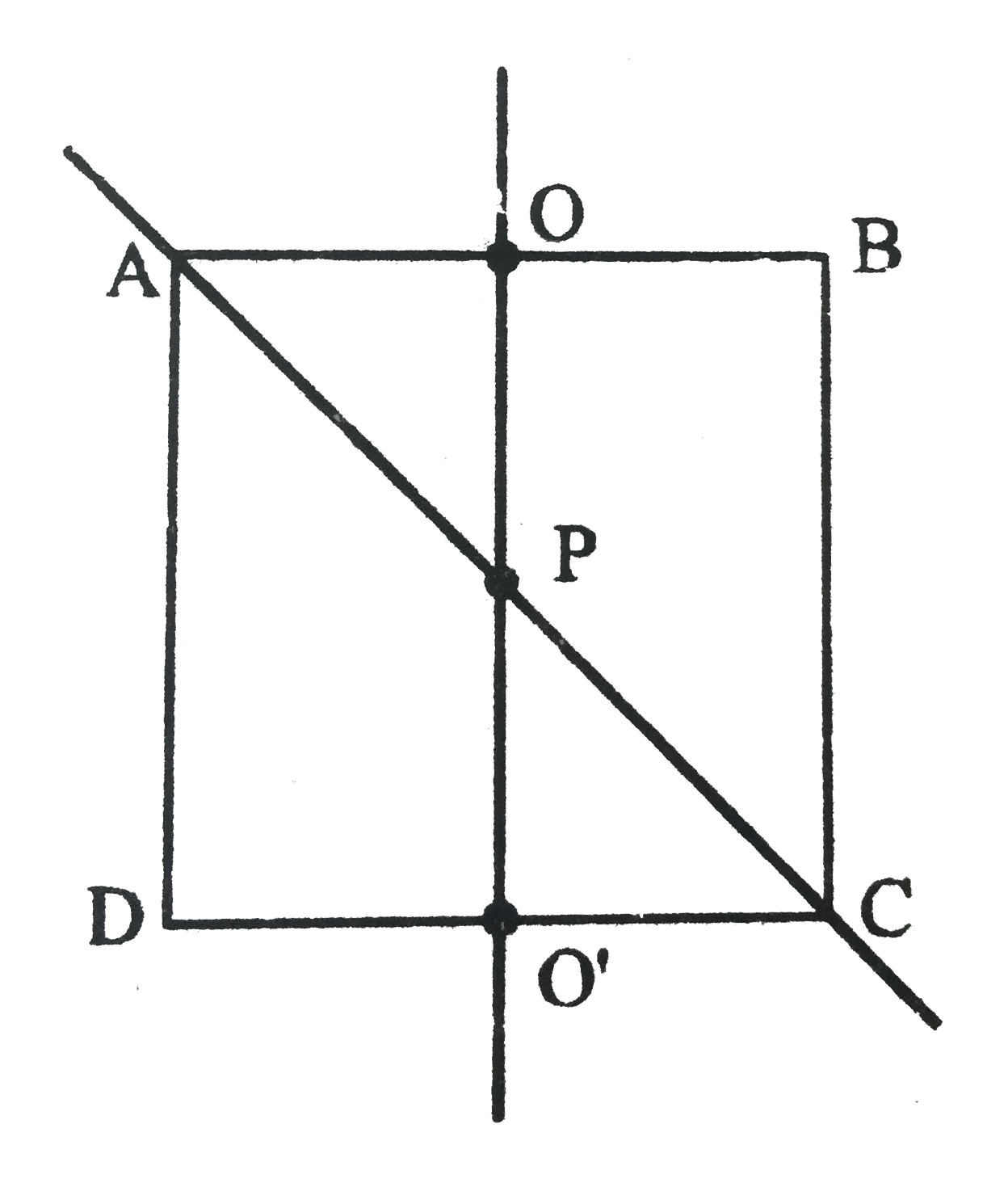
Let I1 and I2 be the moment of inertia of a uniform square plate about axes APC and OPO respectively as shown in the figure.P is centre of square. The ratio I1I2 moment of inertia is -
 .A12√2B2C12D1
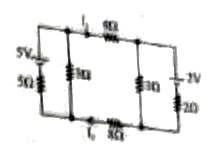
.A12√2B2C12D1In the circuit shown, the ratio I1I2 is equal to
 A4.0B3.0C2.5D1.0
A4.0B3.0C2.5D1.0In the circuit as shown in figure, the ratio of current i1/i2 is
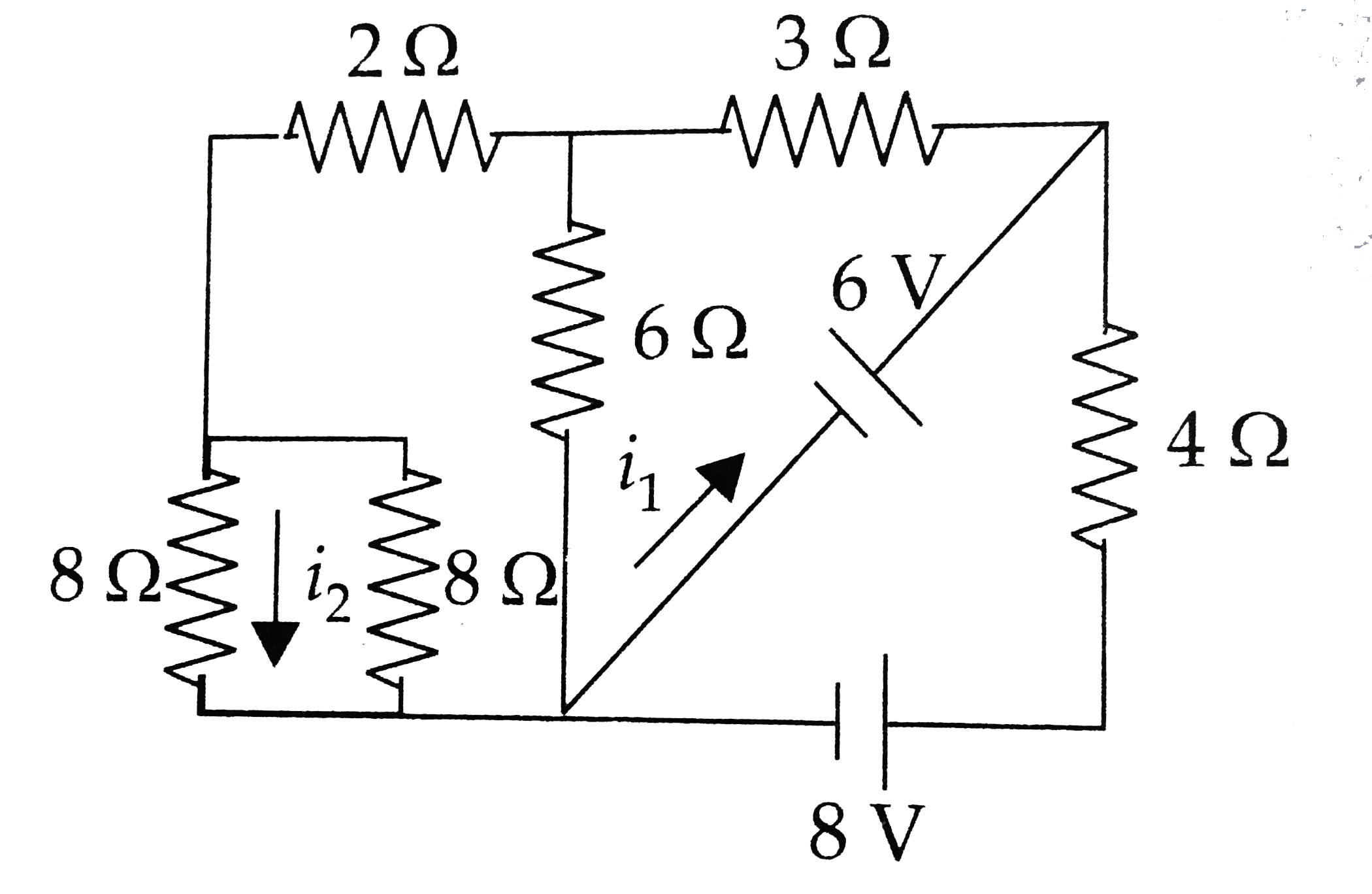 A4/3B1/2C2Dnone of these
A4/3B1/2C2Dnone of these
If I1 is the moment of inertia of a thin rod about an axis perpendicular to its length and passing through its centre of mass and I2 is the moment of inertia (about central axis) of the ring formed by bending the rod, then the ratio of I1 to I2 is
View SolutionA solid sphere of mass M and radius R is divided into two unequal parts. The first part has a mass of 7M8 and is converted into a uniform disc of radius 2R. The second part is converted into a uniform solid sphere. Let I1 be the moment of inertia of the disc about its axis and I2 be the moment of inertia of the new sphere about its axis. The ratio I1I2 is equal to __________ .
View SolutionIn the circuit shown in figure ,find the ratio of currents i1/i2.

View SolutionLet I be the moment of interia of a uniform square plate about an axis AB that passses through its centre and is parallel to two its sides. CD is a line in the plane of the plate that passes through the centre of the plate and makes an angle θ with AB. The moment of inertia of the plate about the axis CD is then equal to-
View SolutionIf I1 is the moment of inertia of a thin' rod about an axis perpendicular to its length and passing through the centre of mass and I2 is the moment of inertia of the ring formed by bending this rod in the form of a ring, then I1I2 is
View Solution
