Making use of the Maxwell distribution function, find <<1/v>>, the mean value of the reciprocal of the velocity of molecules in an ideal at a temperature T, it the mass of eacd molecule is equal to m. Compare the value obtained with the reciprocal of the mean velocity.
<<1v>>=∫∞0(m2πkT)3/2e−mv22kT4πv2dv1v
=(m2πkT)3/24π122kTm∫∞0e−xdx
=2(m2πkT)1/2=(2mπkT)1/2=(16π2mπ8kT)1/2=4π<v>.
Topper's Solved these Questions
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - The Second Law Of Thermodynamics - Entropy
47 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Liquids Capillary Effects
25 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - The First Law Of Thermodynamics - Heat Capacity
36 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
Similar Questions
From the Maxwell distribution function frind <<v2x>>, the mean value of the squared vx projection of the molecular velocity in a gas at a temperature T. The mass of each molecule is equal to m.
View SolutionUsing the Maxwell distribution function, calculate the mean velocity projection (vx) and the mean value of the modules of this projection <<|vx|>> if the mass of each molecule is equal to m and the gas temperature is T.
View Solution
Knowledge Check
The root mean square velocity of a gas molecule at any temperature T K of a gas molecule of molecular weight M is
A√8RT/MB√2RT/MC√3RT/MDzeroLet V denote the root mean square speed of the molecules in an ideal diatomic gas at absolute temperature T. The mass of a molecule is m. Neglecting vibrational energy terms, which is True?
Aa molecule can have a speed greater than √2vBv is proportional to √TCthe average rotational kinetic energy of a molecule is mv24Dthe average kinetic energy of a molecule is 5mv26The root mean square velocity of a gas molecule of mass m at a given temperature is proportional to
Am0BmCm1/2Dm−1/2
Making use of the Maxwell distribution function, calculate the number v of gas molecules reaching a unit area of a wall per unit time, if the concentration of molecules is equal to n, the temperature to T, and the mass of each molecule is m.
View SolutionThe root mean square velocity of a gas molecule of mass m at a given temperature is proportional to
View SolutionThe rms velocity of molecules of a gas at temperature T is Vrms. Then the root mean square of the component of velocity in any one particular direction will be –
View SolutionThe velocity distribution of molecules in a beam coming out of a hole in a vessel is described by the function F(v)=Av3e−mv2/2kT, where T is the temperature of the gas in the vessel. Find the most probable values of
(a) the velocity of the molecules in the beam , compare the result obtained with the most probable velocity of the molecules in the vessel ,
(b) the kinetic energy of the molecules in the beam.
View SolutionRead the following paragraph and answer the questions given below:
According to Maxwell distribution, the area under the curve is equal to the total number of molecules in collision. On increasing temperature, fraction of molecules having speed equal to ump (most probable speed) decreases. The speed distribution also depends on the mass of the molecules along with the temperature.
In general, the distribution depends upon the value of MT (where M is molar mass and T is temperature in kelvin).
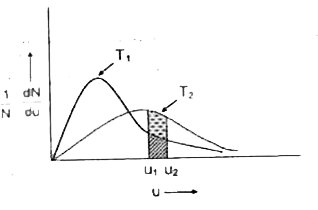
Choose the correct option:
Relationship between T1andT2 a is given as
View Solution
