Match the following: (P = momentum of particle, K = kinetic energy of particle)

Video Solution

Text Solution

Verified by Experts

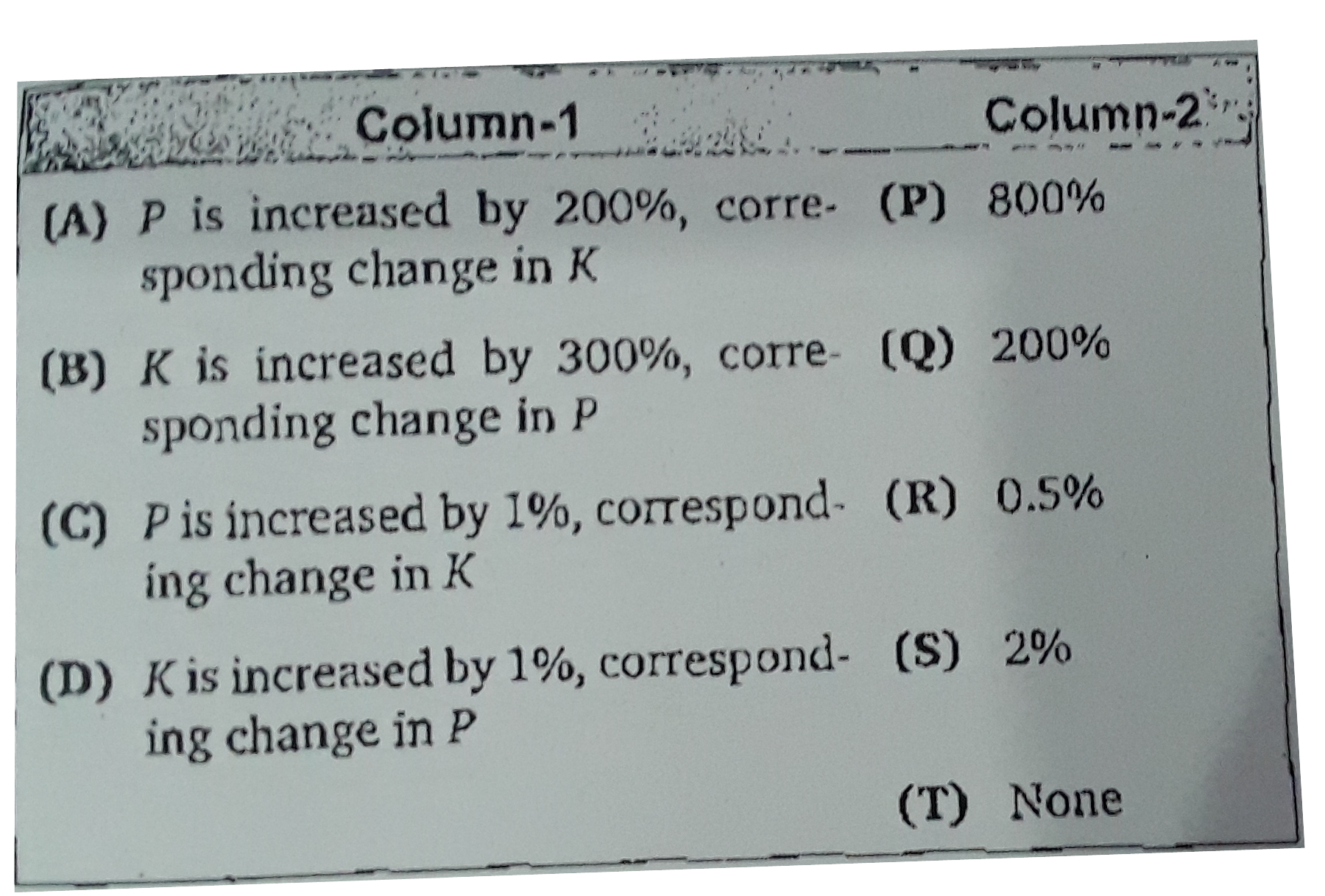
The correct Answer is:(a $\to$ p, B $\to$ q, C $\to$ s, D $\to$ r

(a) $K = \frac{p^{2}}{2 m}$
$\Rightarrow \frac{3 p^{2}}{2 m} = 9 (\frac{p^{2}}{2 m} = 9 K$
$∴$ % increase in K = 80% $< b r > (b)$ p=sqrt(2Km) $< b r >$ p^(') = sqrt(2(4K)m) = 2sqrt(2Km) = 2p
$∴ %$ increase in p = 100% $. < b r > (c)$ p = sqrt(2Km) = (2Km)^(1/2) $< b r >$ rArr K6(') = (101/100)^(2)p/2m = 2% $< b r > (d) % \in c r e a s e \in$ p =1/2 $(% I n c r e a s e \in K) = 0.5 %$

|

Class 11 PHYSICS CENTRE OF MASS

Topper's Solved these Questions

Similar Questions

Knowledge Check

For a moving particle (mass m, velocity v) having a momentum p, which one of the following correctly describes the kinetic energy of the particle?
A $\frac{p^{2}}{2 m}$
B $\frac{p}{2 m}$
C $\frac{v^{2}}{2 m}$
D $\frac{v}{2 m}$

DC PANDEY ENGLISH-CENTRE OF MASS-Match the coloumns

Home
Ask Doubt
Profile