Moment of inertia of a solid sphere about its diameter is I . If that sphere is recast into 8 identical small spheres, then moment of inertia of such small sphere about its diameter is
The correct Answer is:D
To solve the problem, we need to find the moment of inertia of each of the 8 identical small spheres that are formed from the original solid sphere.
1. Understand the Moment of Inertia of the Original Sphere:
The moment of inertia I of a solid sphere about its diameter is given by the formula:
I=25mR2
where m is the mass of the sphere and R is its radius.
2. Determine the Mass of Each Small Sphere:
When the original sphere is recast into 8 identical small spheres, the mass of each small sphere ms is:
ms=m8
3. Determine the Radius of Each Small Sphere:
The volume of the original sphere is:
V=43πR3
The volume of one small sphere is:
Vs=18V=18(43πR3)=16πR3
Let r be the radius of each small sphere. The volume of a small sphere can also be expressed as:
Vs=43πr3
Setting the two expressions for the volume equal gives:
43πr3=16πR3
Canceling π and multiplying through by 34:
r3=18R3
Taking the cube root:
r=R2
4. Calculate the Moment of Inertia of Each Small Sphere:
Using the formula for the moment of inertia for a solid sphere about its diameter, we can find the moment of inertia Is of each small sphere:
Is=25msr2
Substituting ms=m8 and r=R2:
Is=25(m8)(R2)2
Is=25(m8)(R24)
Is=25⋅mR232
Is=mR280
5. Relate Is to I:
Recall that I=25mR2. Therefore, we can express Is in terms of I:
Is=116I
Final Answer:
The moment of inertia of each small sphere about its diameter is:
Is=116I
The moment of inertia I of a solid sphere about its diameter is given by the formula:
I=25mR2
where m is the mass of the sphere and R is its radius.
When the original sphere is recast into 8 identical small spheres, the mass of each small sphere ms is:
ms=m8
The volume of the original sphere is:
V=43πR3
The volume of one small sphere is:
Vs=18V=18(43πR3)=16πR3
Let r be the radius of each small sphere. The volume of a small sphere can also be expressed as:
Vs=43πr3
Setting the two expressions for the volume equal gives:
43πr3=16πR3
Canceling π and multiplying through by 34:
r3=18R3
Taking the cube root:
r=R2
Using the formula for the moment of inertia for a solid sphere about its diameter, we can find the moment of inertia Is of each small sphere:
Is=25msr2
Substituting ms=m8 and r=R2:
Is=25(m8)(R2)2
Is=25(m8)(R24)
Is=25⋅mR232
Is=mR280
Recall that I=25mR2. Therefore, we can express Is in terms of I:
Is=116I
The moment of inertia of each small sphere about its diameter is:
Is=116I
Topper's Solved these Questions
Similar Questions
Knowledge Check
If temperature increases, the moment of inertia of a solid sphere about its diameter
AincreasesBdecreasesCremains unchangedDbecomes negativeThe M.1. of a solid sphere about its diameter is I. It is then casted into 8 small identical spheres. What is the M.I. of each small sphere about its diameter?
A18B124C116D132With the increase in temperate, moment of inertia of a solid sphere about the diameter.
AdecreasesBincreasesCdoes not changeDnone of these
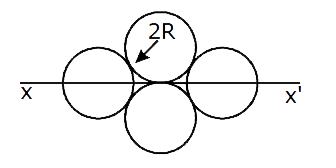
The moment of inertia of a sphere about its diameter is I. Four such spheres are arranged as shown in figure. Find the moment of inertia of the system about the axis XX'. (radius of each sphere is 2R).
View SolutionFind the moment of inertia of solid sphere of mass M about a diameter as shown in Fig.
View SolutionMoment of inertia of a solid sphere of radius R and density p about its diameter is
View SolutionA circular disc of radius R and thickness R/6 has moment of inertia I about an axis passing through its centre and perpendicular to its plane. It is melted and recast into a solid sphere. The M.I of the sphere about its diameter as axis of rotation is
View SolutionA disc of radius R and thickness has moment of inertia / about an axis passing through its centre and perpendicular to its plane. Disc is melted and recast into a solid sphere. The moment of inertia of a sphere about its diameter is
View Solution
