On earth, two parts of a space probe weigh 11 000 N and 3400 N. These parts are separated by a center-to-center distance of 12 m and may be treated as uniform spherical objects. Find the magnitude of the gravitational force that each part exerts on the other out in space, far from any other objects.
1.7×10−6 N
3.4×10−7N
1.8×10−7 N
3.6×10−5 N
The correct Answer is:C
To find the gravitational force that each part of the space probe exerts on the other, we can follow these steps:
Step 1: Calculate the masses of the two parts
The weight of an object (W) is related to its mass (m) by the equation:
W=m⋅g
where g is the acceleration due to gravity. On Earth, g≈9.8m/s2.
For the first part (weight = 11,000 N):
m1=W1g=11000N9.8m/s2≈1122.45kg
For the second part (weight = 3,400 N):
m2=W2g=3400N9.8m/s2≈346.94kg
Step 2: Use the gravitational force formula
The gravitational force (F) between two masses is given by Newton's law of universal gravitation:
F=Gm1⋅m2r2
where:
- G is the gravitational constant, G≈6.67×10−11N m2/kg2
- r is the distance between the centers of the two masses, which is given as 12 m.
Step 3: Substitute the values into the formula
Now, substituting the values we have:
F=6.67×10−11(1122.45kg)⋅(346.94kg)(12m)2
Step 4: Calculate the force
Calculating the product of the masses:
m1⋅m2=1122.45⋅346.94≈389,040.53kg2
Calculating r2:
r2=122=144m2
Now substituting these values back into the force equation:
F=6.67×10−11389040.53144
F≈6.67×10−11⋅2705.28≈1.80×10−7N
Final Answer
The magnitude of the gravitational force that each part exerts on the other is approximately:
F≈1.80×10−7N
---
The weight of an object (W) is related to its mass (m) by the equation:
W=m⋅g
where g is the acceleration due to gravity. On Earth, g≈9.8m/s2.
m1=W1g=11000N9.8m/s2≈1122.45kg
m2=W2g=3400N9.8m/s2≈346.94kg
The gravitational force (F) between two masses is given by Newton's law of universal gravitation:
F=Gm1⋅m2r2
where:
- G is the gravitational constant, G≈6.67×10−11N m2/kg2
- r is the distance between the centers of the two masses, which is given as 12 m.
Now, substituting the values we have:
F=6.67×10−11(1122.45kg)⋅(346.94kg)(12m)2
Calculating the product of the masses:
m1⋅m2=1122.45⋅346.94≈389,040.53kg2
r2=122=144m2
F=6.67×10−11389040.53144
F≈6.67×10−11⋅2705.28≈1.80×10−7N
The magnitude of the gravitational force that each part exerts on the other is approximately:
F≈1.80×10−7N
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION - I EXERCISE - PRACTICE QUESTIONS (More than One Correct Choice Type)5videos
FORCE AND MOTION - I
- BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION - I EXERCISE - PRACTICE QUESTIONS (Linked Comprehension)10videos
FORCE AND MOTION - I
- BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION - I EXERCISE - PROBLEMS52videos
FORCE AND MOTION - I
- BOOK - RESNICK AND HALLIDAYCHAPTER - FLUIDSEXERCISE - PRACTICE QUESTIONS (Integer Type )5videos
FLUIDS
- BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION-IIEXERCISE - PRACTICE QUESTIONS (Integer Type)1videos
FORCE AND MOTION-II
Similar Questions
Thee uniform spheres each having a mass M and raius a are kept in such a way that each touches the other two. Find the magnitude of the gravitational force on any of the spheres due to the other two.
View SolutionThree uniform spheres, each having a mass M=5kg and radius a=2.5m are kept in such a way that each touches the other two. Find the magnitude of the gravitational force in GN on any of the spheres due to the other two.
View Solution
Knowledge Check
- Question 1 - Select One
Two parallel wires of length 9m each are separated by a distance 0.15m .If they carry equal currents in the same direction and exerts a total force of 30×10−7N on each other, then the value of current must be
A2.5ampB3.5ampC1.5ampD0.5amp - Question 2 - Select One
Two charges exert a force of 10 N on each other when separated by a distance 0.2 m in air. When they are placed in another medium of dielectric constant K=4, and separated by distance R. they exert same force. The distance R equats to
A2mB1mC0.2mD0.1m - Question 3 - Select One
Statement I: In free space a uniform spherical planet of mass M has a smooth narrow tunnel along its diameter. This planet and another superdense small particle of mass M start approaching towards each other from rest under action of their gravitational forces. When the particle passes through the centre of the planet, sum of kinetic energies of both the bodies is maximum.
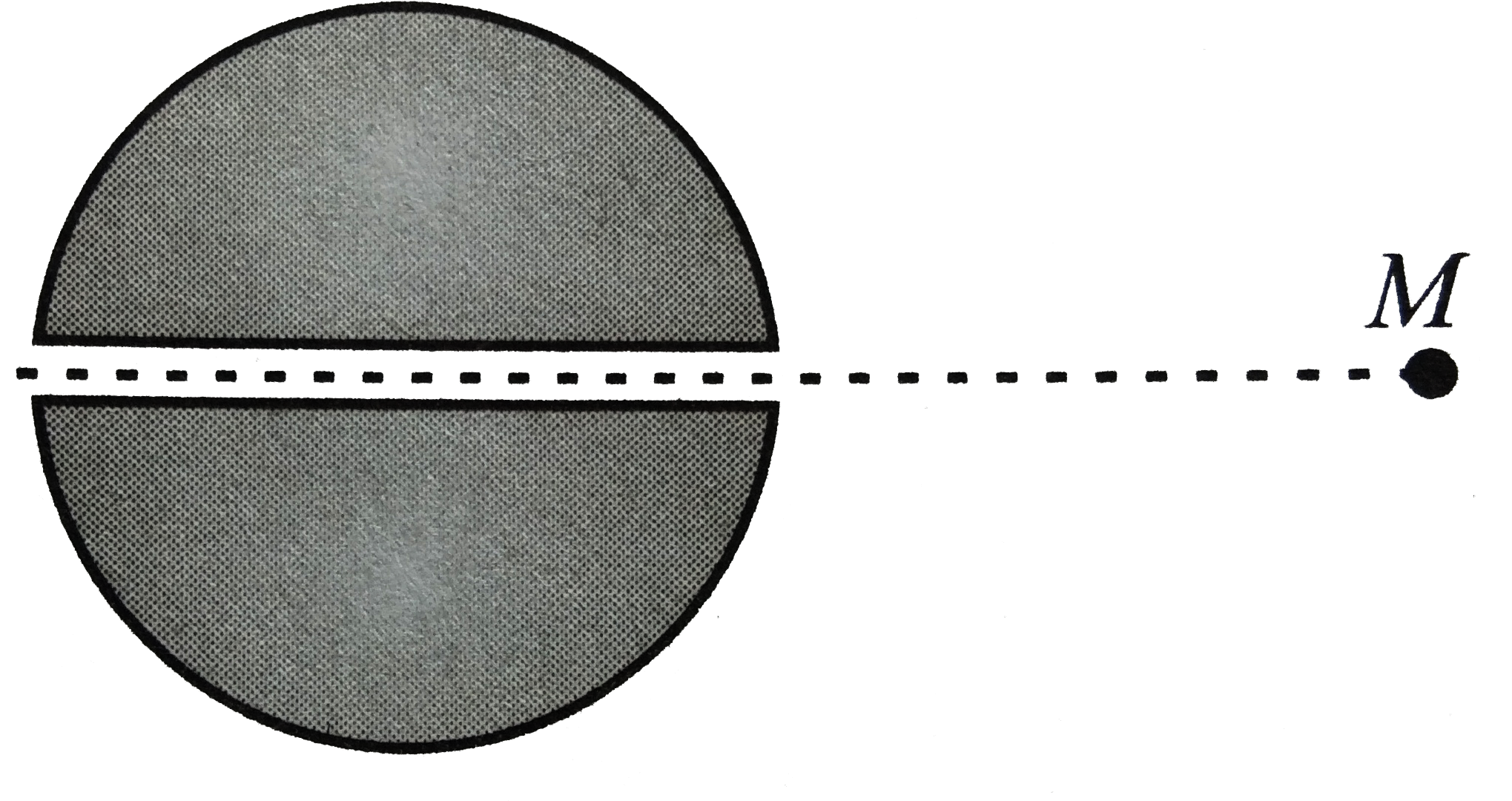
Statement II: When the resultant of all forces acting on a particle or a particle like object (initially at rest) is constant in direction, the kinetic energy of the particle keeps on increasing.AStatement I is True, Statement II is True: Statement II is a correct explanation for Statement I.BStatement I is True, Statement II is True: Statement II is Not a correct explanation for Statement I.CStatement I is True, Statement II is False.DStatement I is False, Statement II is True.
A particle is to be placed, in turn, outside four objects each of mass m:(1) a large uniform solid sphere, (2) a large uniform spherical shell,(3) a small uniform solid sphere, and (4) a small uniform shell. In each situation,the distance between the particle and the center of the object is d. Rank the objects according tothe magnitude of the gravitational force they exert on the particle, greatest first.
View SolutionA small object is suspended at rest from two strings as shown in fig. The magnitude of the force exerted by each string on the object is 10√2N. Find the magnitude of the mass of the object.
 View Solution
View SolutionConsider an object of mass M that is small enough to be treated as a particle but large enough that a section of mass m can be removed and positioned 3.0 mm from the remaining part. To maximize the magnitude of the gravitational force between the removed section and the remaining part, what should the ratio of m/M be?
View SolutionTwo equal charges repel each other with a force of 4×10−5N. when they are 0.03 m apart in air. Find the magnitude of the charges. What will be the force if the distance between them is doubled?
View SolutionTwo forces of magnitudes 3N and 4 N act together on an object making an angle 60∘ with each other. Find the resultant force acting on the object.
View Solution

