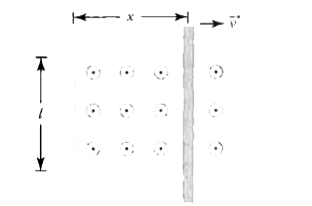
PQ is a conducting cross bar sliding on a rectangular closed bar STUR. There is uniform magnetic field in the area shown
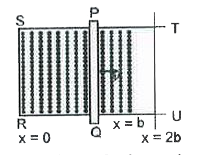
If R is the resistance of the rod PQ, assuming the resistance of the supporting bars are negligible. The force applied to pull out the rod at constant velocity up to x=b from x=0 is:
zero
BLVR
−Bl2vR
B2l2vR
Whe a rod with no constraints is moving with a constant velocity, no force is needed. However in this case, the current is in the downward direction. The lorentzian force acting on the current is llB. This is negative -llB. - TO cancel this force of friction, one has to apply an equal force llB in the positive direction. Force is
llB=BlvRlB=B2l2v2R
Topper's Solved these Questions
- E.M. INDUCTION AND A.C. CURRENTS BOOK - MODERN PUBLICATIONCHAPTER - E.M. INDUCTION AND A.C. CURRENTS EXERCISE - MULTIPLE CHOICE QUESTIONS LEVEL-II
51 Videos
- E.M. INDUCTION AND A.C. CURRENTS BOOK - MODERN PUBLICATIONCHAPTER - E.M. INDUCTION AND A.C. CURRENTS EXERCISE - MULTIPLE CHOICE QUESTIONS LEVEL -III (QUESTIONS FROM AIEEE/JEE EXAMINATION)
14 Videos
- DESCRIPTION OF MOTION IN TWO AND THREE DIMENSIONBOOK - MODERN PUBLICATIONCHAPTER - DESCRIPTION OF MOTION IN TWO AND THREE DIMENSIONEXERCISE - Revision TEST
36 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - RECENT COMPETITIVE QUESTIONS
4 Videos
Similar Questions
A metal rod of length l cuts across a uniform magnetic field B with a velocity v. If the resistance of the circuit of which the rod forms a part is r, then the force required to move the rod is
View SolutionA metal rod of length l cuts across a uniform magnetic field B with a velocity v. If the resistance of the circuit of which the rod forms a part is r, then the force required to move the rod is
View Solution
Knowledge Check
Two long parallel conducting rails are placed in a uniform magnetic field. On one side, the rails are connected with a resistance R. Two rods MNandM'N' each having resistance r are placed as showing in Fig. Now on the rods MNandM'N' forces are applied such that the rods move with constant velocity v.
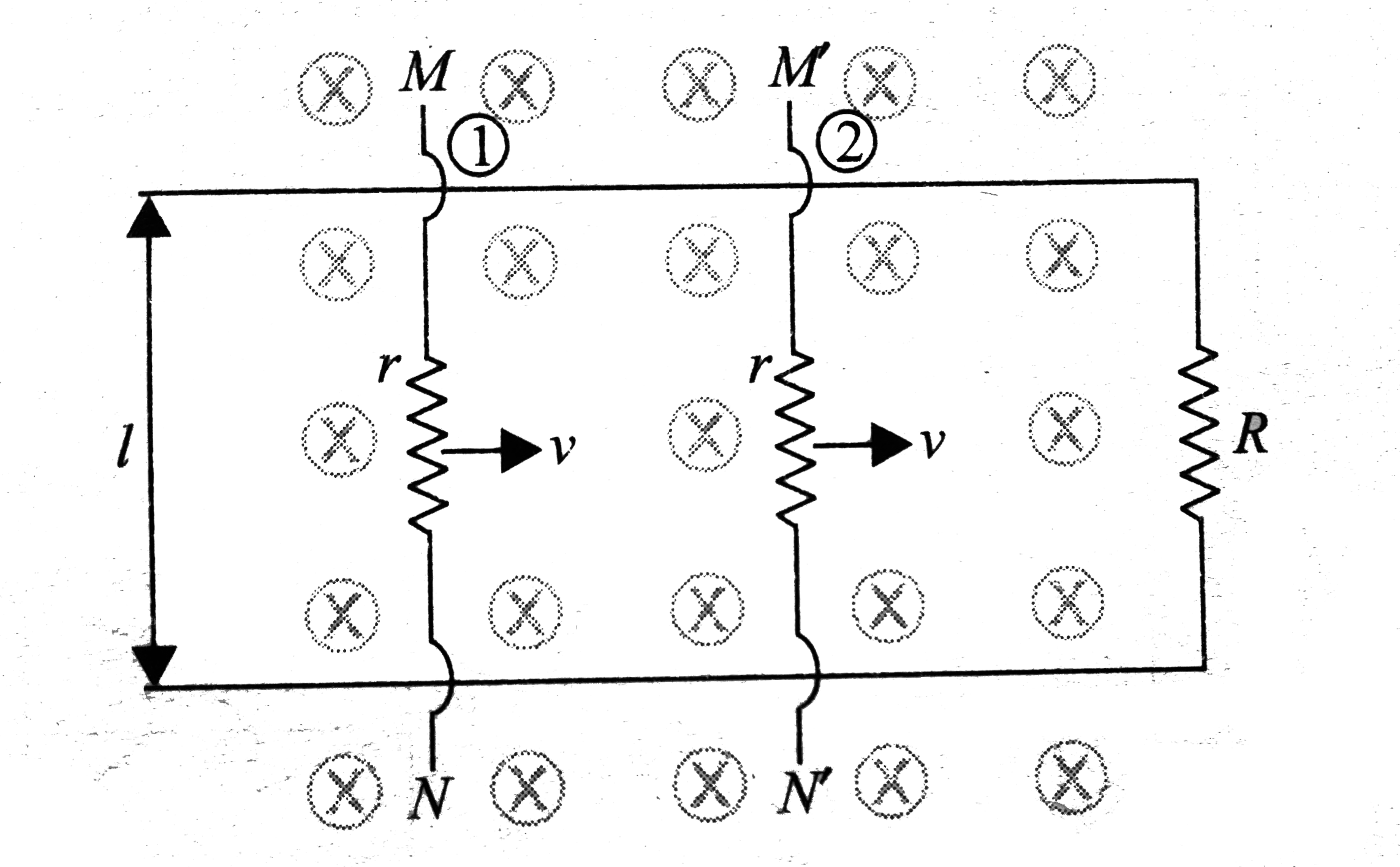
(ii). If in the previous problem resistance of values R1andR2 are connected on both ends as shown in Fig. current I, flowing through resistance R1 is given byA(a) BlR2(v1r2−v2r1)R1R2(r1+r2)+r2r1(R1+R2)B(b) BlR2(v1r2+v2r1)R1R2(r1+r2)+r2r1(R1+R2)C( c) BlR2(v1r2−v2r1)R1R2(r1−r2)+r2r1(R1−R2)D(d) BlR2(v1r2−v2r1)R1R2(r1+r2)−r2r1(R1+R2)A metallic rod completes its circuit as shown in the figure. The circuit is normal to a magnetic field of B=0.15 tesla. If the resistances of the rod is 3Ω the force required to move the rod with a constant velocity of 2m/sec is-
 A3.75×10−3NB3.75×10−2NC3.75×102ND3.75×10−4N
A3.75×10−3NB3.75×10−2NC3.75×102ND3.75×10−4NTwo long parallel conducting rails are placed in a uniform magnetic field. On one side, the rails are connected with a resistance R. Two rods MNandM'N' each having resistance r are placed as showing in Fig. Now on the rods MNandM'N' forces are applied such that the rods move with constant velocity v.
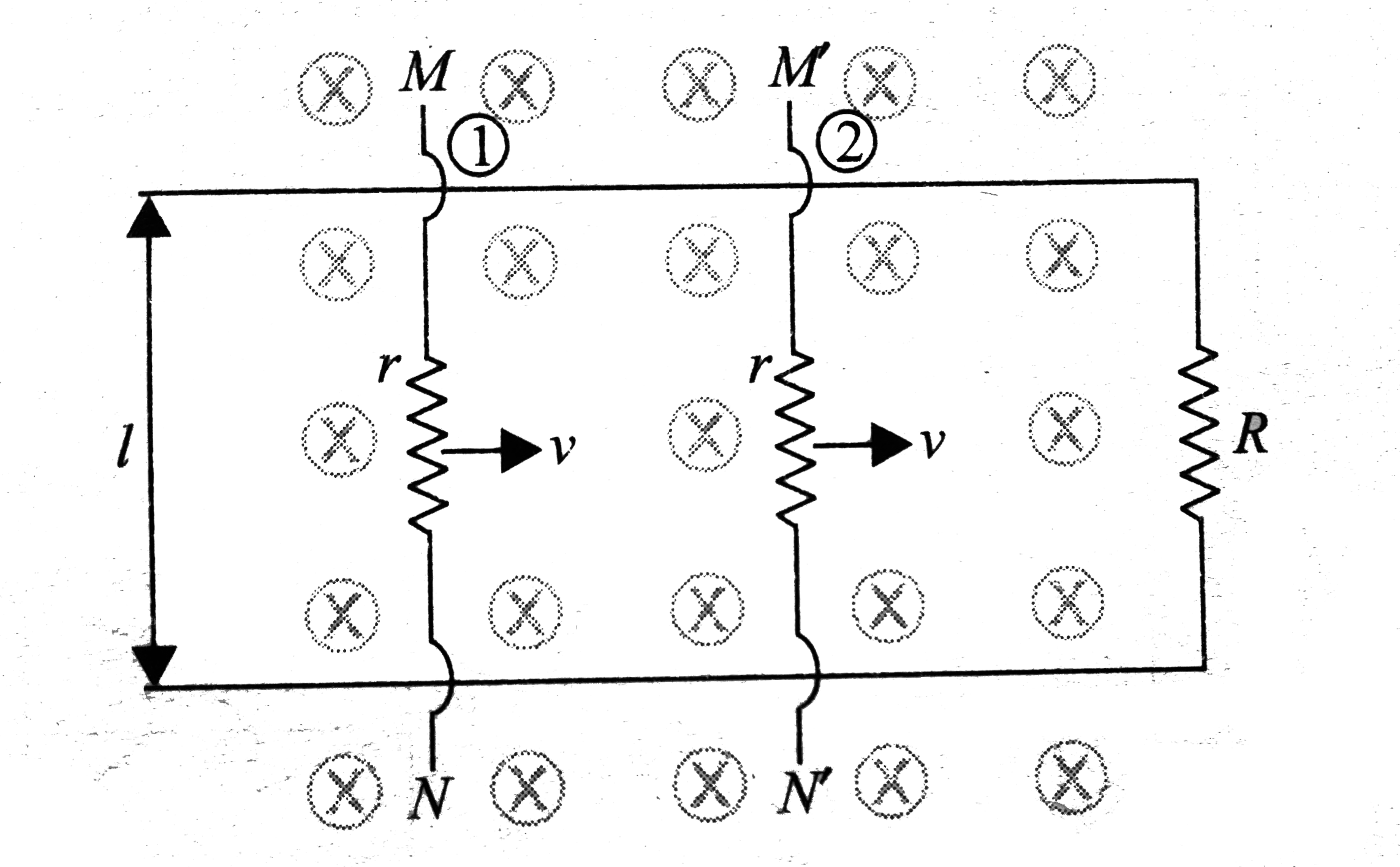
(i). The current flowing through resistance R if the rod Mn moves toward left and the rod M'N' moves toward the right isA(a) BlvR+(r/2)B(b) 2BlvR+rC( c) zeroD(d) 3Blv2(R+r)
Two long parallel conducting rails are placed in a uniform magnetic field. On one side, the rails are connected with a resistance R. Two rods MNandM'N' each having resistance r are placed as showing in Fig. Now on the rods MNandM'N' forces are applied such that the rods move with constant velocity v.

(i). The current flowing through resistance R if the rod Mn moves toward left and the rod M'N' moves toward the right is
View SolutionA conducting rod of resistance r moves uniformly with a constant speed v in a uniform magnetic field. If the rod keeps moving uniformly, then the amount of force required is
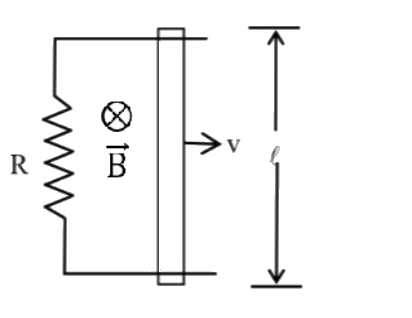
View SolutionA conducting rod of resistance r moves uniformly with a constant speed v in a uniform magnetic field. If the rod keeps moving uniformly, then the amount of force required is

View SolutionA metallic rod completes its circuit as shown in the figure. The circuit is normal to a magnetic field of B=0.15 tesla. If the resistances of the rod is 3Ω the force required to move the rod with a constant velocity of 2m/sec is-

View SolutionA rod with resistance R lies across frictionless conducting rails in a constant uniform magnetic field →B, as shown in the following figure. Assume the rails have negligible resistance. The magnitude of the force that must be applied by a person to pull the rod to the right at constant speed v is
View Solution
