Refraction of ray incident on a boundary of another plane
A ray given by −ˆi−2ˆj is incident on xz plane as shown in Fig. 34-4. Above the xz plane, refractive index is n1=2 and below the xz plane refractive index is n2=√5/2. Find a unit vector in the direction of the refracted ray.
(1) Note that although the incident ray is drawn in the first quadrant, its direction is in the third quadrant. This is because the direction of a vector is given by the direction in which its arrow points. To represent this vector in a vector form, we have to redraw it in such a way that its tail is at the origin. Note that refraction deviates a ray from its path, it does not changes the quadrant. For the complete direction of the refracted ray, we can use Snell.s law.
(2) First , we canvert the incident ray vector into a unit vector by dividing it by its magnitude. Then we can apply Snell.s law. As seen from Fig. 34-4, we can say that the tangential component of the incident ray is 1 sin theta because the magnitude of the incident ray vector has been made unity.
Similarly, the tangential component of the refracted ray is given by 1sinϕ. By Snell.s law,
n1sinθ=n2sinϕ
So, we can say that
n1× tangential component of incident ray =n2× tangential component of refracted ray.
Once we get the tangential component of the refracted ray, we get the normal component by using the fact that the refracted ray is also a unit vector. So , its magnitude is 1.
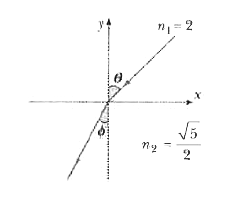
A ray travelling in x-y plane is incident on a boundary which separates the two media. The boundary of the media is x−z plane.
Calculation : Converting the incident ray vector to a unit vector :
ˆei=(−ˆi−2ˆj)√(−1)2+(−2)2=−ˆi√5−2√5ˆj.
From Fig. 34-4, it can be seen that the normal is in the y direction.
The component of this vector in the tangential direction (x direction in this case) is −ˆi/√5. So, by Snell.s law, we can say that
−ˆi√5×2=√52× tangential component of refracted ray.
Tangential component of refracted ray =−4i5
Let the normal component of the refracted ray be −cˆj.
(The refracted ray should also travel in the third quadrant). Threfore,
ˆer=−4ˆi5−cˆj
So,
1=√(−45)2+(−c)2
c=35.
Therefore,
ˆer=−45ˆi−35ˆj
Learn : In more involved problems , we can also involve the fact that incident ray, refracted ray, and normal all three lie in the same plane. Hence, their scalar triple product is zero. If one finds it encourging , then one must try this our : ˆe(ˆer׈n)=0
Topper's Solved these Questions
- GEOMETRICAL OPTICS : REFRACTIONBOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Check point
6 Videos
- GEOMETRICAL OPTICS : REFRACTIONBOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Problems
44 Videos
- GEOMETRICAL OPTICS : REFLECTION BOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFLECTION EXERCISE - PRACTICE QUESTIONS (Integer Type)
3 Videos
- GRAVITATIONBOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - PRACTICE QUESTIONS (INTEGER TYPE)
4 Videos
Similar Questions
Light is incident on a plane glass sheet at an angle of 70∘. Find the refractive index of glass sheet if the reflected and refracted rays are perpendicular to each other.
View SolutionThe x−y plane is the boundary between two transparent media. Medium-1 with z≤0 has a refractive index √2 and medium -2 with z≤0 has a refractive index √2. A ray of light in medium -1 given by vector A=√3ˆi−ˆk is incident on the plane of separation. The unit vector in the direction of the refracted ray in medium-2 is
View Solution
Knowledge Check
A ray of light is incident on the surface of plate of glass of refractive index 1.5 at the polarising angle. The angle of refraction of the ray will be
A33.7∘B23.7∘C43.7∘D53.7∘The x−y plane is the boundary between two transparent media. Medium-1 with z≤0 has a refractive index √2 and medium -2 with z≤0 has a refractive index √2. A ray of light in medium -1 given by vector A=√3ˆi−ˆk is incident on the plane of separation. The unit vector in the direction of the refracted ray in medium-2 is
A1√2(ˆk−ˆi)B1√2(ˆi+ˆj)C1√2(ˆi−ˆk)D1√2(ˆi+ˆk)For a given incident ray as shown in Fig., the condition of total internal reflection of ray will be satisfied if the refractive index of the block will be.
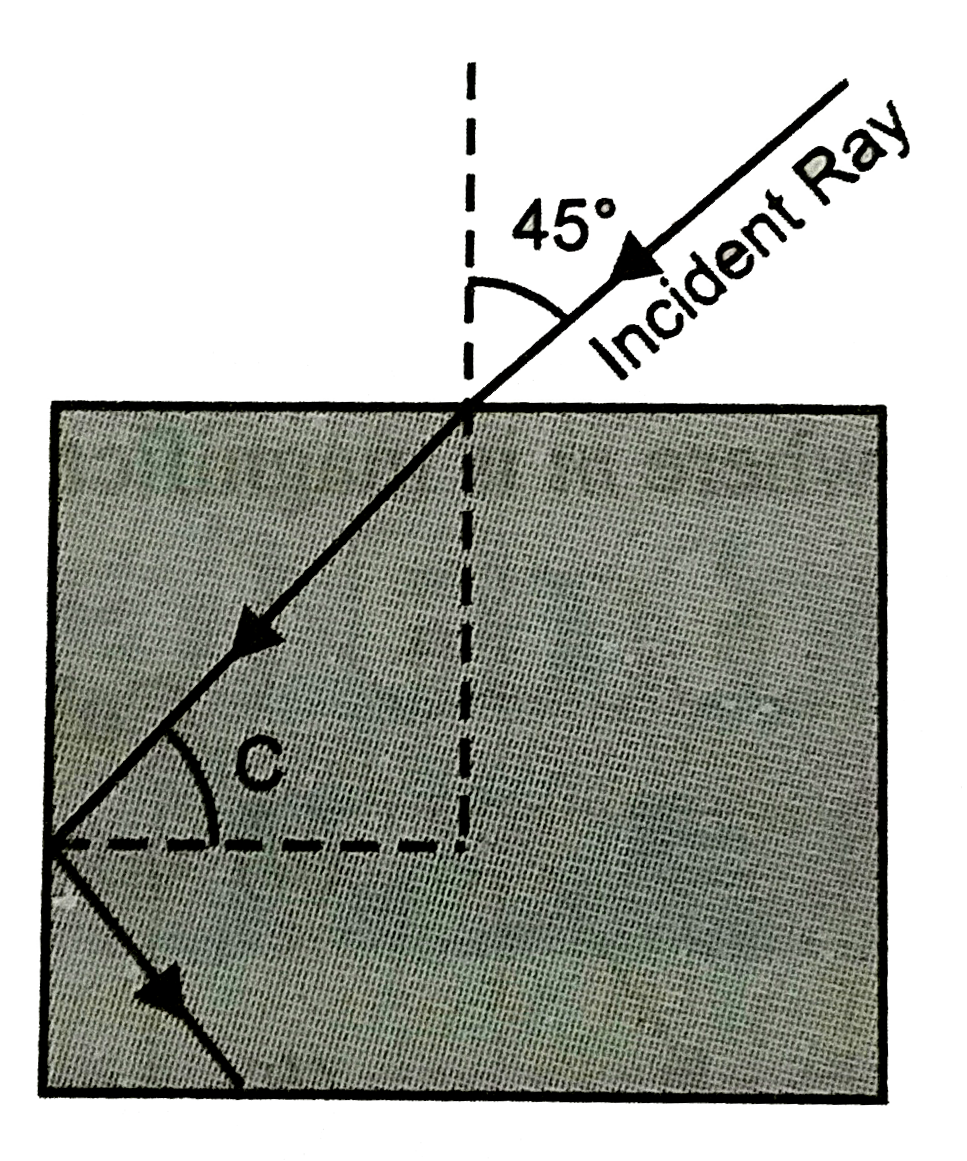 .A√3+12B√2+12C√32D√76
.A√3+12B√2+12C√32D√76
A ray of light travelling in air is incident on the plane surface of a transparent medium. The angle of incidence is 45∘ and the angle of refraction is 30∘ . Find the refractive index of the medium .
View SolutionFor a given incident ray as shown in Fig., the condition of total internal reflection of ray will be satisfied if the refractive index of the block will be.
 .
.
View SolutionA light ray incident along vector 2ˆi+4ˆj+√5ˆk strikes in the x−z from medium of refractive index √3 and enters into medium II of refractive index is μ2. The value of μ2 for which the value of angle of refraction becomes 90∘ is
View Solutionx-y plane separates two media, z≥0 contains a medium of refractive index 1 and z≤0 contains a medium of refractive index 2. A ray of light is incident from first medium along a vectorˆi+ˆj−ˆk. Find the unit vector along the refracted ray.
View SolutionA light ray incident along vector 2ˆi+4ˆj+√5ˆk strikes in the x−z from medium of refractive index √3 and enters into medium II of refractive index is μ2. The value of μ2 for which the value of angle of refraction becomes 90∘ is
View Solution
