Speed of sound in atmosphere at a height h0' is 1080kmhr–1. The variation of temperature and pressure of the atmosphere with height h from the surface is given by
T=T0−βh and P=P0(1−βhT0)MgRβ
Where T0= temperature at the surface of the earth =273K,
P0= atmospheric pressure at the surface of the earth,
β=0.006.∘C/m,M= molar mass of air ≅29gmol−1,g=9.8ms−2
R= gas constant =8.31Jmol−1K−1
consider air to be a mixture of diatomic hases and calculate the atmospheric temperature and pressure at height h0. Also find h0. Take (0.82)5.7=0.32.
The correct Answer is:T=224K,P=0.32P0,h0=8167m
Step by step video, text & image solution for Speed of sound in atmosphere at a height h_(0)' is 1080 km hr^( –1). The variation of temperature and pressure of the atmosphere with height h from the surface is given by T=T_(0)- beta h and P=P_(0) (1-(betah)/T_(0))^((Mg)/(R beta)) Where T_(0)= temperature at the surface of the earth =273 K, P_(0)= atmospheric pressure at the surface of the earth, beta=0.006 .^(@)C//m, M= molar mass of air cong 29 g mol^(-1), g=9.8 ms^(-2) R= gas constant =8.31 J mol^(-1) K^(-1) consider air to be a mixture of diatomic hases and calculate the atmospheric temperature and pressure at height h_(0). Also find h_(0). Take (0.82)^(5.7)=0.32. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A long taut string is plucked at its centre. The pulse travelling on it can be described as y(x,t)=e−(x+2t)2+e−(x−2t)2. Draw the shape of the string at time t=0, a short time after t=0 and a long time after t=0.
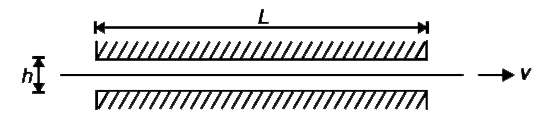
View SolutionDuring a painting process, a thin, flat tape of width b (dimension perpendicular to the plane of the figure) is pulled through a paint filled channel of length L. The density and viscosity of the paint liquid is ρ and η respectively. The tape is pulled at a constant speed v and width of the channel is h. Find the minimum force needed to pull the tape.
View Solution(i) The cause of increases in kinetic energy when a man starts running without his feet slipping on ground is asked to two students. Their answers are– Harshit: Cause of increase in kinetic energy is work done by friction force. Without friction the man cannot run. Akanksha: Cause of increase in kinetic energy is work done by internal (muscle) forces of the body. Who is right? (ii) An inextensible rope is hanging from a tree. A monkey, having mass m, climbs to a height h grabbing the rope tightly. The monkey starts from rest and ends up hanging motionlessly on the rope at height h. (a) How much work is done by gravity on the monkey? (b) How much work is done by the rope on the monkey? (c) Using work – energy theorem, explain the increase in mechanical energy of the monkey.
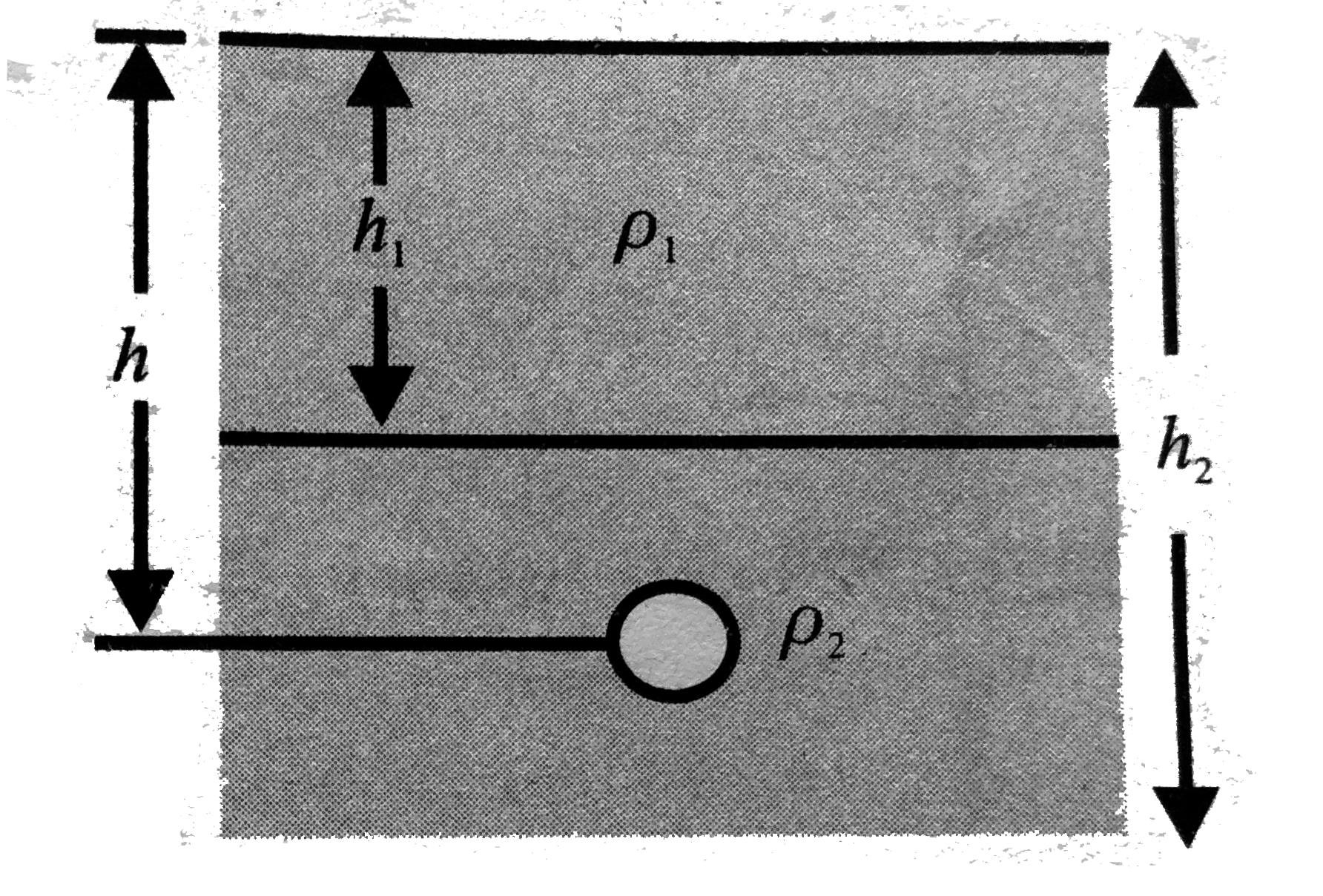
View SolutionCalculate the pressure inside a small air bubble of radus r situated at a depth h below the free surface of liquids of densities ρ1 and ρ2 and surface tennsions T1 and T2. The thickness of the first and second liquids are h1 and h2 respectively. Take atmosphere pressure =P0.
View SolutionThe air tight and smooth piston of a cylindrical vessel are connected with a string as shown. Initially pressure and temperature of the gas are P_(0) and T_(0) . The atmospheric pressure is also P_(0) . At a later time, tension in the string is (3)/(8) P_(0)A where A is cthe cross-sectional are of the cylinder. at this time, the temperature of the gas has become.
View SolutionAt the bottom of a lake where temperature is 7^(0)C the pressure is 2.8 atmosphere. An air bubble of radius 1 cm at the bottom rises to the surface. Where the temperature is 27^(0)C . Radius of air bubble at the surface is
View SolutionAssume that the temperature remains essentially constant in the upper parts of the atmosphere. The atmospheric pressure varies with height as. (the mean molecular weight of air is M, where P0= atmospheric pressure at ground reference)
View SolutionCloud formation condition Consider a simplified model of cloub formation. Hot air in contact with the earth's surface contains water vapor. This air rises convectively till the water vapor content reaches its situation pressure. When this happens, the water vpor starts condensing and droplets are formed. We shall estimate the estimate the height a which this happens.We assume that the atmosphere consists of the diatomic gases oxygen and nitrogen in the mass proportion 21:79 respectively. We further assume that the atmosphere is an ideal gas, g the acceleration due to gravity is constant and air processes are adiabatic. Under these assumptions one can show that the pressure is given by p = p_(0) ((T_(0)-T_(z))/(T_(0)))^(alpha) Here p_(0) and T_(0) is the pressure and temperature respectively at seal level (z = 0),T is the lapse rate (magnitude of the change in temperature T with height z abive the earth's surface, i.e. T gt 0) .
View SolutionAn adiabatic cylinder of cross section A is fitted with a mass less conducting piston of thickness d and thermal conductivity K. Initially, a monatomic gas at temperature T_(0) and pressure P_(0) occupies a volume V_(0) in the cylinder. The atmospheric pressure is P_(0) and the atmospheric temperature is T_(1) (gt T_(0)) . Find (a) the temperature of the gas as a function of time (b) the height raised by the piston as a function of time. Neglect friction and heat capacities of the container and the piston.
View SolutionA solid body A of mass m and specific heat capacity ‘s’ has temperature T1=400K. It is placed, at time t=0, in atmosphere having temperature T0=300K. It cools, following Newton’s law of cooling and its temperature was found to be T2=350Kat time t0. At time t0, the body A is connected to a large water bath maintained at atmospheric temperature T0, using a conducting rod of length L, cross section A and thermal conductivity k. The cross sectional area A of the connecting rod is small compared to the overall surface area of body A. Find the temperature of A at time t=2t0.
View SolutionIn troposhere, temperature varles linearly with elevation as T=T_(0)-ay, where T_(0) is the temperature at lthe earth's surface, then
View SolutionAn air bubble is formed at depth h below the surface of water. The pressure inside the bubble is- ( P_(0)= atmospheric pressure, r = radius of bubble)
View SolutionPressure at the Earth's surface is pa=105Pa and the density of air at Earth's surface is ρ0=1.4kg/m3. At height h from the surface of Earth the density of air is reduced to ρ02 the value of h is (Assume that the temperature is constant through out the earth's atmosphere and let In (2)=0.7)
View SolutionThe temperature of a room of volume Vrise from T1 to T2. How much will the mass of the air in the room changes if the atmospheric pressure is p0. The molecular weight of the air is M.
View SolutionConsidering the pressure 'P' to be proportional to the density of air 'd', the pressure at height 'h' if the pressure on surface of earth is 'P0' and density 'd0', under isothermal conditions
View Solution
