STATEMENT-1: The stream of water flowing at high speed from a garden hose pipe tends to spread like a fountain when held vertically up, but tends to narrow down when held vertically down.
STATEMENT-2: In any steady flow of an incompressible fluid, the volume flow rate of the fluid remains constant.
If both assertion and reason are true and the reason is correct explanation of the assertion.
If both assertion and reason are true but reason is not the correct explanation of assertion.
If assertion is true, but the reason is false.
If assertion is false, but the reason is true.
The correct Answer is:D
To analyze the statements provided in the question, we will break down the reasoning step by step.
Step 1: Understanding Statement 1
Statement 1 describes the behavior of water flowing from a garden hosepipe when held in two different orientations: vertically up and vertically down.
- When held vertically up: The stream of water spreads out like a fountain. This occurs because the water is moving against gravity, and as it exits the hosepipe, the kinetic energy of the water is converted into potential energy, causing the water to spread out.
- When held vertically down: The stream of water narrows down. In this case, the water is moving with gravity, and the acceleration due to gravity increases the speed of the water as it exits the hosepipe.
Step 2: Understanding Statement 2
Statement 2 states that in any steady flow of an incompressible fluid, the volume flow rate remains constant.
- The volume flow rate (Q) is defined as the product of the cross-sectional area (A) of the flow and the velocity (V) of the fluid:
Q=A×V
- For an incompressible fluid, the volume flow rate must remain constant throughout the flow. This means that if the area decreases, the velocity must increase to keep the product A×V constant.
Step 3: Applying the Continuity Equation
When the hosepipe is held down:
- The cross-sectional area (A) of the stream decreases as the water flows downward.
- According to the continuity equation, if the area decreases, the velocity (V) of the water must increase to maintain a constant flow rate.
Step 4: Conclusion
Based on the analysis:
- Statement 1 is true because it accurately describes the behavior of the water stream in both orientations.
- Statement 2 is also true as it correctly explains the principle of conservation of mass for incompressible fluids.
Furthermore, Statement 2 serves as the correct explanation for Statement 1, as it provides the underlying principle (continuity equation) that explains why the stream behaves differently when oriented in different directions.
Final Answer
Both statements are true, and Statement 2 is the correct explanation of Statement 1. Thus, the correct answer is option 1.
---
Statement 1 describes the behavior of water flowing from a garden hosepipe when held in two different orientations: vertically up and vertically down.
Statement 2 states that in any steady flow of an incompressible fluid, the volume flow rate remains constant.
Q=A×V
- For an incompressible fluid, the volume flow rate must remain constant throughout the flow. This means that if the area decreases, the velocity must increase to keep the product A×V constant.
When the hosepipe is held down:
- According to the continuity equation, if the area decreases, the velocity (V) of the water must increase to maintain a constant flow rate.
Based on the analysis:
- Statement 2 is also true as it correctly explains the principle of conservation of mass for incompressible fluids.
Both statements are true, and Statement 2 is the correct explanation of Statement 1. Thus, the correct answer is option 1.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - MCQ_TYPE
3 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - LC_TYPE
9 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - SCQ_TYPE
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
Statement-1: The stream of water emerging from a wate tap "necks down" as it falls.
Statement-2: The volume flow rate at different levels is same.
View SolutionStatement 1 : The velocity increases, when water flowing in broader pipe enter a narrow pipe.
Statement 2 : According to equation of continuity, product of area and velocity is constant.
View SolutionFigure shows water in a container having 2.0−mm thick walls made of a material of thermal conductivity 0.50Wm−1C−1 . The container is kept in a melting-ice bath at 0∘C . The total surface area in contact with water is0.05m2 . A wheel is clamped inside the water and is coupled to a block of mass M as shown in the figure. As the goes down, the wheel rotates. It is found that after some time a steady state is reached in which the block goes down with a constant speed of 10cms−1 and the temperature of the water remains constant at 1.0∘C .Find the mass M of the block. Assume that the heat flow out of the water only through the walls in contact. Take g=10ms−2 .

View SolutionAn uniform disc is rotating at a constant speed in a vertical plane about a fixed horizontal axis passing through the centre of the disc. A piece of the disc from its rim detaches itself from the disc at the instant when it is at horizontal level with the centre of the disc and moving upwards, then about the fixed axis.
STATEMENT-1 : Angular speed of the disc about the aixs of rotation will increase.
and
STATEMENT-2 : Moment of inertia of the disc is decreased about the axis of rotation.
View SolutionThe human ciculatory system can be thought of as a closed system of interconnecting pipes through which fluid is continuously circulated by two pumps the two pumps the right and left verticles of the heart, work as simple two-stroke force pumps. The muscles of the heart regulate the force by contracting and relaxing. the contraction (systole) lasts about 0.2s and a complete systole/diastole (contraction/relaxation) cycle lasts about 0.8s. For flood pressures and speeds in the normal range. the volume flow rate of blood through a blood vessel is directly proportional tot he pressure difference over a length of the vessel and to the fourth power of the radius of the vessel. The total mechanical energy per unit volume of blood just as it leaves the heart is E/V=ρgh+P+ρv2
Q. Why is diastolic blood pressure muchh lower than systolic pressure?
A. because the heart exerts more force on the blood during diastole
B. Because the heart exerts no force on the blood during diastole
C. Because the radii of the blood vessels increase during diastole while the force exerted by the heart on the blood remains the same.
D. Because the radii of the blood vessels increase during diastole while the force exerted by the heart on the blood remains the same.
View SolutionStatement-1 : When an orifice is made in the middle of the wall of a vessel, the range of the liquid coming out of the orifice is equal to the height of the liquid.
Statement-2 : Liquid is flowing through two identical pipes A and B. Volume of liquid flowing per second through A and B are v0and2v0 respectively. Flow in A is turbulent and steady in B.
Statement-3 : Rate of flow of a viscous liquid through a pipe is directly proportional to he fourth power of the radius of pipe.
View SolutionWhen an object moves through a fluid, as when a ball falls through air or a glass sphere falls through water te fluid exerts a viscous foce F on the object this force tends to slow the object for a small sphere of radius r moving is given by stoke's law, Fw=6πηrv. in this formula η in the coefficient of viscosity of the fluid which is the proportionality constant that determines how much tangential force is required to move a fluid layer at a constant speed v, when the layer has an area A and is located a perpendicular distance z from and immobile surface. the magnitude of the force is given by F=ηAv/z. For a viscous fluid to move from location 2 to location 1 along 2 must exceed that at location 1, poiseuilles's law given the volumes flow rate Q that results from such a pressure difference P2−P1. The flow rate of expressed by the formula Q=πR4(P2−P1)8ηL poiseuille's law remains valid as long as the fluid flow is laminar. For a sfficiently high speed however the flow becomes turbulent flow is laminar as long as the reynolds number is less than approximately 2000. This number is given by the formula Re=2¯vρRη In which ¯v is the average speed ρ is the density η is the coefficient of viscosity of the fluid and R is the radius of the pipe. Take the density of water to be ρ=1000kg/m3
Q. Calculate the highest average speed that blood (ρ≈1000kg/m3) could have and still remain in laminar flow when it flows through the arorta (R=8×10−3m) Take the coeffiicient of viscosity of blood to be 4×10−3Pa−s
View Solution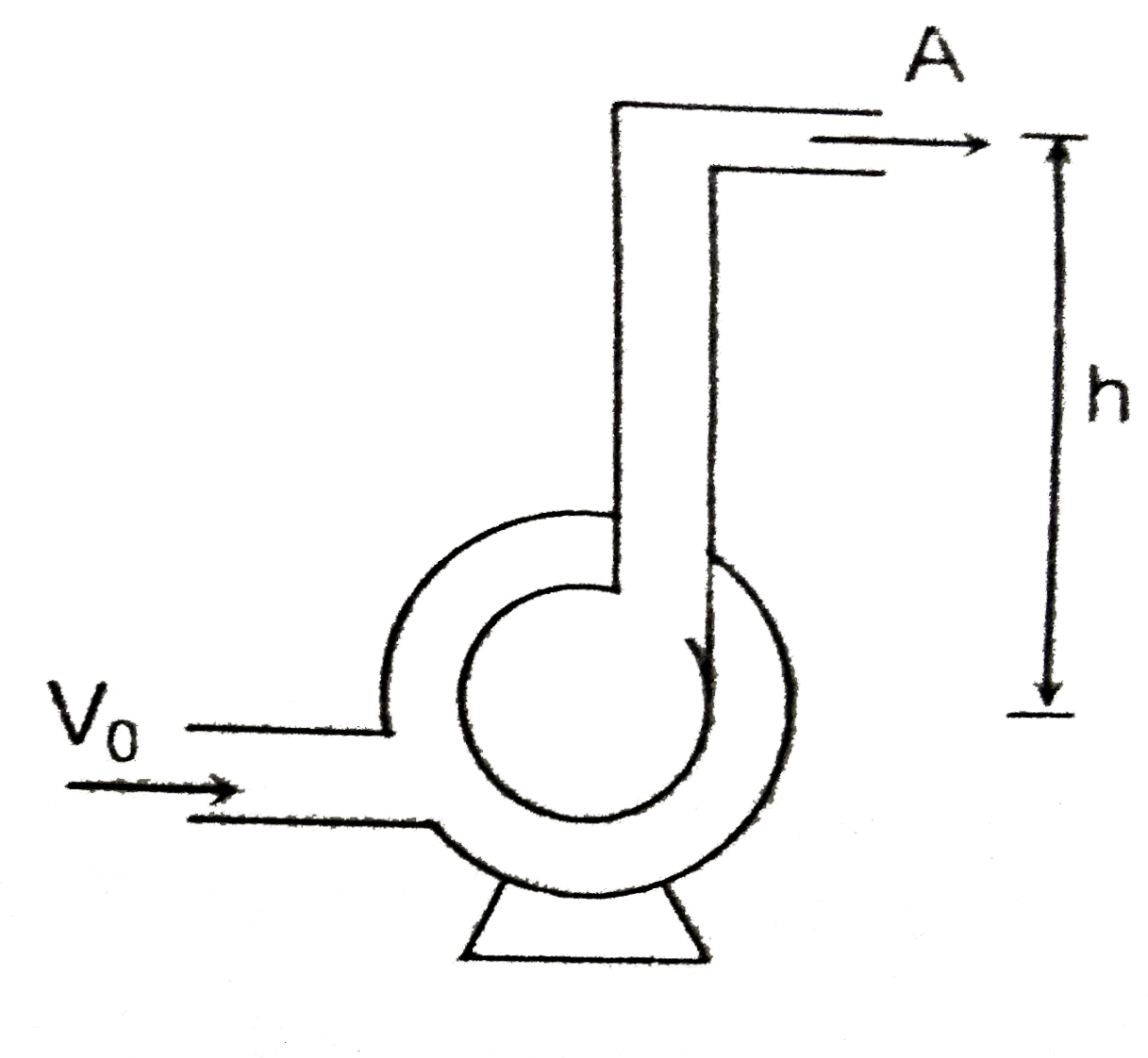
In a certain pump whose rated power is P. ideal fluid of denstiy ρ enters with speed V0 which lifts water upto height h through a pipe of uniform cross section area without discontinuity. Volumetric flow rate of the liquid is Q. Speed of fluid when it comes out of the pipe at point A is (Assume that pump works at 100 % efficiency and flow is streamline)
View SolutionWhen an object moves through a fluid, as when a ball falls through air or a glass sphere falls through water te fluid exerts a viscous foce F on the object this force tends to slow the object for a small sphere of radius r moving is given by stoke's law, Fw=6πηrv. in this formula η in the coefficient of viscosity of the fluid which is the proportionality constant that determines how much tangential force is required to move a fluid layer at a constant speed v, when the layer has an area A and is located a perpendicular distance z from and immobile surface. the magnitude of the force is given by F=ηAv/z. For a viscous fluid to move from location 2 to location 1 along 2 must exceed that at location 1, poiseuilles's law given the volumes flow rate Q that results from such a pressure difference P2−P1. The flow rate of expressed by the formula Q=πR4(P2−P1)8ηL poiseuille's law remains valid as long as the fluid flow is laminar. For a sfficiently high speed however the flow becomes turbulent flow is laminar as long as the reynolds number is less than approximately 2000. This number is given by the formula Re=2¯vρRη In which ¯v is the average speed ρ is the density η is the coefficient of viscosity of the fluid and R is the radius of the pipe. Take the density of water to be ρ=1000kg/m3
Q. Blood vessel is 0.10 m in length and has a radius of 1.5×10−3m blood flows at rate of 10−7m3/s through this vessel. The pressure difference that must be maintained in this flow between the two ends of the vessel is 20 Pa what is the viscosity sufficient of blood?
View SolutionWhen an object moves through a fluid, as when a ball falls through air or a glass sphere falls through water te fluid exerts a viscous foce F on the object this force tends to slow the object for a small sphere of radius r moving is given by stoke's law, Fw=6πηrv. in this formula η in the coefficient of viscosity of the fluid which is the proportionality constant that determines how much tangential force is required to move a fluid layer at a constant speed v, when the layer has an area A and is located a perpendicular distance z from and immobile surface. the magnitude of the force is given by F=ηAv/z. For a viscous fluid to move from location 2 to location 1 along 2 must exceed that at location 1, poiseuilles's law given the volumes flow rate Q that results from such a pressure difference P2−P1. The flow rate of expressed by the formula Q=πR4(P2−P1)8ηL poiseuille's law remains valid as long as the fluid flow is laminar. For a sfficiently high speed however the flow becomes turbulent flow is laminar as long as the reynolds number is less than approximately 2000. This number is given by the formula Re=2¯vρRη In which ¯v is the average speed ρ is the density η is the coefficient of viscosity of the fluid and R is the radius of the pipe. Take the density of water to be ρ=1000kg/m3
Q. What is the viscous force on a glass sphere of radius r=1mm falling through water (η=1×10−3Pa−s) when the sphere has speed of 3m/s?
View Solution
