Statement I: The de-Broglie wavelength λ of a particle of mass m moving with a velocity v is given by relation λ=hmv
Statement II : The de-Broglie wavelength of an electron which is accelerated from rest through a potential of 150 volt 1Å.Solution in Kannada
A. Statement-I is false, statement-II is true.
Statement-I is true, statement-II is false.
Statement-I is true, statement-II is true. Statement-II is correct explanation of statement-I
Statement-I is true, statement-II is true and statement - II is not correct explanation of statement-I.
The correct Answer is:C
Step by step video, text & image solution for Statement I: The de-Broglie wavelength lamda of a particle of mass m moving with a velocity v is given by relation lamda=(h)/(mv) Statement II : The de-Broglie wavelength of an electron which is accelerated from rest through a potential of 150 volt 1Å. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELECTRONS & PHOTONSBOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - MULTIPLE CHOICE QUESTIONS (LEVEL-II)
63 Videos
- ELECTRONS & PHOTONSBOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - MULTIPLE CHOICE QUESTIONS (LEVEL-III)
9 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - RECENT COMPETITIVE QUESTIONS
4 Videos
- ELECTROSTATICSBOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - RECENT COMPETITIVE QUESTIONS
39 Videos
Similar Questions
Maximum frequency of the photon produced by the union of a proton and a antiproton is (given : mp=1.67×10−27kg):
View SolutionEnergy required for the electron excitation in Li++ from the first to the third Bohr orbit is :
View SolutionThe speed of electromagnetic wave in vacuum depends upon the source of radiation
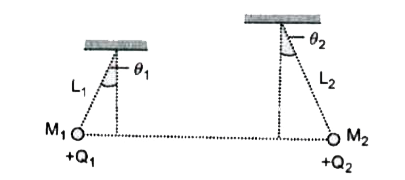
View SolutionTwo small spheres of masses M1andM2 are suspended by weightless insulating threads of lengths L1andL2 The spheres carry charges Q1andq2 respectively . The spheres are suspended such that they are in level with one another and the threads are inclined to the vertical at angles of θ1andθ2 as shown . Which one o fthe following conditions , if θ1=θ2 ?
View SolutionStatement-1: An electron of mass m when accelerated through a potential difference V , has de-broglie wavelength lambda . The de-broglie wavelength associated with a proton of mass M accelerated through the same potential difference will be lambdasqrt(M//m) . Statement-2: de-broglie wavelength lambda=h/(sqrt(2meV)) .
View SolutionThe de Broglie wavelength λ of an electron accelerated through a potential V in volts is
View Solutionसिद्ध कीजिए कि V वोल्ट विभवान्तर से त्वरित किए गए कण कि दी-ब्रोग्ली तरंगदैर्ध्य λ=√150VÅ होगी ।
View SolutionIf the de broglie wavelength of an electron is 1Å, then the velocity of the electron will be
View SolutionThe de Broglie wavelength associated with an electron accelerated through a potential difference V is lamda . What will be its wavelength when the accelerating potential is increased to 4 V?
View SolutionShow that the de Broglie wavelength of charged particles accelerated through a potential of V volt is given by λ=h/√2mqV. Hence show that the de-Broglie wavelength of electron is 12.27√VÅ .
View SolutionStatement I: If a stationary electron is accelerated with a potential difference of 1V, its de Broglie wavelength becomes 12.27 Å approximately. Statement II: The relation between the de Broglie wavelength lamda and the accelerating potential V of an electron is given by lamda = (12.27)/(V) Å
View SolutionAn electron accelerated through a potential of 10000 V from rest has a de Broglie wavelength λ. What should be the accelerating potential so that the wavelength is doubled?
View Solutionविभवान्तर 50 वोल्ट पर त्वरित इलेक्ट्रॉन से सम्बद्ध डी-ब्रॉग्ली तरंगदैर्घ्य होता है-
View Solutionदिखाइए कि V विभवान्तर में त्वरित करने पर एक इलेक्ट्रॉन के लिए डी-ब्रॉगली तरंगदैर्ध का मान λ=12.27√V Å द्वारा दिया जाता है।
View SolutionAn electron of mass m, when accelerated through a potential difference V, has de-Broglie wavelength λ, the de-Broglie wavelength associated with a protom of mass M and accelerated through the same potential difference V will be
View Solution
