The altitude of a hang glider is increasing at a rate of 6.80 m/s. At the same time, the shadow of the glider moves along the ground at a speed of 15.5 m/s when the Sun is directly overhead. Find the magnitude of the glider's velocity.
4.72 m/s
14.1 m/s
9.44 m/s
16.9 m/s
The correct Answer is:D
To find the magnitude of the glider's velocity, we can use the Pythagorean theorem since the glider's altitude (vertical component) and the speed of its shadow (horizontal component) are perpendicular to each other.
1. Identify the components of velocity:
- The vertical component of the glider's velocity (altitude increase) is given as vy=6.80m/s.
- The horizontal component of the glider's velocity (shadow movement) is given as vx=15.5m/s.
2. Use the Pythagorean theorem:
The magnitude of the resultant velocity v can be calculated using the formula:
v=√v2x+v2y
3. Substitute the values:
v=√(15.5)2+(6.8)2
4. Calculate the squares:
- (15.5)2=240.25
- (6.8)2=46.24
5. Add the squares:
v=√240.25+46.24=√286.49
6. Calculate the square root:
v≈16.92m/s
Final Answer:
The magnitude of the glider's velocity is approximately 16.92m/s.
---
- The vertical component of the glider's velocity (altitude increase) is given as vy=6.80m/s.
- The horizontal component of the glider's velocity (shadow movement) is given as vx=15.5m/s.
The magnitude of the resultant velocity v can be calculated using the formula:
v=√v2x+v2y
v=√(15.5)2+(6.8)2
- (15.5)2=240.25
- (6.8)2=46.24
v=√240.25+46.24=√286.49
v≈16.92m/s
The magnitude of the glider's velocity is approximately 16.92m/s.
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - PRACTICE QUESTIONS (MORE THAN ONE CORRECT CHOICE TYPE)3videos
MOTION IN TWO AND THREE DIMENSIONS
- BOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - PRACTICE QUESTIONS (LINKED COMPREHENSION)14videos
MOTION IN TWO AND THREE DIMENSIONS
- BOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - PROBLEMS50videos
MOTION IN TWO AND THREE DIMENSIONS
- BOOK - RESNICK AND HALLIDAYCHAPTER - MOTION ALONG A STRAIGHT LINEEXERCISE - PRACTICE QUESTIONS ( Integer Type )3videos
MOTION ALONG A STRAIGHT LINE
- BOOK - RESNICK AND HALLIDAYCHAPTER - OSCILLATIONSEXERCISE - Practice Questions57videos
OSCILLATIONS
Similar Questions
A plane flies at 2.00 times the speed of sound. Its sonic boom reaches a man on the ground 35.4 s after the plane passes directly overhead. What is the altitude of the plane? Assume the speed of sound to be 330 m/s.
View SolutionThe shadow of a tower standing on a level ground is found to be 40 m longer when the Suns altitude is 30othan when it is 60o. Find the height of the tower.
View Solution
Knowledge Check
- Question 1 - Select One
Ram moves in east direction at a speed of 6 m/s and Shyam moves 30∘ east of north at a speed of 6 m/s. The magnitude of their relative velocity is
A3 m/sB6 m/sC6√3m/sD6√2m/s - Question 2 - Select One
Rain is falling with a sped of 12√2m/s at an angle 45∘ with the vertical line. A man in a glider going at a speed of u at an angle of 37∘ with respect to the ground. Find the speed of the glider so that rain appears to him falling vertically. Consider the motion of the glider and rain drops in the same vertical plane.
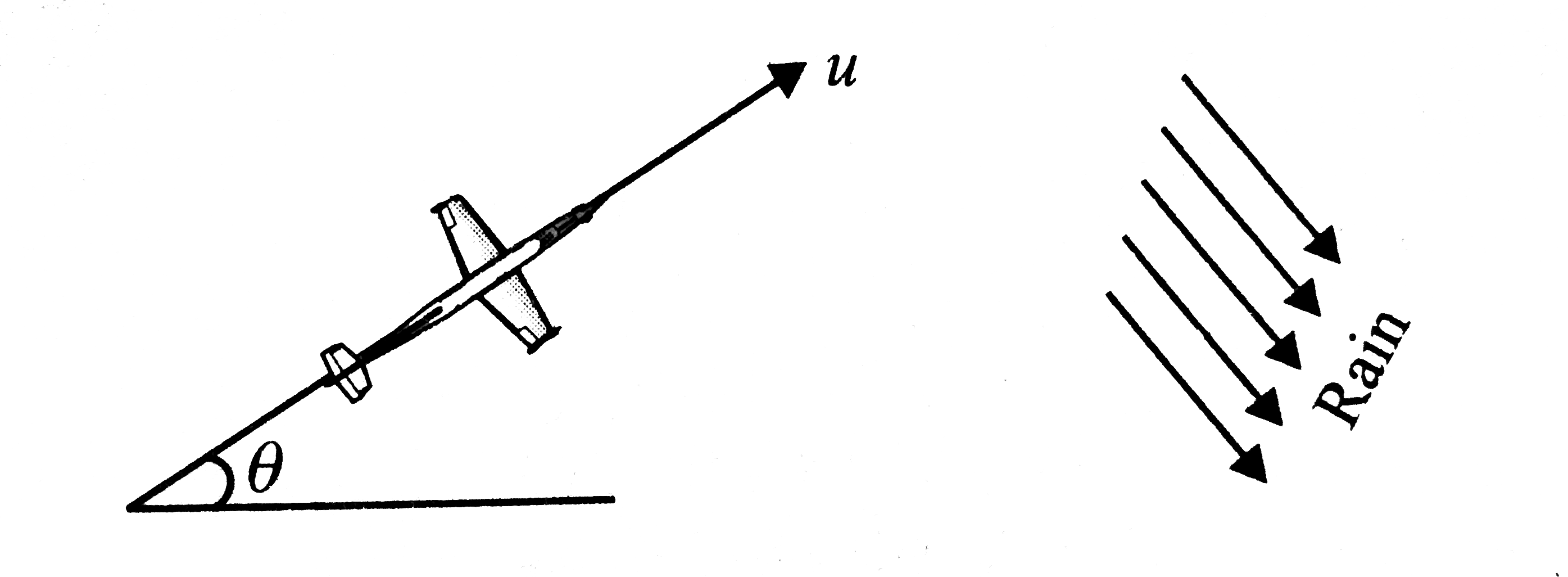 A15ms−1B30ms−1C10ms−1D25ms−1
A15ms−1B30ms−1C10ms−1D25ms−1
The shadow of a vertical tower on level ground increases by 10 m when the altitude of the sun changes from 45∘ to 30∘. Find the height of the tower.
View SolutionA car is moving northwards at a constant speed of 5 m/s . It makes a right turn and continues to move with a constant speed of 5 m/s . Find the magnitude of change in velocity.
View Solution
Vijay is trying to find the average height of a tower near his house. He is using the properties of similar triangles.The height of Vijay’s house if 20m when Vijay’s house casts a shadow 10m long on the ground. At the same time, the tower casts a shadow 50m long on the ground and the house of Ajay casts 20m shadow on the ground.
What will be the length of the shadow of the tower when Vijay’s house casts a shadow of 12m?View SolutionA vertical pole of length 8 m casts a shadow of 15 m long on the ground. At the same time, a tower casts a shadow 45 m long. Find the height of the tower.
View SolutionThe shadow of a tower standing on a level ground is found to be 40m longer when Suns altitude is 30o than when it was 60o . Find the height of the tower.
View SolutionTwo objects of masses 100g and 200g are moving along the same line in the same line in the same direction with velocities of 2m/s and 1m/s, respectively. They collide and after the collison, the first object moves at a velocity of 1.67m/s in the same direction. Determine the velocity of the second object.
View Solution

