The angle of incidance for an equilateral prism is 60∘. What should be the refractive index of prism so that the ray is parallel to the base inside the prism ?
√2
√3
43
98
The correct Answer is:B
To solve the problem, we need to find the refractive index (μ) of an equilateral prism when the angle of incidence is 60 degrees, and the ray inside the prism is parallel to the base.
1. Understand the Geometry of the Prism:
- An equilateral prism has angles of 60 degrees at each vertex.
- The angle of incidence (i) is given as 60 degrees.
2. Identify the Angles:
- Since the prism is equilateral, the angle at the base (angle of the prism) is also 60 degrees.
- When the ray is refracted inside the prism and is parallel to the base, the angle of refraction (r) at the second face of the prism will also be 30 degrees (because the total angle at the vertex of the prism is 60 degrees).
3. Apply Snell's Law:
- Snell's Law states that n1sin(i)=n2sin(r).
- Here, n1 is the refractive index of air (approximately 1), i is the angle of incidence (60 degrees), n2 is the refractive index of the prism (μ), and r is the angle of refraction (30 degrees).
4. Substitute the Values into Snell's Law:
- We can write the equation as:
1⋅sin(60∘)=μ⋅sin(30∘)
5. Calculate the Sine Values:
- We know that:
- sin(60∘)=√32
- sin(30∘)=12
6. Substitute the Sine Values into the Equation:
- The equation now becomes:
√32=μ⋅12
7. Solve for the Refractive Index (μ):
- Multiply both sides by 2 to eliminate the fraction:
√3=μ
- Thus, the refractive index of the prism is:
μ=√3
Final Answer:
The refractive index of the prism should be √3.
---
- An equilateral prism has angles of 60 degrees at each vertex.
- The angle of incidence (i) is given as 60 degrees.
- Since the prism is equilateral, the angle at the base (angle of the prism) is also 60 degrees.
- When the ray is refracted inside the prism and is parallel to the base, the angle of refraction (r) at the second face of the prism will also be 30 degrees (because the total angle at the vertex of the prism is 60 degrees).
- Snell's Law states that n1sin(i)=n2sin(r).
- Here, n1 is the refractive index of air (approximately 1), i is the angle of incidence (60 degrees), n2 is the refractive index of the prism (μ), and r is the angle of refraction (30 degrees).
- We can write the equation as:
1⋅sin(60∘)=μ⋅sin(30∘)
- We know that:
- sin(60∘)=√32
- sin(30∘)=12
- The equation now becomes:
√32=μ⋅12
- Multiply both sides by 2 to eliminate the fraction:
√3=μ
- Thus, the refractive index of the prism is:
μ=√3
The refractive index of the prism should be √3.
Topper's Solved these Questions
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - A. Only one option is correct (JEE Advance)
73 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - B. More than one option is correct
20 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
Similar Questions
What is the angle of incidence for an equilateral prism of refractive index √3 so that the ray si parallel to the base inside the prism?
View SolutionThe angle of minimum deviation produced by an equilateral prism is 46∘ The refractive index of material of the prism.
View SolutionIf the angle of minimum deviation is of 60∘ for an equilateral prism , then the refractive index of the material of the prism is
View SolutionA ray of light incident at an angle of incidence 'I' passes through an equilateral glass prism such that the refracted ray inside the prism is parallel to its base and emerges from the prism at an angle of emergence 'e'.
How is the angle of emergence 'e' related to the angle of incidence 'i' ?
View SolutionA ray of light incident at an angle of incidence 'I' passes through an equilateral glass prism such that the refracted ray inside the prism is parallel to its base and emerges from the prism at an angle of emergence 'e'.
What can you say about the value of the angle of deviation in such a situation ?
View SolutionA ray of light undergoes a deviation of 30∘ when incident on an equilateral prism of refractive index √2.
What is the angle subtended by the ray inside the prism with the base of the prism?
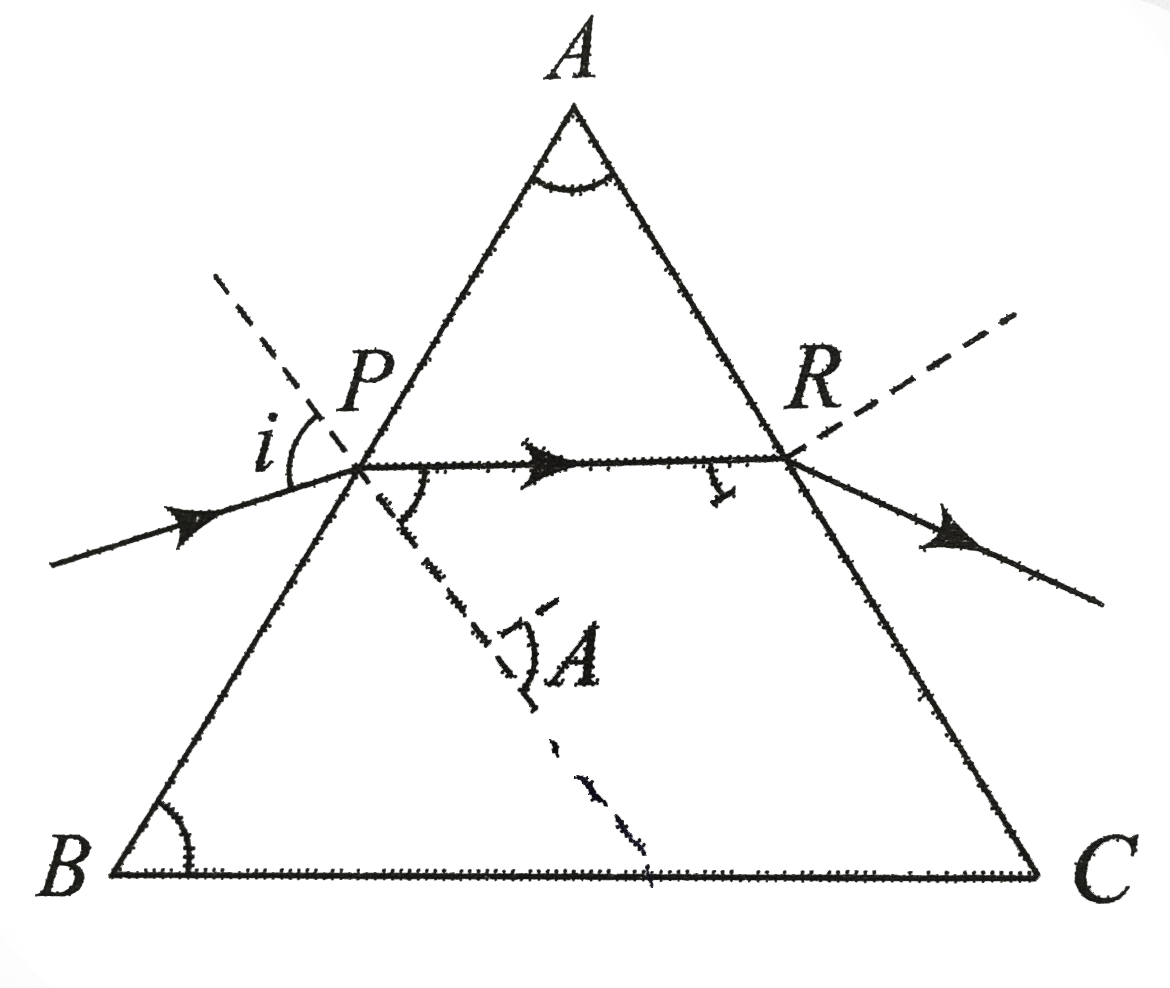
View SolutionA ray of light undergoes deviation of 30∘ when incident on an equilateral prism of refractive index √2. what is the angle subtended by the ray inside the prism with the base of the prism?
View SolutionThe angle of minimum deviation measured with a prism is 30∘ and the angle of prism is 60∘. The refractive index of prism material is
View SolutionA ray of light undergoes deviation of 30∘ when incident on an equilateral prism of refractive index √2. The angle made by the ray inside the prism with the base of the prism is
View SolutionThe refractive index of a prism is 2. this prism can have a maximum refracting angle of
View Solution
