The apparent thickness of a thick plano-convex lens is measured once with the plane face upward and then with the convex face upward. The value will be
more in the firest case
same in the two cases
more in the second case
any of the above depending on the value of its actual thickness
The correct Answer is:c.
To solve the problem of finding the apparent thickness of a thick plano-convex lens when measured with the plane face upward and then with the convex face upward, we will follow these steps:
Step 1: Define the Problem
We have a thick plano-convex lens, and we need to determine the apparent thickness when measured in two orientations:
1. Plane face upward
2. Convex face upward
Step 2: Apparent Thickness with Plane Face Upward
When the plane face of the lens is facing upward, we can use the formula for apparent depth:
Apparent Thickness=Dμ
where D is the actual thickness of the lens and μ is the refractive index of the lens material.
Step 3: Apparent Thickness with Convex Face Upward
When the convex face is facing upward, we need to apply the formula for refraction at a curved surface. The formula for refraction at a curved surface is given by:
μ2V−μ1u=μ2−μ1R
In this case:
- μ2=1 (the refractive index of air)
- μ1=μ (the refractive index of the lens)
- u=−D (the object distance, taken as negative since it is on the same side as the incoming light)
- R=−D (the radius of curvature, taken as negative for the convex surface)
Substituting these values into the formula, we have:
1V−μ−D=1−μ−D
Step 4: Solve for V
Rearranging the equation gives:
1V+μD=μ−1D
1V=μ−1−μD=−1D
Thus, we find:
V=−D
Step 5: Conclusion
From the calculations:
- Apparent thickness with plane face upward: Dμ
- Apparent thickness with convex face upward: −D
Since −D indicates that the image appears at a distance D below the lens, we can conclude that the apparent thickness when the convex face is upward appears greater than when the plane face is upward.
Final Answer
The apparent thickness of the lens is greater when measured with the convex face upward.
---
We have a thick plano-convex lens, and we need to determine the apparent thickness when measured in two orientations:
1. Plane face upward
2. Convex face upward
When the plane face of the lens is facing upward, we can use the formula for apparent depth:
Apparent Thickness=Dμ
where D is the actual thickness of the lens and μ is the refractive index of the lens material.
When the convex face is facing upward, we need to apply the formula for refraction at a curved surface. The formula for refraction at a curved surface is given by:
μ2V−μ1u=μ2−μ1R
In this case:
- μ2=1 (the refractive index of air)
- μ1=μ (the refractive index of the lens)
- u=−D (the object distance, taken as negative since it is on the same side as the incoming light)
- R=−D (the radius of curvature, taken as negative for the convex surface)
1V−μ−D=1−μ−D
Rearranging the equation gives:
1V+μD=μ−1D
1V=μ−1−μD=−1D
Thus, we find:
V=−D
From the calculations:
- Apparent thickness with plane face upward: Dμ
- Apparent thickness with convex face upward: −D
The apparent thickness of the lens is greater when measured with the convex face upward.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Assertion-Reasoninig
2 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Linked Comprehension
51 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Subjective
26 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
A plano-convex lens is made of flint glass, its focal length is
View SolutionA plano-convex lens is made of glass of refractive index 1.5. The radius of curvature of its convex surface is R. Its focal length is
View SolutionThe plane surface of a plano-convex lens of focal length f is silvered. It will behave as
View SolutionA plano convex lens made of glass (n=1.5) has a focal length 10.0 cm.
(i) calculate the radius of curvature surface.
(ii) If an identical plano-convex lens is placed in contact with the first (plane surface facing each other), What will be the power of the combination ( in diopter) ?
(b) An astronomical telescope consists of two convex lenses having focal length 80 cm and 4 cm. When it is normal adjustment, what is its :
(i) length
(ii) Magnifying power ?
View SolutionFocal length of the plano-convex lens is 15cm. A small object is placed at A as shown in figure. The plane surface is silvered. The image will form at
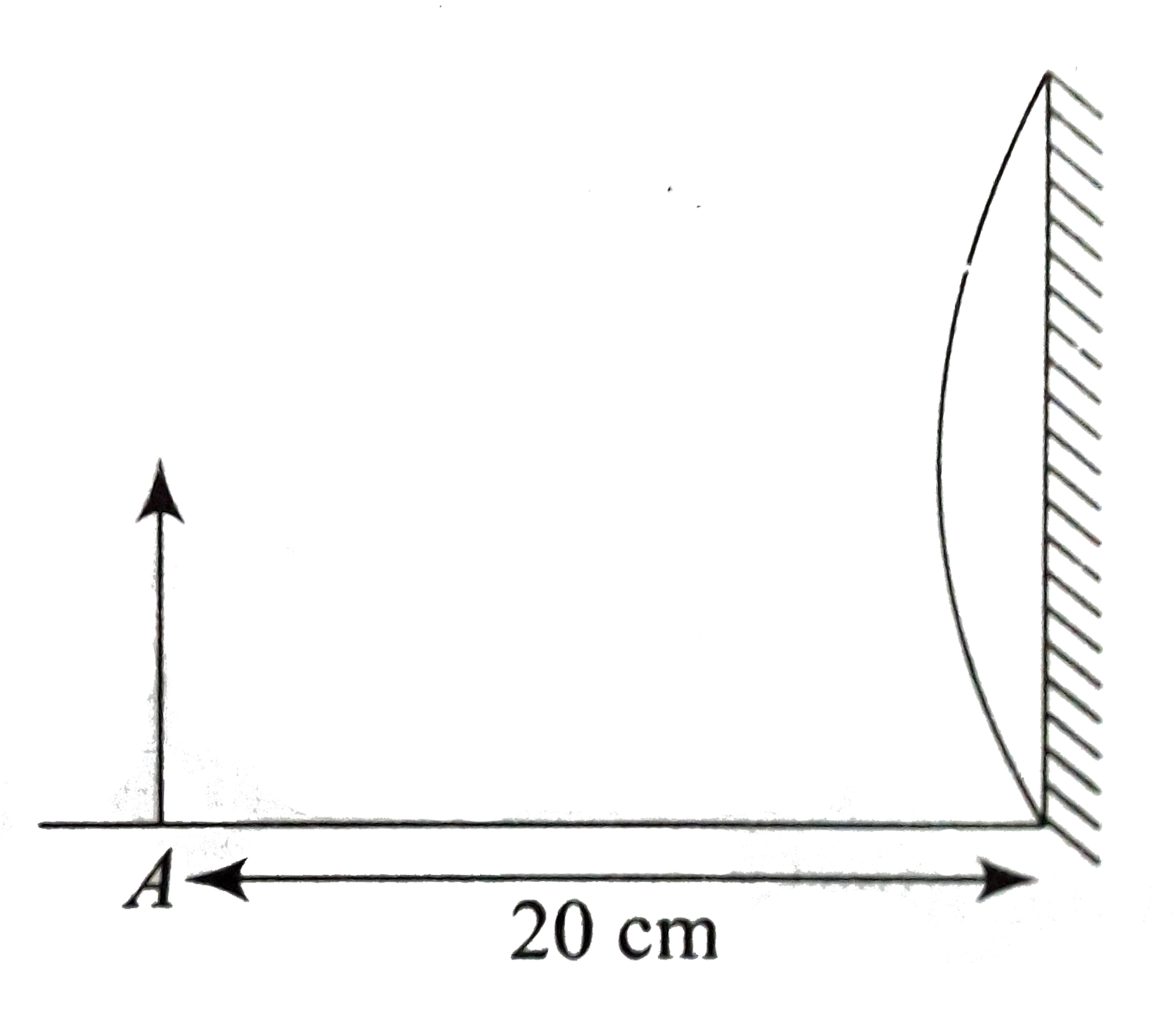
View SolutionThe radius of curvature of curved surface of a thin plano-convex lens is 10cm and the refractive index is 1.5. If the plano surface is silvered, then the focal length will be.
View SolutionA plano-convex lens is made of refractive index of 1.6. The focal length of the lens is
View SolutionA plano-convex lens has thickness 4cm. When places on a horizontal table with the curved surface in contact with it, the apparent depth of the bottom-most point of the lens if found to be 3cm. If the lens is inverted such that the plane face of the lens is in contact with the table, the apparent depth of the center of the plane face of the lens is found to be 25/8 cm. Find the focal length of the lens.
View SolutionTwo plano-convex lenses of focal lengths 20cm and 30cm are placed together to form a double convex lens. The final focal length will be
View SolutionThe place faces of two identical plano-convex lenses, each with focal length f are pressed against each other using an optical glue to from a usual convex lens .The distance from the optical centre at which an object must be placed to obtain the image same as the size of the object is
View Solution
