Question

The atomic mass ${.}_2^{4}He$ is $4.0026u$ and the atomic mass of ${.}_1^{1}H$ is $1.0078u$. Using atomic mass units instead of kilograms, obtain the binding energy of ${.}_2^{4}He$ nucleus.

Explanation

To determine the binding energy, we calcualte the mass defect in atomic mass units and then use the fact that one atomic mass unit is equivalent to `931.5 MeV` of energy. The mass of `4.0026 u` for `._2^4 He` inclides the mass defect, we must subtract `4.0026 u` from the sum of the individual masses of the nucleons, including the mass to the electrons . As Fig. illustrates, the electron mass will be included if the masses of two hydrogen atoms are used in the calculation instead of the masses of two protons. The mass of a hydrogen atom is given as `1.0078 u`, and the mass of a neutron is given in Table `5.1` as `1.0087 u`.
`._2^4 H`

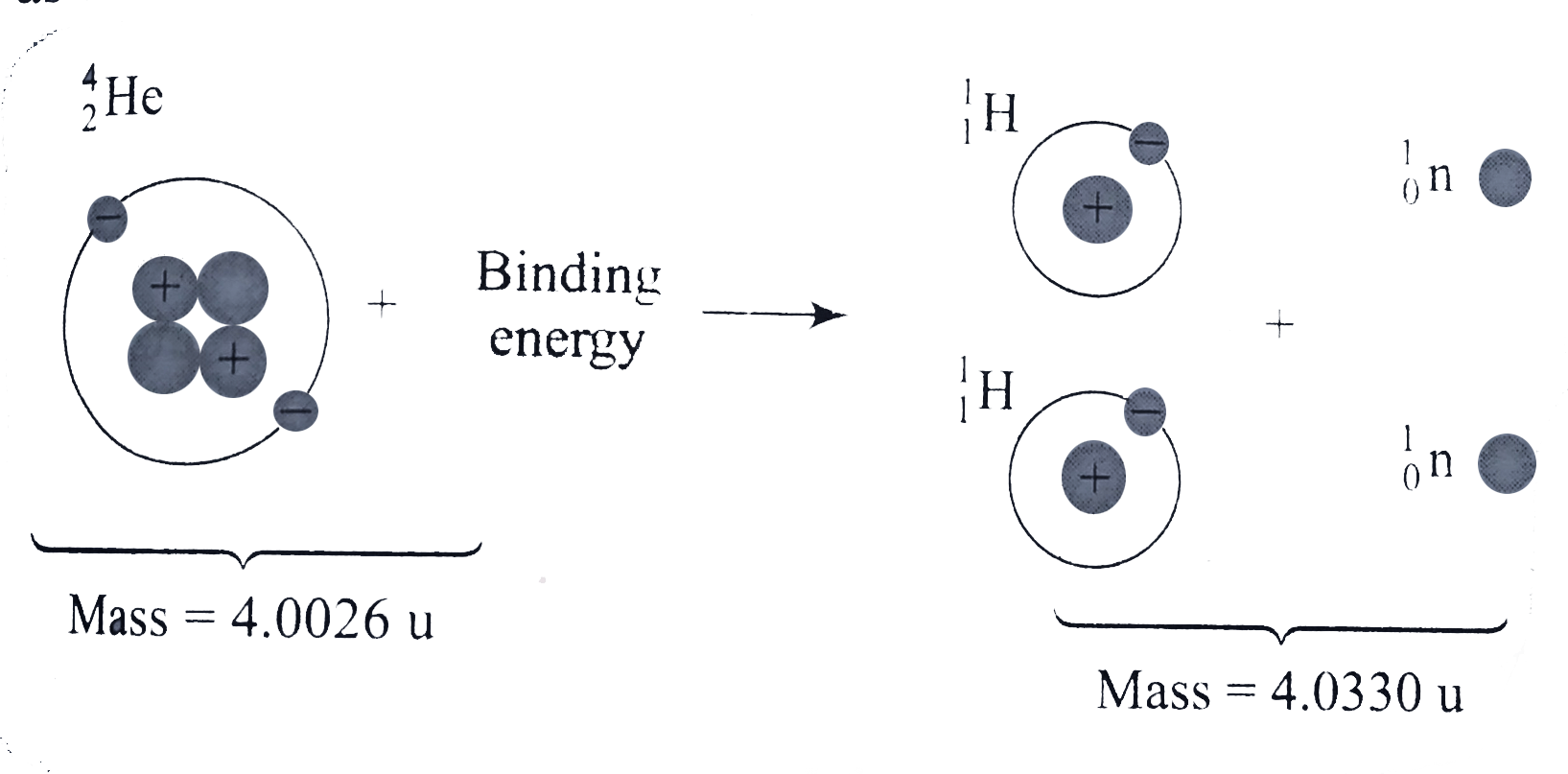
The sum of the individual masses is
`ubrace(2(1.0078 u))_(("Two hydrogen"),("atoms"))+ (2(1.0087 u))/("Two neutrons") =4.0330 u`
The mass defect is `Delta m =4.0330 u -4.0026 u =0.0304 u`
Since `1 u` is equivalent to `931.5 MeV` , the binding energy is` 28.3 MeV`.