The centre of gravity of a uniform ball is:
at is geometrical centre
at its bottom
at its topmost point
at any point on its surface
To determine the center of gravity of a uniform ball, we can follow these steps:
1. Understand the Definition: The center of gravity (CG) is the point where the weight of a body is considered to act. For symmetrical objects, this point is often at the geometric center.
2. Identify the Type of Object: In this case, we have a uniform ball. A uniform ball means that the mass is distributed evenly throughout its volume.
3. Consider the Symmetry: A uniform ball is a three-dimensional object that is symmetrical in all directions. This symmetry is crucial in determining the center of gravity.
4. Locate the Geometric Center: For a sphere (which is the shape of a ball), the geometric center is the point that is equidistant from all points on the surface of the sphere. This point is at the center of the ball.
5. Conclusion: Since the ball is uniform and symmetrical, the center of gravity will be located at the geometric center of the ball.
Final Answer:
The center of gravity of a uniform ball is at its center, which is the geometric center of the ball.
---
The center of gravity of a uniform ball is at its center, which is the geometric center of the ball.
Topper's Solved these Questions
- FORCE BOOK - ICSECHAPTER - FORCE EXERCISE - EXERCISE 1(C )
14 Videos
- FORCE BOOK - ICSECHAPTER - FORCE EXERCISE - EXERCISE 1( C)
1 Videos
- FORCE BOOK - ICSECHAPTER - FORCE EXERCISE - EXERCISE 1B
11 Videos
- ELECTRO-MAGNETISM BOOK - ICSECHAPTER - ELECTRO-MAGNETISM EXERCISE - EXERCISE-10 (C) NUMERICALS
1 Videos
- HOUSEHOLD CIRCUITS BOOK - ICSECHAPTER - HOUSEHOLD CIRCUITS EXERCISE - EXERCISE -9(B) (MULTIPLE CHOICE TYPE)
2 Videos
Similar Questions
Where is the centre of gravity of a uniform ring situated ?
View SolutionTwo identical uniform rods AB and CD, each of length L are jointed to form a T-shaped frame as shown in figure. Locate the centre of mass of the frame. The centre of mass of a uniform rod is at the middle point of the rod.
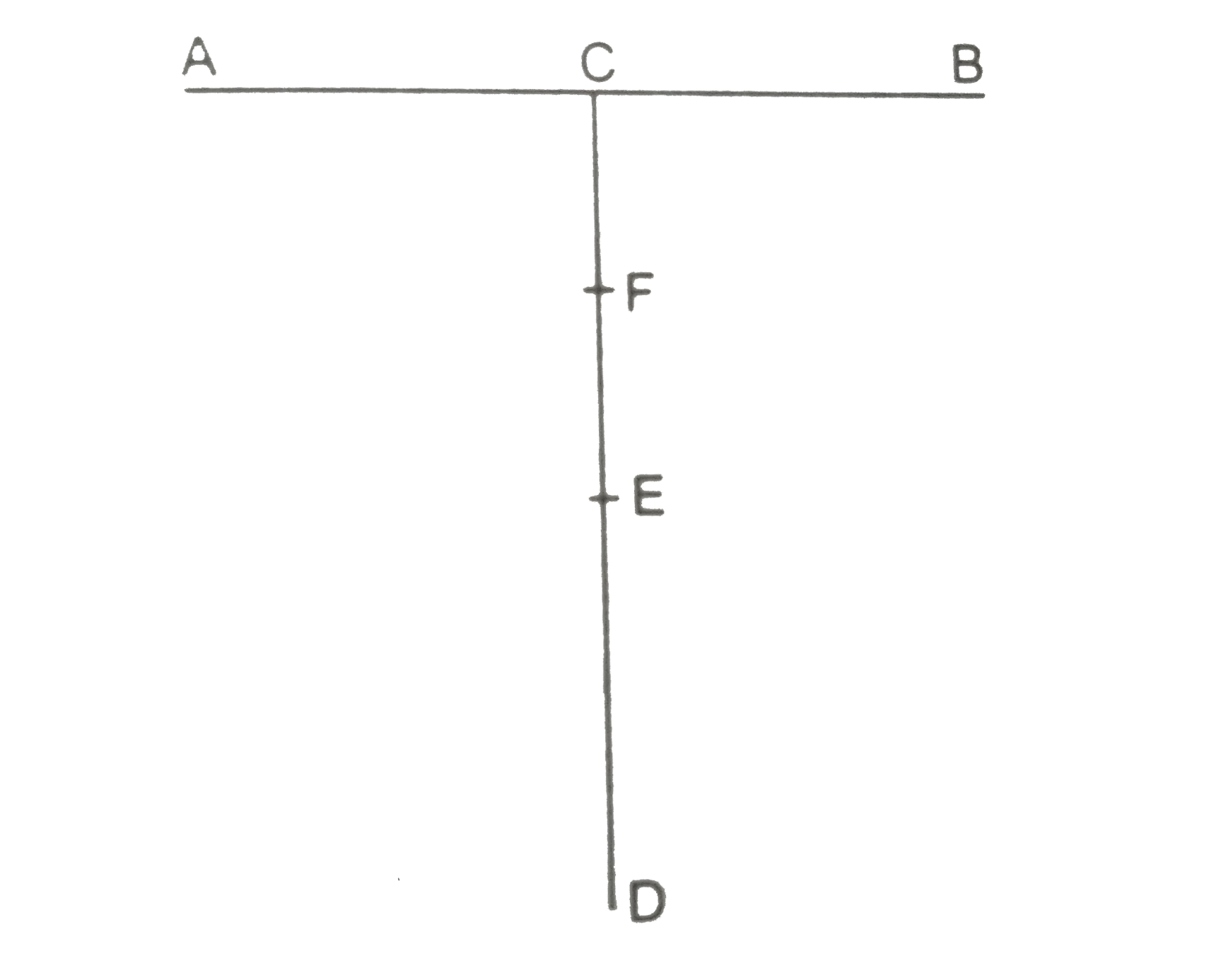
View Solution
Knowledge Check
Assertion: The centre of gravity of a body coincides with its centre of mass only if the gravitational field does not vary form one part of the body to the other.
Reason: Centre of gravity is independent of the gravitational field.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertionCIf assertion is true but reason is false.DIf both assertion and reason are false.
Find the centre of mass of a uniform solid cone.
View SolutionDefine the centre of gravity of a homogenous rigid body.
View SolutionWhat is the location of the centre of mass of a uniform triangular lamina ?
View SolutionDetermine the centre of mass of a uniform hemisphere of radius R.
View SolutionDefine the term centre of gravity of a body?
View SolutionName the factor affecting the Centre of gravity of a body.
View SolutionA boy holds a rubber ball in his hand. The reaction force of gravity on the ball is the force exerted by the
View Solution
