The centre of gravity of a hollow cone of height h is at distance x from its vertex where the value of x is:
h/3
h/4
2h/3
3h/4
To find the distance x from the vertex of a hollow cone to its center of gravity, we can follow these steps:
Step 1: Understand the Geometry of the Hollow Cone
A hollow cone has a circular base and a vertex. The height h is the perpendicular distance from the base to the vertex.
Step 2: Identify the Center of Gravity Location
For a hollow cone, the center of gravity is located along the axis of the cone. It is positioned at a certain distance from the base and from the vertex.
Step 3: Use Known Values
It is a known fact that the center of gravity of a hollow cone is located at a distance of h3 from the base. Therefore, the distance from the vertex to the center of gravity can be calculated as follows:
Step 4: Calculate the Distance from the Vertex
Since the total height of the cone is h, the distance from the vertex to the center of gravity x can be determined by subtracting the distance from the base to the center of gravity from the total height:
x=h−h3
Step 5: Simplify the Expression
Now, we simplify the expression:
x=h−h3=3h3−h3=2h3
Conclusion
Thus, the distance x from the vertex to the center of gravity of the hollow cone is:
x=2h3
A hollow cone has a circular base and a vertex. The height h is the perpendicular distance from the base to the vertex.
For a hollow cone, the center of gravity is located along the axis of the cone. It is positioned at a certain distance from the base and from the vertex.
It is a known fact that the center of gravity of a hollow cone is located at a distance of h3 from the base. Therefore, the distance from the vertex to the center of gravity can be calculated as follows:
Since the total height of the cone is h, the distance from the vertex to the center of gravity x can be determined by subtracting the distance from the base to the center of gravity from the total height:
Now, we simplify the expression:
Thus, the distance x from the vertex to the center of gravity of the hollow cone is:
Topper's Solved these Questions
- BOOK - ICSECHAPTER - FORCE EXERCISE - EXERCISE 1(C )14videos
FORCE
- BOOK - ICSECHAPTER - FORCE EXERCISE - EXERCISE 1( C)1videos
FORCE
- BOOK - ICSECHAPTER - FORCE EXERCISE - EXERCISE 1B11videos
FORCE
- BOOK - ICSECHAPTER - ELECTRO-MAGNETISM EXERCISE - EXERCISE-10 (C) NUMERICALS1videos
ELECTRO-MAGNETISM
- BOOK - ICSECHAPTER - HOUSEHOLD CIRCUITS EXERCISE - EXERCISE -9(B) (MULTIPLE CHOICE TYPE)2videos
HOUSEHOLD CIRCUITS
Similar Questions
The largest magnitude the electric field on the axis of a uniformly charged ring of radius 3m is at a distance h from its centre. What is the value of h? (Take 1√2=0.7)
View SolutionFor a uniformly charged ring of radius R, the electric field on its exis has the largest magnitude at a distance h from its centre. Then value of h is :
View Solutiona body is thrown horizontally with a velocity √2gh from the top of a tower of height h. It strikes the level gound through the foot of the tower at a distance x from the tower. The value of x is :-
View SolutionThe value of g at a certain height h above the free surface of the earth is x/4 where x is the value of g at the surface of the earth. The height h is
View SolutionA ball is thrown from a point in level with velocity u and at a horizontal distance r from the top os a tower of height h.
At what horizontal distance x from the foot of the tower does the ball hit the ground ? View Solution
View SolutionA stone is thrown horizontally with a velocity 2√gh from the top of a tower of height h. It strikes the ground level through the foot of tower at a distance x from it. What is the value of x?
View SolutionThe magnetic field at a distance X from the centre, on the axis of a circular coil of radius R is 127 th of that at the center. The value of X is
View SolutionA glass marble projected horizontally from the top of a table falls at a distance x from the edge of the table. If h is the height of the table, then the velocity of projection is
View SolutionA tank is filled up to a height 2H with a liquid and is placed on a platform of height H from the ground, the distance x from the ground where a small hole is punched to get the maximum range R is
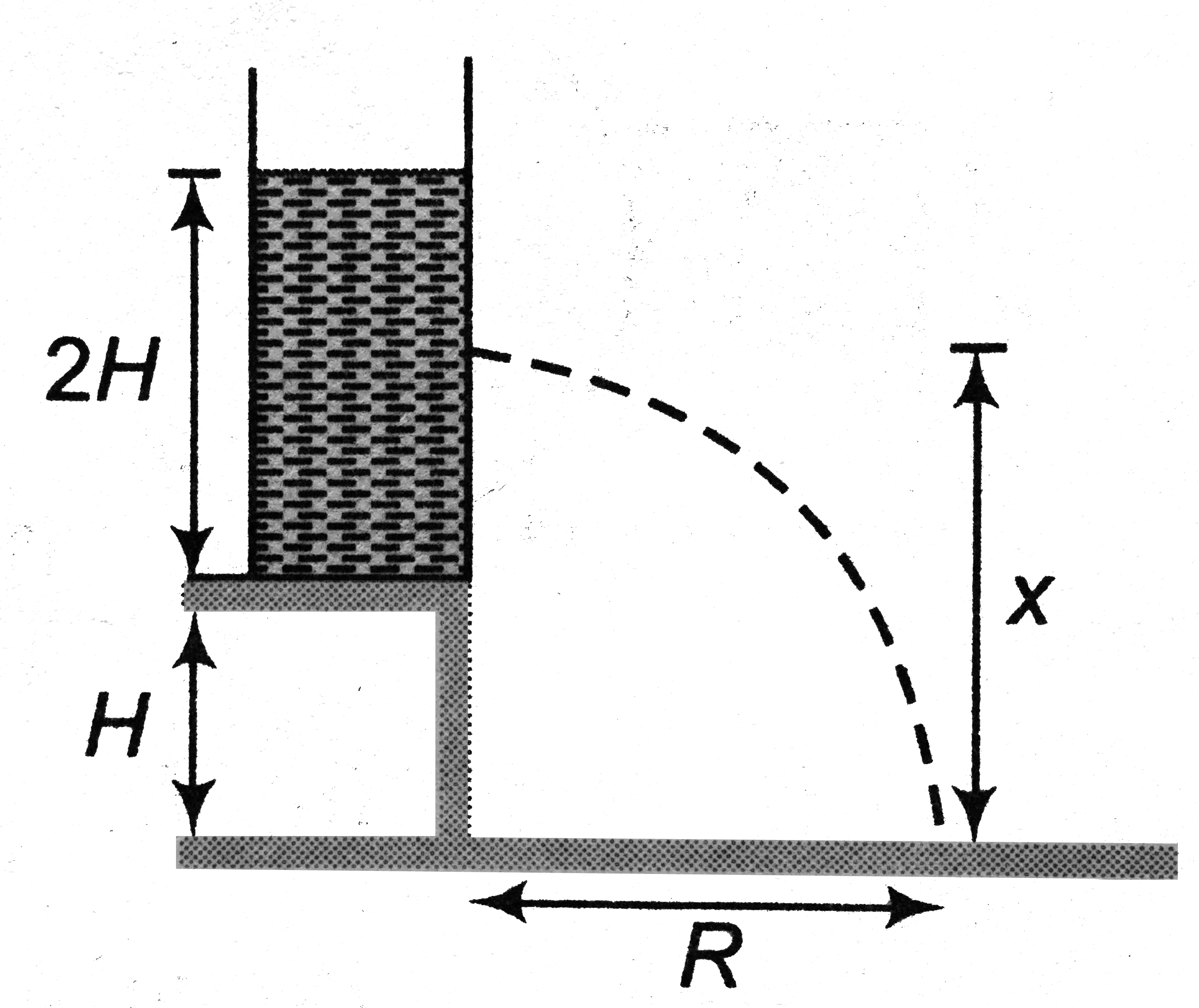 .View Solution
.View SolutionAcceleration due to gravity as same at height h from surface and at depth d from surface, then find value of d
View Solution
